Phân tích đa thức sau thành nhân tử: x2 + 2x – 3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
XO
4 tháng 8 2021
Ta có : x2 + 2x - 3
= x2 - x + 3x - 3
= x(x - 1) + 3(x - 1)
= (x + 3)(x - 1)
TL
2
ND
Nguyễn Đức Trí
VIP
7 tháng 8 2023
a) \(x^4+8x+63\)
\(=x^4+4x^3+9x^2-4x^3-16x^2-36x+7x^2+28x+63\)
\(=x^2\left(x^2+4x+9\right)-4x\left(x^2+4x+9\right)+7\left(x^2+4x+9\right)\)
\(=\left(x^2+4x+9\right)\left(x^2-4x+7\right)\)
ND
Nguyễn Đức Trí
VIP
7 tháng 8 2023
c) \(\left(x^2+2x+7\right)+\left(x^2-2x+4\right)\left(x^2+2x+3\right)\left(1\right)\)
Ta có : \(x^3-8=\left(x-2\right)\left(x^2+2x+4\right)\)
\(\Rightarrow x^2+2x+4=\dfrac{x^3-8}{x-2}\)
\(\left(1\right)\Rightarrow\left[\left(\dfrac{x^3-8}{x-2}+3\right)\right]+\left(x^2-2x+4\right)\left[\left(\dfrac{x^3-8}{x-2}-1\right)\right]\)
\(=\left[\left(\dfrac{x^3-3x-14}{x-2}\right)\right]+\left(x^2-2x+4\right)\left[\left(\dfrac{x^3-2x-5}{x-2}\right)\right]\)
\(=\dfrac{1}{x-2}\left[x^3-3x-14+\left(x^2-2x+4\right)\left(x^3-2x-5\right)\right]\)
PT
1
CM
15 tháng 7 2018
Cách 1: x 2 + 2xy - 15 y 2 = ( x 2 + 2xy + y 2 ) - 16 y 2
= x + y 2 - 4 y 2
= (x + y + 4y)(x + y – 4y)
= (x + 5y)(x – 3y).
Cách 2: x 2 + 2xy - 15 y 2 = x 2 + 5xy – 3xy - 15 y 2
= x(x + 5y) – 3y(x + 5y)
= (x – 3y)(x + 5y).
PT
1
NP
2
2 tháng 8 2021
\(x^2+2x+1-y^2\)
\(=\left(x+1\right)^2-y^2\)
\(=\left(x+1-y\right)\left(x+1+y\right)\)
PT
1
PT
1
CM
15 tháng 4 2019
2x – 2y – x2 + 2xy – y2
(Có x2 ; 2xy ; y2 ta liên tưởng đến HĐT (1) hoặc (2))
= (2x – 2y) – (x2 – 2xy + y2)
= 2(x – y) – (x – y)2
(Có x – y là nhân tử chung)
= (x – y)[2 – (x – y)]
= (x – y)(2 – x + y)
NM
3
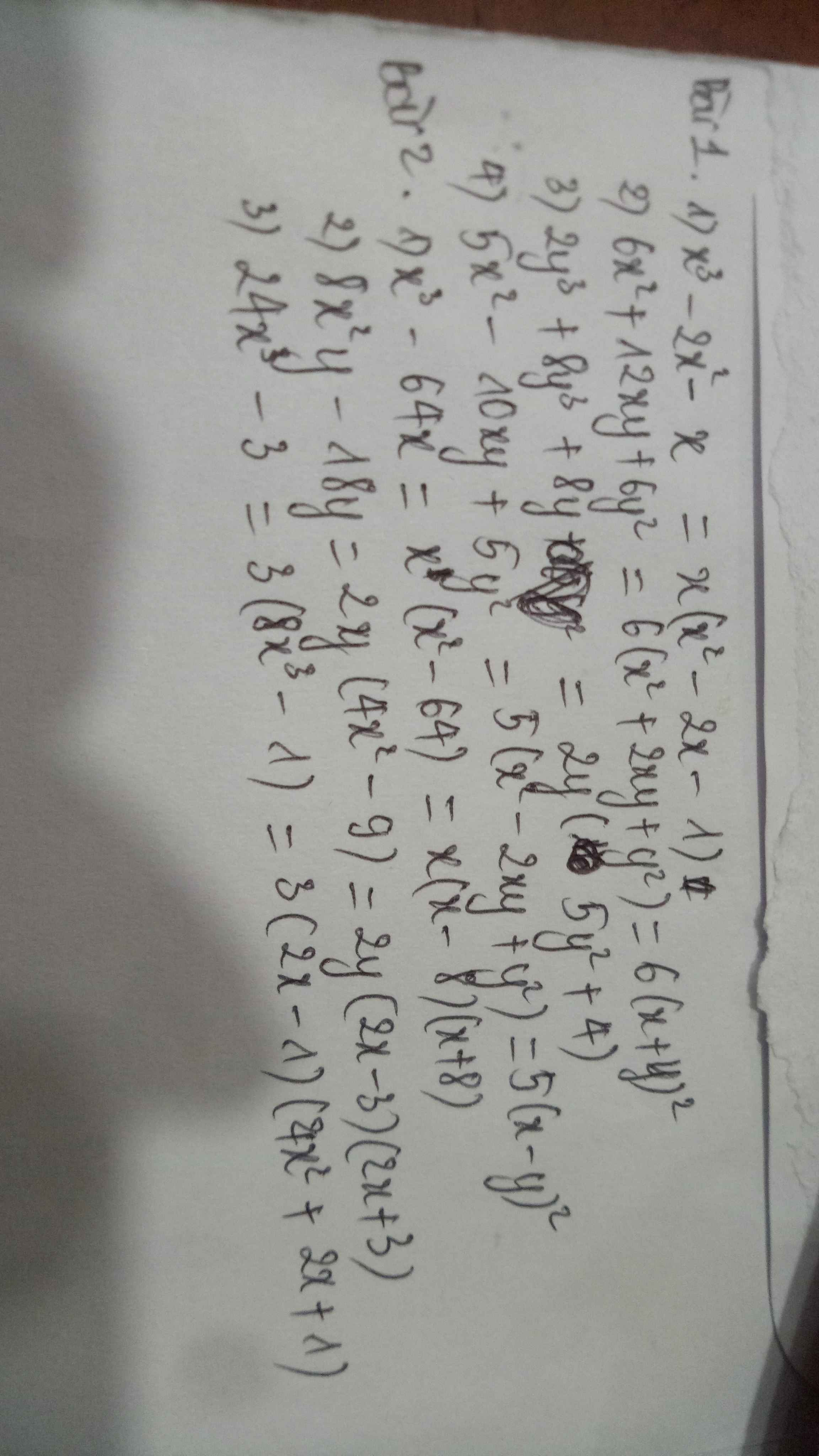
x2 + 2x – 3
= x2 + 2x + 1 – 4
= (x + 1)2 – 22
= (x + 1 + 2)(x + 1 – 2)
= (x + 3)(x – 1)