rút gọn biểu thức: A = sin(90 -x)sin(180 -x)
B= cos(90 -x)có(180 -x)
giúp mk vs, mk cần gấp lắm
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


1234567890-01234567890-=qưertyuiop[]\';;lkjhfgdsazxcvbnm,./\'l;[]7894561230.+-

\(B=\dfrac{1-4\sin^2x\cdot\cos^2x}{\sin^2x+2\sin x\cdot\cos x+\cos^2}+2\sin x\cdot\cos x\\ B=\dfrac{1-4\sin^2x\cdot\cos^2x}{2\sin x\cdot\cos x}+2\sin x\cdot\cos x\\ B=\dfrac{1-4\sin^2x\cdot\cos^2x+4\sin^2x\cdot\cos^2x}{2\sin x\cdot\cos x}=\dfrac{1}{2\sin x\cdot\cos x}\)

a:\(a\cdot sin0+b\cdot cos0+c\cdot sin90\)
\(=a\cdot0+b\cdot1+c\cdot1\)
=b+c
b: \(a\cdot cos90+b\cdot sin90+c\cdot sin180\)
\(=a\cdot0+b\cdot1+c\cdot0\)
=b
c: \(a^2\cdot sin90+b^2\cdot cos90+c^2\cdot cos180\)
\(=a^2\cdot1+b^2\cdot0+c^2\left(-1\right)\)
\(=a^2-c^2\)

\(=cosa\cdot sina-1-1+sina\cdot cosa+2\)
\(=2\cdot sina\cdot cosa=sin2a\)


\(\Leftrightarrow3sin^2x-2sinx.cosx-5cos^2x=0\)
Nhận thấy \(cosx=0\) ko phải nghiệm, chia 2 vế cho \(cos^2x\)
\(3tan^2x-2tanx-5=0\)
\(\Leftrightarrow\left[{}\begin{matrix}tanx=-1\\tanx=\frac{5}{3}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=-45^0+k180^0\\x=arctan\left(\frac{5}{3}\right)+k180^0\end{matrix}\right.\)

1.
\(\Leftrightarrow2sinx.cosx+2cosx=0\)
\(\Leftrightarrow2cosx\left(sinx+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=0\\sinx=-1\end{matrix}\right.\)
\(\Leftrightarrow cosx=0\) (do \(cosx=0\Leftrightarrow sinx=\pm1\) bao hàm luôn cả pt \(sinx=-1\))
\(\Leftrightarrow x=\dfrac{\pi}{2}+k\pi\)
2.
\(\Leftrightarrow\left[{}\begin{matrix}2x-10^0=60^0+k360^0\\2x-10^0=120^0+n360^0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=35^0+k180^0\\x=65^0+n180^0\end{matrix}\right.\)
Do \(-120^0< x< 90^0\Rightarrow\left\{{}\begin{matrix}-120^0< 35^0+k180^0< 90^0\\-120^0< 65^0+n180^0< 90^0\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}k=0\\n=\left\{-1;0\right\}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=35^0\\x=-115^0\\x=65^0\end{matrix}\right.\)
3. Làm tương tự câu 2
4.
\(\Leftrightarrow\dfrac{1}{2}-\dfrac{1}{2}cos\left(10x+\dfrac{4\pi}{5}\right)-\left(\dfrac{1}{2}+\dfrac{1}{2}cos\left(\dfrac{x}{2}-2\pi\right)\right)=0\)
\(\Leftrightarrow cos\left(10x+\dfrac{4\pi}{5}\right)+cos\left(\dfrac{x}{2}-2\pi\right)=0\)
\(\Leftrightarrow cos\left(10x+\dfrac{4\pi}{5}\right)+cos\left(\dfrac{x}{2}\right)=0\)
\(\Leftrightarrow cos\left(10x+\dfrac{4\pi}{5}\right)=-cos\left(\dfrac{x}{2}\right)=cos\left(\pi-\dfrac{x}{2}\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}10x+\dfrac{4\pi}{5}=\pi-\dfrac{x}{2}+k2\pi\\10x+\dfrac{4\pi}{5}=\dfrac{x}{2}-\pi+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow...\)

a: 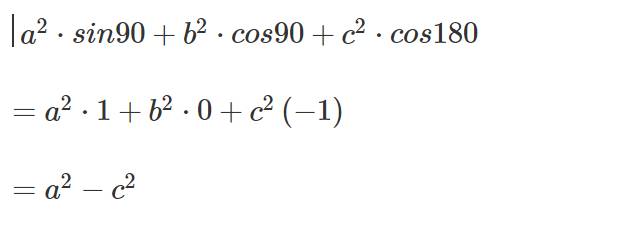
b: \(B=3-sin^290^0+2\cdot cos^260^0-3\cdot tan^245^0\)
\(=3-1+2\cdot\left(\dfrac{1}{2}\right)^2-3\cdot1^2\)
\(=2-3+2\cdot\dfrac{1}{4}=-1+\dfrac{1}{2}=-\dfrac{1}{2}\)
c: \(C=sin^245^0-2\cdot sin^250^0+3\cdot cos^245^0-2\cdot sin^240^0+4\cdot tan55\cdot tan35\)
\(=\left(\dfrac{\sqrt{2}}{2}\right)^2+3\cdot\left(\dfrac{\sqrt{2}}{2}\right)^2-2\cdot\left(sin^250^0+sin^240^0\right)+4\)
\(=\dfrac{1}{2}+3\cdot\dfrac{1}{2}-2+4\)
\(=2-2+4=4\)

= cos \(_{^{ }\beta}\).cos\(\beta\).(-cot\(\beta\)) vậy dấu của A là dấu trừ