Giải bất phương trình sau :
2x^2+10x+19>0
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



1:
a: =>3x=6
=>x=2
b: =>4x=16
=>x=4
c: =>4x-6=9-x
=>5x=15
=>x=3
d: =>7x-12=x+6
=>6x=18
=>x=3
2:
a: =>2x<=-8
=>x<=-4
b: =>x+5<0
=>x<-5
c: =>2x>8
=>x>4

`x^2+2x+3>2`
`<=>x^2+2x+1>0`
`<=>(x+1)^2>0`
`<=>x+1 ne 0`
`<=>x ne -1`
`(x+5)(3x^2+2)>0`
Vì `3x^2+2>=2>0`
`=>x+5>0<=>x>-5`
c) Ta có: \(21x-10x^2+9< 0\)
\(\Leftrightarrow10x^2-21x-9>0\)
\(\Leftrightarrow x^2-\dfrac{21}{10}x-\dfrac{9}{10}>0\)
\(\Leftrightarrow x^2-2\cdot x\cdot\dfrac{21}{20}+\dfrac{441}{400}>\dfrac{801}{400}\)
\(\Leftrightarrow\left(x-\dfrac{21}{20}\right)^2>\dfrac{801}{400}\)
\(\Leftrightarrow\left[{}\begin{matrix}x>\dfrac{3\sqrt{89}+21}{20}\\x< \dfrac{-3\sqrt{89}+21}{20}\end{matrix}\right.\)

a) Xét tam thức \(f\left( x \right) = 7{x^2} - 19x - 6\) có \(\Delta = 529 > 0\), có hai nghiệm phân biệt \({x_1} = - \frac{2}{7},{x_2} = 3\) và có \(a = 7 > 0\)
Ta có bảng xét dấu như sau
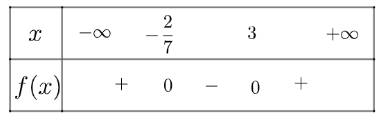
Vậy nghiệm của bất phương trình là đoạn \(\left[ { - \frac{2}{7};3} \right]\)
b) \( - 6{x^2} + 11x > 10 \Leftrightarrow - 6{x^2} + 11x - 10 > 0\)
Xét tam thức \(f\left( x \right) = - 6{x^2} + 11x - 10\) có \(\Delta = - 119 < 0\)và có \(a = - 6 < 0\)
Ta có bảng xét dấu như sau

Vậy bất phương trình vô nghiệm
c) \(3{x^2} - 4x + 7 > {x^2} + 2x + 1 \Leftrightarrow 2{x^2} - 6x + 6 > 0\)
Xét tam thức \(f\left( x \right) = 2{x^2} - 6x + 6\) có \(\Delta = - 12 < 0\)và có \(a = 2 > 0\)
Ta có bảng xét dấu như sau
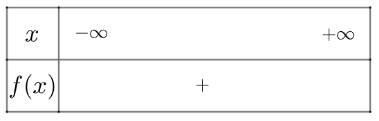
Vậy bất phương trình có vô số nghiệm
d) Xét tam thức \(f\left( x \right) = {x^2} - 10x + 25\) có \(\Delta = 0\), có nghiệm kép \({x_1} = {x_2} = 5\) và có \(a = 1 > 0\)
Ta có bảng xét dấu như sau
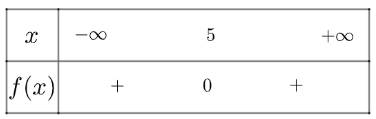
Vậy nghiệm của bất phương trình là \(x = 5\)

a) \(|2x+1|=|x-3|\)
\(\Leftrightarrow|2x+1|-|x-3|=0\)
Lập bảng xét dấu :
| x | \(\frac{-1}{2}\) | 3 | |||
| 2x+1 | - | 0 | + | \(|\) | + |
| x-3 | - | \(|\) | - | 0 | + |
Nếu \(x< \frac{-1}{2}\) thì \(|2x+1|=-2x-1\)
\(|x-3|=3-x\)
\(pt\Leftrightarrow\left(-2x-1\right)-\left(3-x\right)=0\)
\(\Leftrightarrow-2x-1-3+x=0\)
\(\Leftrightarrow-x=4\)
\(\Leftrightarrow x=-4\left(tm\right)\)
Nếu \(\frac{-1}{2}\le x\le3\) thì \(|2x+1|=2x+1\)
\(|x-3|=3-x\)
\(pt\Leftrightarrow\left(2x+1\right)-\left(3-x\right)=0\)
\(\Leftrightarrow2x+1-3+x=0\)
\(\Leftrightarrow3x-2=0\)
\(x=\frac{2}{3}\left(tm\right)\)
Nếu \(x>3\) thì \(|2x+1|=2x+1\)
\(|x-3|=x-3\)
\(pt\Leftrightarrow\left(2x+1\right)-\left(x-3\right)=0\)
\(\Leftrightarrow2x+1-x+3=0\)
\(\Leftrightarrow x=-4\) ( loại )
\(x^4+x^2+6x-8=0\)
\(\Leftrightarrow\left(x^4+2x^2+1\right)-\left(x^2-6x+9\right)=0\)
\(\Leftrightarrow\left(x^2+1\right)^2-\left(x-3\right)^2=0\)
Mà \(\left(x^2+1\right)^2\ge0\forall x\)
\(\left(x-3\right)^2\ge0\forall x\)
Dấu bằng xảy ra khi :
\(\hept{\begin{cases}x^2+1=0\\x-3=0\end{cases}}\Leftrightarrow\hept{\begin{cases}x^2=-1\\x=3\end{cases}}\)
Lại có \(x^2\ge0\forall x\)
\(\Leftrightarrow x^2=-1\) ( vô lí )
Vậy phương trình có tập nghiệm \(S=\left\{3\right\}\)

\(a,\Leftrightarrow\left(x-5\right)^2=0\Leftrightarrow x-5=0\Leftrightarrow x=5\\ b,\Leftrightarrow\left(2x-1\right)^2=0\Leftrightarrow2x-1=0\Leftrightarrow x=1\\ c,\Leftrightarrow\left(1-2x\right)^2-\left(3x-2\right)^2=0\\ \Leftrightarrow\left(1-2x-3x+2\right)\left(1-2x+3x-2\right)=0\\ \Leftrightarrow\left(3-5x\right)\left(x-1\right)=0\Leftrightarrow\left[{}\begin{matrix}x=1\\x=\dfrac{3}{5}\end{matrix}\right.\\ d,\Leftrightarrow\left(x-2\right)^3=-\left(5-2x\right)^3\\ \Leftrightarrow x-2=-\left(5-2x\right)=2x-5\\ \Leftrightarrow x=3\)

\(a,\dfrac{x-3}{x}=\dfrac{x-3}{x+3}\)\(\left(đk:x\ne0,-3\right)\)
\(\Leftrightarrow\dfrac{x-3}{x}-\dfrac{x-3}{x+3}=0\)
\(\Leftrightarrow\dfrac{\left(x-3\right)\left(x+3\right)-x\left(x-3\right)}{x\left(x+3\right)}=0\)
\(\Leftrightarrow x^2-9-x^2+3x=0\)
\(\Leftrightarrow3x-9=0\)
\(\Leftrightarrow3x=9\)
\(\Leftrightarrow x=3\left(n\right)\)
Vậy \(S=\left\{3\right\}\)
\(b,\dfrac{4x-3}{4}>\dfrac{3x-5}{3}-\dfrac{2x-7}{12}\)
\(\Leftrightarrow\dfrac{4x-3}{4}-\dfrac{3x-5}{3}+\dfrac{2x-7}{12}>0\)
\(\Leftrightarrow\dfrac{3\left(4x-3\right)-4\left(3x-5\right)+2x-7}{12}>0\)
\(\Leftrightarrow12x-9-12x+20+2x-7>0\)
\(\Leftrightarrow2x+4>0\)
\(\Leftrightarrow2x>-4\)
\(\Leftrightarrow x>-2\)
I don't know,sorry
Ta có: \(2x^2+10x+19=\left(\sqrt{2}x\right)^2+2.\sqrt{2}x.\frac{5}{\sqrt{2}}+\frac{25}{2}+\frac{13}{2}\)
\(=\left(\sqrt{2}x+\frac{5}{\sqrt{2}}\right)^2+\frac{13}{2}>0\forall x\)
Vậy tập nghiệm của bất phương trình \(T=\left\{\pm\infty\right\}\)