Tìm m để phương trình x2-2019x+m-1=0 có nghiệm x1, x2 thỏa mãn x12+2018x22=2019x1x2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
28 tháng 3 2023
x1^2+x2^2=(x1+x2)^2-2x1x2
=m^2-2(m-1)=m^2-2m+2
=>x1^2=m^2-2m+2-x2^2
x1^2+3x2=19
=>m^2-2m+2-x2^2+3x2=19
=>-x2^2+3x2+m^2-2m-17=0
=>x2^2-3x2-m^2+2m+17=0(1)
Để (1) có nghiệm thì Δ1>0
=>(-3)^2-4*1*(-m^2+2m+17)>0
=>9-4(-m^2+2m+17)>0
=>9+4m^2-8m-68>0
=>4m^2-8m-59>0
=>\(\left[{}\begin{matrix}m< \dfrac{2-3\sqrt{7}}{2}\\m>\dfrac{2+3\sqrt{7}}{2}\end{matrix}\right.\)
NT
1
AH
Akai Haruma
Giáo viên
26 tháng 2 2022
Tham khảo lời giải tại đây:
https://hoc24.vn/cau-hoi/tim-m-de-phuong-trinh-x2-7x-m-2-0-co-nghiem-x1-x2-thoa-man-x12-3x2-3.4915847121620
LD
1
AH
Akai Haruma
Giáo viên
26 tháng 2 2022
Lời giải:
Để pt có nghiệm thì: $\Delta=49-4(m-2)\geq 0$
$\Leftrightarrow m\leq 14,25$
Khi đó, áp dụng định lý Viet: $x_1+x_2=7; x_1x_2=m-2$
Để $x_1^2+3x_2=-3$
$\Leftrightarrow (7-x_2)^2+3x_2+3=0$
$\Leftrightarrow x_2^2-11x_2+52=0$
$\Leftrightarrow (x_2-5,5)^2=-21,75<0$ (vô lý)
Vậy không tồn tại $m$ thỏa điều kiện đề bài.
AH
Akai Haruma
Giáo viên
17 tháng 3 2021
Lời giải:
Để PT có 2 nghiệm $x_1,x_2$ thì:
$\Delta'=(m+1)^2-(m^2-1)>0\Leftrightarrow 2m+2>0\Leftrightarrow m>-1$
Áp dụng định lý Viet:
$x_1+x_2=2(m+1)$ và $x_1x_2=m^2-1$
Khi đó, để $x_1^2+x_2^2=x_1x_2+8$
$\Leftrightarrow (x_1+x_2)^2-2x_1x_2=x_1x_2+8$
$\Leftrightarrow (x_1+x_2)^2=3x_1x_2+8$
$\Leftrightarrow 4(m+1)^2=3(m^2-1)+8$
$\Leftrightarrow m^2+8m-1=0$
$\Leftrightarrow m=-4\pm \sqrt{17}$. Vì $m>-1$ nên $m=-4+\sqrt{17}$
DT
27 tháng 4 2023
Để phương trình có 2 nghiệm thì:
\(\Delta\ge0\)
\(m^2+10m+25-8m-24\ge0\)
\(m^2+2m+1\ge0\)
\(\left(m+1\right)^2\ge\forall m\) => Pt đã cho có 2 nghiệm với mọi giá trị m.
Theo viet: \(\left\{{}\begin{matrix}x_1+x_2=m+5\\x_1x_2=2m+6\end{matrix}\right.\)
Có:
\(x_1^2+x_2^2=35\) (đưa cái đề đàng hoàng vào.-.)
<=> \(\left(x_1+x_2\right)^2-2x_1x_2=35\)
<=> \(\left(m+5\right)^2-2.\left(2m+6\right)=35\)
<=> \(m^2+10m+25-4m-12-35=0\)
<=> \(m^2+6m-22=0\)
delta' = 32 +22 = 31 > 0
=> \(\left\{{}\begin{matrix}m_1=-3+\sqrt{31}\\m_2=-3-\sqrt{31}\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
2 tháng 7 2021
Giả sử pt có 2 nghiệm, theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2m-n\\x_1x_2=2m+3n-1\end{matrix}\right.\)
\(\left\{{}\begin{matrix}x_1+x_2=-1\\x_1^2+x_2^2=13\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x_1+x_2=-1\\\left(x_1+x_2\right)^2-2x_1x_2=13\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_1+x_2=-1\\x_1x_2=-6\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2m-n=-1\\2m+3n-1=-6\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}n=-1\\m=-1\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
16 tháng 3 2022
\(\Delta'=\left(m-5\right)^2+2m-9=m^2-8m+16=\left(m-4\right)^2\ge0;\forall m\)
Pt đã cho luôn luôn có nghiệm
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2\left(m-5\right)\\x_1x_2=-2m+9\end{matrix}\right.\)
Do \(x_1\) là nghiệm của pt nên:
\(x_1^2-2\left(m-5\right)x_1-2m+9=0\Rightarrow x_1^2=2\left(m-5\right)x_1+2m-9\)
Thay vào bài toán:
\(2\left(m-5\right)x_1+2m-9+2\left(m-5\right)x_2=4m^2\)
\(\Leftrightarrow2\left(m-5\right)\left(x_1+x_2\right)+2m-9=4m^2\)
\(\Leftrightarrow2\left(m-5\right).2\left(m-5\right)+2m-9=4m^2\)
\(\Leftrightarrow-38m+91=0\)
\(\Rightarrow m=\dfrac{91}{38}\)
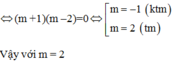
\(\Delta=2019^2-4m+4\)
\(x_1^2-x_1x_2-2018x_1x_2+2018x_2^2=0\)
\(\Leftrightarrow x_1\left(x_1-x_2\right)-2018x_2\left(x_1-x_2\right)=0\)
\(\Leftrightarrow\left(x_1-x_2\right)\left(x_1-2018x_2\right)=0\)
TH1: \(x_1=x_2\Rightarrow\Delta=0\Rightarrow2019^2-4m+4=0\Rightarrow m=\frac{2019^2+4}{4}\)
TH2: \(x_1=2018x_2\) kết hợp Viet ta có hệ:
\(\left\{{}\begin{matrix}x_1+x_2=2019\\x_1=2018x_2\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x_1=2018\\x_2=1\end{matrix}\right.\)
\(x_1x_2=m-1\Rightarrow m-1=2018\Rightarrow m=2019\)