a\(\sqrt{x-2\sqrt{x}+1}=3\)3
b,\(\sqrt{x^2-4}+2\sqrt{x-2}\)=0
giúp mk với
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(\sqrt{x}+\dfrac{1}{2\sqrt{x}}=3\)
\(\Leftrightarrow\dfrac{2\sqrt{x}\sqrt{x}}{2\sqrt{x}}+\dfrac{1}{2\sqrt{x}}=\dfrac{3.2\sqrt{x}}{2\sqrt{x}}\)
\(\Leftrightarrow\dfrac{2x}{2\sqrt{x}}-\dfrac{6\sqrt{x}}{2\sqrt{x}}+\dfrac{1}{2\sqrt{x}}=0\)
\(\Leftrightarrow2x-6\sqrt{x}+1=0\)
\(\Leftrightarrow...\)
\(\Rightarrow2x+1=6\sqrt{x}\)
\(\Rightarrow2x-6\sqrt{x}+1=0\)
\(\Rightarrow\sqrt{x}=\dfrac{3\pm\sqrt{7}}{2}\)
\(\Rightarrow x=\left(\dfrac{3\pm\sqrt{7}}{2}\right)^2=\dfrac{8\pm3\sqrt{7}}{2}\)

a: Ta có: \(\sqrt{x}< 3\)
nên \(0\le x< 9\)
b: Ta có: \(\sqrt{4x+16}+\sqrt{x+4}+2\sqrt{9x+36}=35\)
\(\Leftrightarrow2\sqrt{x+4}+\sqrt{x+4}+6\sqrt{x+4}=35\)
\(\Leftrightarrow\sqrt{x+4}=\dfrac{35}{9}\)
\(\Leftrightarrow x+4=\dfrac{1225}{81}\)
hay \(x=\dfrac{901}{81}\)
a) \(\sqrt{x}< 3\Rightarrow x< 9\)
b) \(\sqrt{4x+16}+\sqrt{x+4}+2\sqrt{9x+36}=35\)
\(\Rightarrow2\sqrt{x+4}+\sqrt{x+4}+6\sqrt{x+4}=35\)
\(\Rightarrow\sqrt{x+4}=\dfrac{35}{9}\)
\(\Rightarrow x+4=\dfrac{1225}{81}\)
\(\Rightarrow x=\dfrac{901}{81}\)
c) \(\sqrt{x+2\sqrt{x-1}}=3\)
\(\Rightarrow\sqrt{\left(x-1\right)+2\sqrt{x-1}+1}=3\)
\(\Rightarrow\sqrt{\left(x-1+1\right)^2}=3\)
\(\Rightarrow\sqrt{x^2}=3\)
\(\Rightarrow\left|x\right|=3\) \(\Rightarrow\left\{{}\begin{matrix}x=3\\x=-3\end{matrix}\right.\)

\(B=\dfrac{x+3\sqrt{x}+2+2x-4\sqrt{x}-5\sqrt{x}-2}{x-4}=\dfrac{3x-6\sqrt{x}}{x-4}=\dfrac{3\sqrt{x}}{\sqrt{x}+2}\)
B=2/3A
=>3căn x/căn x+2=2/3*3=2
=>3căn x=2căn x+4
=>x=16

a: =>2x^2-3x-2=6x+2
=>2x^2-9x-4=0
=>\(x=\dfrac{9\pm\sqrt{113}}{4}\)
b: \(=\dfrac{x-3\sqrt{x}+4-\sqrt{x}}{\sqrt{x}\left(\sqrt{x}-2\right)}=\dfrac{\left(\sqrt{x}-2\right)^2}{\sqrt{x}\left(\sqrt{x}-2\right)}=\dfrac{\sqrt{x}-2}{\sqrt{x}}\)
a) \(\dfrac{2x^2-3x-2}{2x+1}\)=3
b)B=\(\dfrac{x-3\sqrt{x}+4}{x-2\sqrt{x}}\)=\(\dfrac{1}{\sqrt{x}-2}\)

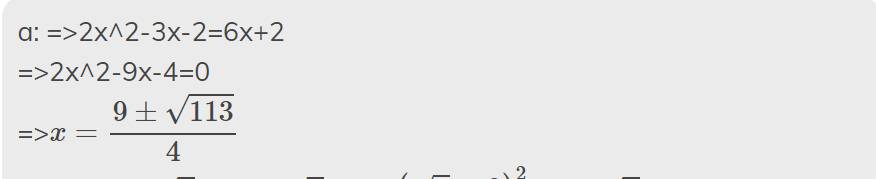
b: =>x-3căn x+4=căn x
=>(căn x-2)^2=0
=>căn x-2=0
=>x=4(loại)

a) \(\sqrt{4x^2+4x+1}=6\)
\(\Leftrightarrow\sqrt{\left(2x+1\right)^2}=6\)
\(\Leftrightarrow\left(2x+1\right)^2=6^2\)
\(\Leftrightarrow\left[{}\begin{matrix}2x+1=6\\2x+1=-6\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{2}\\x=-\dfrac{7}{2}\end{matrix}\right.\)
b) \(\sqrt{4x^2-4\sqrt{7}x+7}=\sqrt{7}\)
\(\Leftrightarrow\sqrt{\left(2x-\sqrt{7}\right)^2}=\sqrt{7}\)
\(\Leftrightarrow\left(2x-\sqrt{7}\right)^2=\left(\sqrt{7}\right)^2\)
\(\Leftrightarrow\left[{}\begin{matrix}2x-\sqrt{7}=\sqrt{7}\\2x-\sqrt{7}=-\sqrt[]{7}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\sqrt{7}\\x=0\end{matrix}\right.\)
a) \(\sqrt{4x^2+4x+1}=6\)
\(\Leftrightarrow\sqrt{\left(2x+1\right)^2}=6\)
\(\Leftrightarrow\left|2x+1\right|=6\)
\(\Leftrightarrow\left[{}\begin{matrix}2x+1=6\\2x+1=-6\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{2}\\x=-\dfrac{7}{2}\end{matrix}\right.\)
b) \(pt\Leftrightarrow\sqrt{\left(2x-\sqrt{7}\right)^2}=\sqrt{7}\)
\(\Leftrightarrow\left|2x-\sqrt{7}\right|=\sqrt{7}\)
\(\Leftrightarrow\left[{}\begin{matrix}2x-\sqrt{7}=\sqrt{7}\\2x-\sqrt{7}=-\sqrt{7}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\sqrt{7}\\x=0\end{matrix}\right.\)

a) \(\sqrt{x}< 3\)<=> x<9
b)\(\sqrt{4-x}\) ≤ 2 <=> 4 - x ≤ 4 <=> x≥0
c)\(\sqrt{x+2}=\sqrt{4-x}\) <=> x+2=4-x <=>2x=2<=>x=1
Vậy x=1
d)\(\sqrt{x^2-1}\)=x-1 <=> x\(^2\)-1=x\(^2\)-2x+1 <=> x\(^2\)-\(x^2\)-2x+1+1=0 <=> 2x=2 <=> x=1
Vậy x=1

a: Ta có: \(x-3\sqrt{x+1}=-3\)
\(\Leftrightarrow\sqrt{9x+9}=x+3\)
\(\Leftrightarrow x^2+6x+9-9x-9=0\)
\(\Leftrightarrow x\left(x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=3\end{matrix}\right.\)
a, ĐK: \(x\ge-1\)
\(x-3\sqrt{x+1}=-3\)
\(\Leftrightarrow x+3\left(1-\sqrt{x+1}\right)=0\)
\(\Leftrightarrow x-\dfrac{3x}{1+\sqrt{x+1}}=0\)
\(\Leftrightarrow x\left(1-\dfrac{3}{1+\sqrt{x+1}}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\1+\sqrt{x+1}=3\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\left(tm\right)\\x=3\left(tm\right)\end{matrix}\right.\)

a: ĐKXĐ: \(\left\{{}\begin{matrix}x\ge0\\x\ne9\end{matrix}\right.\)
b: Ta có: \(P=\left(\dfrac{2\sqrt{x}}{\sqrt{x}+3}+\dfrac{\sqrt{x}}{\sqrt{x}-3}-\dfrac{3x+3}{x-9}\right):\left(\dfrac{2\sqrt{x}-2}{\sqrt{x}-3}-1\right)\)
\(=\dfrac{2x-6\sqrt{x}+x+3\sqrt{x}-3x-3}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}:\dfrac{2\sqrt{x}-2-\sqrt{x}+3}{\sqrt{x}-3}\)
\(=\dfrac{-3\left(\sqrt{x}+1\right)}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\cdot\dfrac{\sqrt{x}-3}{\sqrt{x}+1}\)
\(=\dfrac{-3}{\sqrt{x}+3}\)
c: Thay \(x=4-2\sqrt{3}\) vào P, ta được:
\(P=\dfrac{-3}{\sqrt{3}-1+3}=\dfrac{-3}{2+\sqrt{3}}=-6+3\sqrt{3}\)
a: Để P nguyên thì \(-3⋮\sqrt{x}+3\)
\(\Leftrightarrow\sqrt{x}+3=3\)
hay x=0
\(\sqrt{x-2\sqrt{x}+1}=3\Leftrightarrow\sqrt{\left(\sqrt{x}-1\right)^2}=3\Leftrightarrow\left|\sqrt{x}-1\right|=3\)
\(\Leftrightarrow\orbr{\begin{cases}\sqrt{x}-1=3\\\sqrt{x}-1=-3\end{cases}}\Leftrightarrow\orbr{\begin{cases}\sqrt{x}=4\\\sqrt{x}=-2\left(voli\right)\end{cases}}\Rightarrow x=16\)
câu a là 3 nhé ko phải 33 đâu