Cho hình chóp đều SABC cạnh đáy a. Mặt bên tạo với đáy 1 góc 45°. Thể tích của mặt cầu ngoại tiếp hình chóp là V .Tính V
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
24 tháng 8 2021
Gọi O là tâm đáy \(\Rightarrow AO=\dfrac{a\sqrt{3}}{3}\)
\(SA=\dfrac{AO}{cos60^0}=\dfrac{2a\sqrt{3}}{3}\)
\(SO=\sqrt{SA^2-AO^2}=a\)
\(\Rightarrow R=\dfrac{SA^2}{2SO}=\dfrac{2a}{3}\)
\(V=\dfrac{4}{3}\pi R^3=\dfrac{32\pi a^3}{81}\)
\(\Rightarrow\dfrac{V}{\pi a^3}=\dfrac{32}{81}\)
NV
Nguyễn Việt Lâm
Giáo viên
24 tháng 8 2021
Gọi M là trung điểm AB \(\Rightarrow\widehat{SMO}=45^0\)
\(OM=\dfrac{1}{2}AB=a\sqrt{2}\)
\(SO=OM.tan45^0=a\sqrt{2}\)
\(OA=\dfrac{1}{2}AC=2a\)
\(\Rightarrow SA=\sqrt{SO^2+OA^2}=a\sqrt{6}\)
\(\Rightarrow R=\dfrac{SA^2}{2SO}=\dfrac{3a\sqrt{2}}{2}\)
\(V=\dfrac{4}{3}\pi R^3=9\sqrt{2}\pi a^3\)
NV
Nguyễn Việt Lâm
Giáo viên
24 tháng 8 2021
Gọi O là tâm đáy \(\Rightarrow OA=\dfrac{2}{3}.\dfrac{a\sqrt{3}}{2}=\dfrac{a\sqrt{3}}{3}\)
\(\Rightarrow SO=\sqrt{SA^2-OA^2}=\dfrac{a\sqrt[]{33}}{3}\)
\(\Rightarrow R=\dfrac{SA^2}{2SO}=\dfrac{2a\sqrt{33}}{11}\)
\(V=\dfrac{4}{3}\pi R^3=\dfrac{32a^3\sqrt{3}\pi}{11\sqrt{11}}\)
NV
Nguyễn Việt Lâm
Giáo viên
24 tháng 8 2021
\(AC=2a\sqrt{2}.\sqrt{2}=4a\) \(\Rightarrow OA=\dfrac{1}{2}AC=2a\)
\(\widehat{SAO}=30^0\Rightarrow\left\{{}\begin{matrix}SO=AO.tan30^0=\dfrac{2a\sqrt{3}}{3}\\SA=\dfrac{AO}{cos30^0}=\dfrac{4a\sqrt{3}}{3}\end{matrix}\right.\)
\(\Rightarrow R=\dfrac{SA^2}{2SO}=\dfrac{4a\sqrt{3}}{3}\)
\(V=\dfrac{4}{3}\pi R^3=\dfrac{256\pi a^3\sqrt{3}}{27}\)
NV
Nguyễn Việt Lâm
Giáo viên
24 tháng 8 2021
Kẻ \(AH\perp BC\)
Áp dụng hệ thức lượng: \(\dfrac{1}{AH^2}=\dfrac{1}{AB^2}+\dfrac{1}{AC^2}=\dfrac{4}{3a^2}\Rightarrow AH=\dfrac{a\sqrt{3}}{2}\)
\(tan\widehat{SHA}=\dfrac{2}{\sqrt{3}}=\dfrac{SA}{AH}\Rightarrow SA=\dfrac{AH.2}{\sqrt{3}}=a\)
Gọi M là trung điểm BC và N là trung điểm SA, dựng hình chữ nhật AMIN \(\Rightarrow\) I là tâm mặt cầu ngoại tiếp
\(AN=\dfrac{1}{2}SA=\dfrac{a}{2}\) ; \(AM=\dfrac{1}{2}BC=\dfrac{1}{2}\sqrt{AB^2+AC^2}=a\)
\(\Rightarrow R=IA=\sqrt{AM^2+AN^2}=\dfrac{a\sqrt{5}}{2}\)
\(V=\dfrac{4}{3}\pi R^3=...\)
CM
3 tháng 2 2018
Đáp án A
Gọi O là tâm của tam giác A B C ⇒ S A ; A B C ^ = S A ; O A ^ = S A O ^ = 60 °
tam giác SAO vuông tại O, có
tan S A O ^ = S O O A ⇒ S O = tan 60 ° . a 3 3 = a ⇒ S A = O A 2 + S O 2 = 2 a 3 3
bán kính mặt cầu ngoại tiếp khối chóp là R = S A 2 2. S O = 2 a 3
vậy thể tích cần tính là V = 4 3 π R 3 = 4 3 π 2 a 3 3 = 32 π a 3 81
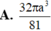
Gọi O là tâm đáy, M là trung điểm AB
\(OA=\dfrac{a\sqrt{3}}{3}\) ; \(OM=\dfrac{1}{2}OA=\dfrac{a\sqrt{3}}{6}\)
\(\widehat{SMO}=45^0\Rightarrow SO=OM=\dfrac{a\sqrt{3}}{6}\)
\(SA=\sqrt{SO^2+OA^2}=\dfrac{a\sqrt{15}}{6}\)
\(\Rightarrow R=\dfrac{SA^2}{2SO}=\dfrac{5a\sqrt{3}}{12}\)
\(V=\dfrac{4}{3}\pi R^3=\dfrac{125\pi a^3\sqrt{3}}{432}\)