Tính giá trị lượng giác của các góc sau đây :
a) \(120^0\)
b) \(150^0\)
c) \(135^0\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a) \(\left\{{}\begin{matrix}sin120^o=\dfrac{\sqrt{3}}{2}\\cos120^o=-\dfrac{1}{2}\\tan120^o=-\sqrt{3}\\cot120^o=-\dfrac{\sqrt{3}}{3}\end{matrix}\right.\)
b) \(\left\{{}\begin{matrix}sin150^o=\dfrac{1}{2}\\cos150^o=-\dfrac{\sqrt{3}}{2}\\tan150^o=-\dfrac{\sqrt{3}}{3}\\cot150^o=-\sqrt{3}\end{matrix}\right.\)
c) \(\left\{{}\begin{matrix}sin180^o=0\\cos180^o=-1\\tan180^o=0\\cot180^o\left(\varnothing\right)\end{matrix}\right.\)

\(\begin{array}{l}\sin {120^o} = \sin \;({180^o} - {60^o}) = \sin {60^o} = \frac{{\sqrt 3 }}{2};\\\cos {150^o} = - \cos \;({180^o} - {30^o}) = - \cos {30^o} = - \frac{{\sqrt 3 }}{2};\\\cot {135^o} = - \cot \;({180^o} - {45^o}) = - \cot {45^o} = - 1.\end{array}\)

Ta có:
\(\cos {15^0} = \cos \left( {{{45}^0} - {{30}^0}} \right) = \cos {45^0}\cos {30^0} + \sin {45^0}\sin {30^0} = \frac{{\sqrt 2 }}{2}.\frac{{\sqrt 3 }}{2} + \frac{{\sqrt 2 }}{2}.\frac{1}{2} = \frac{{\sqrt 6 + \sqrt 2 }}{4}\)
\(\sin {15^0} = \sin \left( {{{45}^0} - {{30}^0}} \right) = \sin {45^0}\cos {30^0} - \cos {45^0}\sin {30^0} = \frac{{\sqrt 2 }}{2}.\frac{{\sqrt 3 }}{2} - \frac{{\sqrt 2 }}{2}.\frac{1}{2} = \frac{{\sqrt 6 - \sqrt 2 }}{4}\)
\(\tan {15^0} = \tan \left( {{{45}^0} - {{30}^0}} \right) = \frac{{\tan {{45}^0} - \tan {{30}^0}}}{{1 + \tan {{45}^0}\tan {{30}^0}}} = \frac{{1 - \frac{{\sqrt 3 }}{3}}}{{1 + \frac{{\sqrt 3 }}{3}}} = 2 - \sqrt 3 \)
\(\cot {15^0} = \frac{1}{{\tan {{15}^0}}} = \frac{1}{{2 - \sqrt 3 }}\)

\(a,cos\left(\dfrac{21\pi}{6}\right)=cos\left(3\pi+\dfrac{\pi}{2}\right)=cos\left(\pi+\dfrac{\pi}{2}\right)=-cos\left(\dfrac{\pi}{2}\right)=0\\ b,sin\left(\dfrac{129\pi}{4}\right)=sin\left(32\pi+\dfrac{\pi}{4}\right)=sin\left(\dfrac{\pi}{4}\right)=\dfrac{\sqrt{2}}{2}\\ c,tan\left(1020^o\right)=tan\left(5\cdot180^o+120^o\right)=tan\left(120^o\right)=-\sqrt{3}\)


a)
Đặt \(A = \left( {2\sin {{30}^o} + \cos {{135}^o} - 3\tan {{150}^o}} \right).\left( {\cos {{180}^o} - \cot {{60}^o}} \right)\)
Ta có: \(\left\{ \begin{array}{l}\cos {135^o} = - \cos {45^o};\cos {180^o} = - \cos {0^o}\\\tan {150^o} = - \tan {30^o}\end{array} \right.\)
\( \Rightarrow A = \left( {2\sin {{30}^o} - \cos {{45}^o} + 3\tan {{30}^o}} \right).\left( { - \cos {0^o} - \cot {{60}^o}} \right)\)
Sử dụng bảng giá trị lượng giác của một số góc đặc biệt, ta có:
\(\left\{ \begin{array}{l}\sin {30^o} = \frac{1}{2};\tan {30^o} = \frac{{\sqrt 3 }}{3}\\\cos {45^o} = \frac{{\sqrt 2 }}{2};\cos {0^o} = 1;\cot {60^o} = \frac{{\sqrt 3 }}{3}\end{array} \right.\)
\( \Rightarrow A = \left( {2.\frac{1}{2} - \frac{{\sqrt 2 }}{2} + 3.\frac{{\sqrt 3 }}{3}} \right).\left( { - 1 - \frac{{\sqrt 3 }}{3}} \right)\)
\(\begin{array}{l} \Leftrightarrow A = - \left( {1 - \frac{{\sqrt 2 }}{2} + \sqrt 3 } \right).\left( {1 + \frac{{\sqrt 3 }}{3}} \right)\\ \Leftrightarrow A = - \frac{{2 - \sqrt 2 + 2\sqrt 3 }}{2}.\frac{{3 + \sqrt 3 }}{3}\\ \Leftrightarrow A = - \frac{{\left( {2 - \sqrt 2 + 2\sqrt 3 } \right)\left( {3 + \sqrt 3 } \right)}}{6}\\ \Leftrightarrow A = - \frac{{6 + 2\sqrt 3 - 3\sqrt 2 - \sqrt 6 + 6\sqrt 3 + 6}}{6}\\ \Leftrightarrow A = - \frac{{12 + 8\sqrt 3 - 3\sqrt 2 - \sqrt 6 }}{6}.\end{array}\)
b)
Đặt \(B = {\sin ^2}{90^o} + {\cos ^2}{120^o} + {\cos ^2}{0^o} - {\tan ^2}60 + {\cot ^2}{135^o}\)
Ta có: \(\left\{ \begin{array}{l}\cos {120^o} = - \cos {60^o}\\\cot {135^o} = - \cot {45^o}\end{array} \right. \Rightarrow \left\{ \begin{array}{l}{\cos ^2}{120^o} = {\cos ^2}{60^o}\\{\cot ^2}{135^o} = {\cot ^2}{45^o}\end{array} \right.\)
\( \Rightarrow B = {\sin ^2}{90^o} + {\cos ^2}{60^o} + {\cos ^2}{0^o} - {\tan ^2}60 + {\cot ^2}{45^o}\)
Sử dụng bảng giá trị lượng giác của một số góc đặc biệt, ta có:
\(\left\{ \begin{array}{l}\cos {0^o} = 1;\;\;\cot {45^o} = 1;\;\;\cos {60^o} = \frac{1}{2}\\\tan {60^o} = \sqrt 3 ;\;\;\sin {90^o} = 1\end{array} \right.\)
\( \Rightarrow B = {1^2} + {\left( {\frac{1}{2}} \right)^2} + {1^2} - {\left( {\sqrt 3 } \right)^2} + {1^2}\)
\( \Leftrightarrow B = 1 + \frac{1}{4} + 1 - 3 + 1 = \frac{1}{4}.\)
c
Đặt \(C = \cos {60^o}.\sin {30^o} + {\cos ^2}{30^o}\)
Sử dụng bảng giá trị lượng giác của một số góc đặc biệt, ta có:
\(\sin {30^o} = \frac{1}{2};\;\;\cos {30^o} = \frac{{\sqrt 3 }}{2};\;\cos {60^o} = \frac{1}{2}\;\)
\( \Rightarrow C = \frac{1}{2}.\frac{1}{2} + {\left( {\;\frac{{\sqrt 3 }}{2}} \right)^2} = \frac{1}{4} + \frac{3}{4} = 1.\)

Với 0 < α < π/2 thì cosα >0, sinα >0. Ta có
1 - sin 2 α = cos 2 α
Mặt khác cos 2 α = ( 2 sin α ) 2 = 4 sin 2 α nên 5 sin 2 α = 1 hay
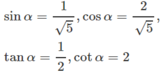
a) \(sin120^o=sin60^o=\dfrac{\sqrt{3}}{2};cos120^o=-cos60^o=-\dfrac{1}{2}\);
\(tan120^o=-\sqrt{3};cot120^o=\dfrac{-1}{\sqrt{3}}\).
b) \(sin150^o=sin30^o=\dfrac{1}{2};cos150^o=-cos30^o=-\dfrac{\sqrt{3}}{2}\).
\(tan150^o=-tan30^o=-\dfrac{\sqrt{3}}{3}\); \(cot150^o=-cot30^o=-\sqrt{3}\).
c)\(sin135^o=sin45^o=\dfrac{\sqrt{2}}{2};cos135^o=-cos45^o=-\dfrac{\sqrt{2}}{2}\).
\(tan135^o=-tan45^o=-1\); \(cot135^o=-1\).
Làm sao bạn tính ra vậy