giải phương trình \(\sqrt{6-x}+\sqrt{x+3}-\sqrt{\left(6-x\right)\left(x+3\right)}=0\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


ĐKXĐ: \(-3\le x\le6\)
Đặt \(\sqrt{3+x}=a;\sqrt{6-x}=b\left(a,b\ge0\right)\),ta có
\(\hept{\begin{cases}a+b-ab=3\left(1\right)\\a^2+b^2=9\end{cases}\Rightarrow\hept{\begin{cases}2a+2b-2ab=6\\\left(a+b\right)^2-2ab=9\end{cases}}}\)
\(\Rightarrow\left(a+b\right)^2-2\left(a+b\right)=3\Rightarrow\left(a+b\right)^2-2\left(a+b\right)-3=0\)
\(\Rightarrow\left(a+b-3\right)\left(a+b+1\right)=0\)
Do \(a,b\ge0\)nên a+b+1>0
\(\Rightarrow a+b-3=0\)\(\Rightarrow a+b=3\)thay vào (1) ta được \(ab=0\Rightarrow\hept{\begin{cases}a+b=3\\ab=0\end{cases}\Rightarrow\hept{\begin{cases}a=0\\b=3\end{cases}}}\)hoặc \(\hept{\begin{cases}a=3\\b=0\end{cases}}\)
Sau đó bn tự thay vào rồi giải tiếp nhé

1) \(\sqrt[]{9\left(x-1\right)}=21\)
\(\Leftrightarrow9\left(x-1\right)=21^2\)
\(\Leftrightarrow9\left(x-1\right)=441\)
\(\Leftrightarrow x-1=49\Leftrightarrow x=50\)
2) \(\sqrt[]{1-x}+\sqrt[]{4-4x}-\dfrac{1}{3}\sqrt[]{16-16x}+5=0\)
\(\Leftrightarrow\sqrt[]{1-x}+\sqrt[]{4\left(1-x\right)}-\dfrac{1}{3}\sqrt[]{16\left(1-x\right)}+5=0\)
\(\)\(\Leftrightarrow\sqrt[]{1-x}+2\sqrt[]{1-x}-\dfrac{4}{3}\sqrt[]{1-x}+5=0\)
\(\Leftrightarrow\sqrt[]{1-x}\left(1+3-\dfrac{4}{3}\right)+5=0\)
\(\Leftrightarrow\sqrt[]{1-x}.\dfrac{8}{3}=-5\)
\(\Leftrightarrow\sqrt[]{1-x}=-\dfrac{15}{8}\)
mà \(\sqrt[]{1-x}\ge0\)
\(\Leftrightarrow pt.vô.nghiệm\)
3) \(\sqrt[]{2x}-\sqrt[]{50}=0\)
\(\Leftrightarrow\sqrt[]{2x}=\sqrt[]{50}\)
\(\Leftrightarrow2x=50\Leftrightarrow x=25\)
1) \(\sqrt{9\left(x-1\right)}=21\) (ĐK: \(x\ge1\))
\(\Leftrightarrow3\sqrt{x-1}=21\)
\(\Leftrightarrow\sqrt{x-1}=7\)
\(\Leftrightarrow x-1=49\)
\(\Leftrightarrow x=49+1\)
\(\Leftrightarrow x=50\left(tm\right)\)
2) \(\sqrt{1-x}+\sqrt{4-4x}-\dfrac{1}{3}\sqrt{16-16x}+5=0\) (ĐK: \(x\le1\))
\(\Leftrightarrow\sqrt{1-x}+2\sqrt{1-x}-\dfrac{4}{3}\sqrt{1-x}+5=0\)
\(\Leftrightarrow\dfrac{5}{3}\sqrt{1-x}+5=0\)
\(\Leftrightarrow\dfrac{5}{3}\sqrt{1-x}=-5\) (vô lý)
Phương trình vô nghiệm
3) \(\sqrt{2x}-\sqrt{50}=0\) (ĐK: \(x\ge0\))
\(\Leftrightarrow\sqrt{2x}=\sqrt{50}\)
\(\Leftrightarrow2x=50\)
\(\Leftrightarrow x=\dfrac{50}{2}\)
\(\Leftrightarrow x=25\left(tm\right)\)
4) \(\sqrt{4x^2+4x+1}=6\)
\(\Leftrightarrow\sqrt{\left(2x+1\right)^2}=6\)
\(\Leftrightarrow\left|2x+1\right|=6\)
\(\Leftrightarrow\left[{}\begin{matrix}2x+1=6\left(ĐK:x\ge-\dfrac{1}{2}\right)\\2x+1=-6\left(ĐK:x< -\dfrac{1}{2}\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=5\\2x=-7\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{2}\left(tm\right)\\x=-\dfrac{7}{2}\left(tm\right)\end{matrix}\right.\)
5) \(\sqrt{\left(x-3\right)^2}=3-x\)
\(\Leftrightarrow\left|x-3\right|=3-x\)
\(\Leftrightarrow x-3=3-x\)
\(\Leftrightarrow x+x=3+3\)
\(\Leftrightarrow x=\dfrac{6}{2}\)
\(\Leftrightarrow x=3\)

ĐK: \(-3\le x\le6.\)
Đặt \(\hept{\begin{cases}\sqrt{3+x}=a\\\sqrt{6-x}=b\end{cases}\Rightarrow\hept{\begin{cases}a^2+b^2=9\\a+b-ab=3\end{cases}\Rightarrow}\hept{\begin{cases}\left(a+b\right)^2-2ab=9\\\left(a+b\right)-ab=3\end{cases}}}\)
Đặt \(\hept{\begin{cases}a+b=u\\ab=v\end{cases}\left(u,v\ge0\right)\Rightarrow\hept{\begin{cases}u^2-2v=9\\u-v=3\end{cases}\Rightarrow}\hept{\begin{cases}u^2-2u-3=0\\v=u-3\end{cases}}}\)
\(\Rightarrow\hept{\begin{cases}u=3\\v=0\end{cases}\Rightarrow\hept{\begin{cases}a+b=3\\ab=0\end{cases}}}\)
Th1: \(\hept{\begin{cases}a=3\\b=0\end{cases}\Rightarrow\hept{\begin{cases}\sqrt{3+x}=3\\\sqrt{6-x}=0\end{cases}\Rightarrow}x=6\left(tmđk\right).}\)
Th2: \(\hept{\begin{cases}a=0\\b=3\end{cases}\Rightarrow\hept{\begin{cases}\sqrt{3+x}=0\\\sqrt{6-x}=3\end{cases}\Rightarrow}x=-3}\left(tmđk\right).\)
Vậy x = 6 hoặc x = -3.

ĐK: \(x\ge1\)
\(pt\Leftrightarrow2\sqrt{\left(x-1\right)\left(x+2\right)}-\sqrt{x-1}-6\sqrt{x+2}+3=0\)
\(\Leftrightarrow\left(2\sqrt{x+2}-1\right)\left(\sqrt{x-1}-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2\sqrt{x+2}=1\\\sqrt{x-1}=3\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}4\left(x+2\right)=1\\x-1=9\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{7}{4}\left(l\right)\\x=10\left(tm\right)\end{matrix}\right.\)
Vậy ...
Xét \(f\left(x;y;z\right)=\left(3x+4y+5z\right)^2-44\left(xy+yz+zx\right)\)
\(=\left(y+2z+3\right)^2-44yz-44\left(y+z\right)\left(1-y-z\right)\)
\(=45y^2+2y\left(24z-19\right)+48z^2-32z+9\)
\(\Delta_y'=\left(24z-9\right)^2-45\left(48z^2-32z+9\right)=-44\left(6z-1\right)^2\le0\)
\(\Rightarrow f\left(x;y;z\right)\ge0\)

Đặt \(\hept{\begin{cases}\sqrt[6]{x-3}=a\\\sqrt[6]{x-7}=b\end{cases}}\)
\(\Rightarrow a^2+b^2-6ab=0\)
Dễ thây a = 0 không là nghiệm.
Đặt \(b=ta\)
\(\Rightarrow a^2+t^2a^2-6ta^2=0\)
\(\Leftrightarrow t^2-6t+1=0\)
Làm nôt
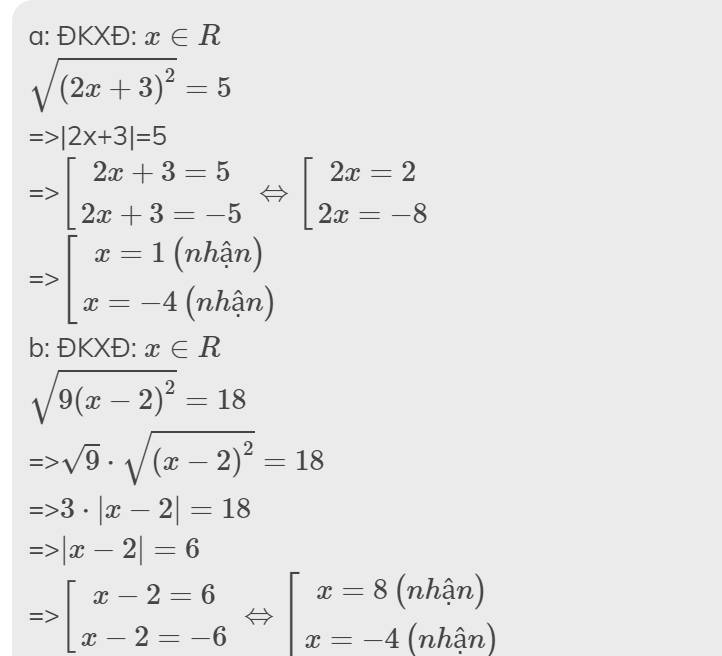
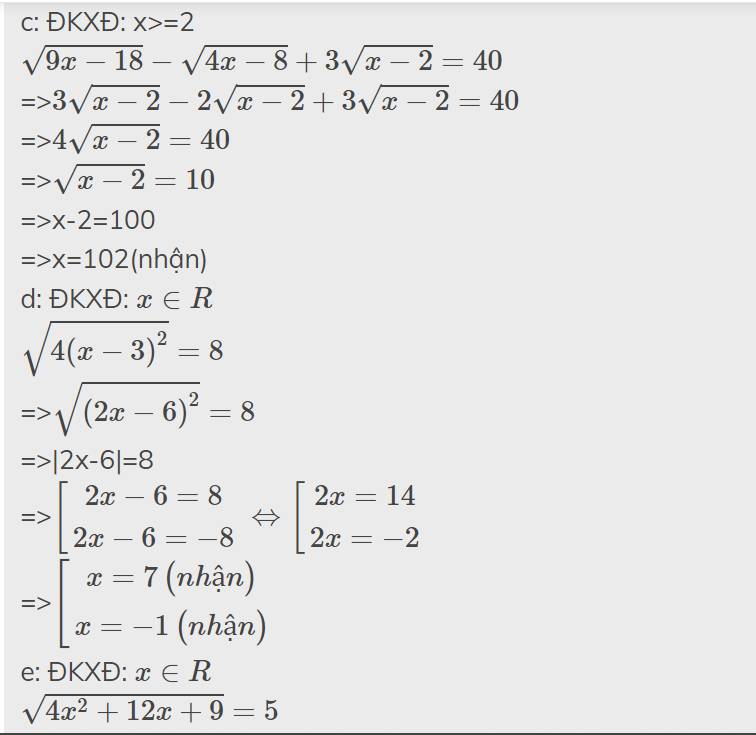
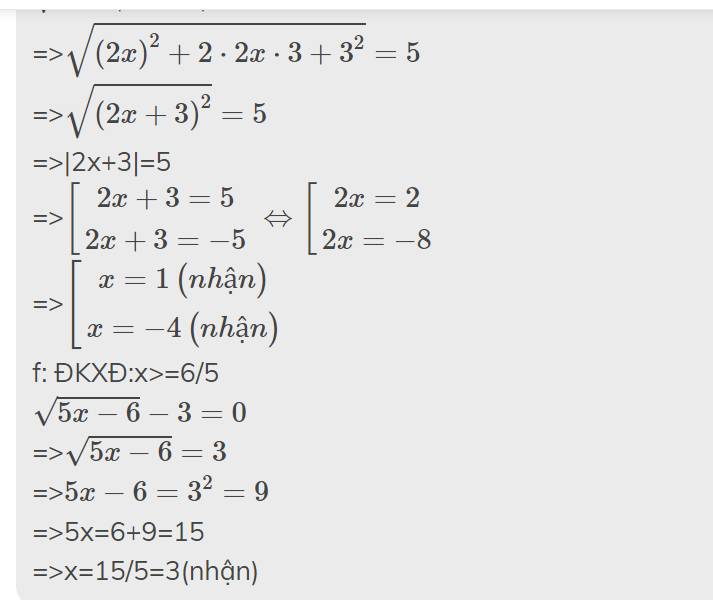
Điều kiện \(-3\le x\le6\)
Đặt \(t=\sqrt{6-x}+\sqrt{x+3}\to t^2=9+2\sqrt{\left(6-x\right)\left(x+3\right)}\to\sqrt{\left(6-x\right)\left(x+3\right)}=\frac{t^2-9}{2}\)
Vậy ta có phương trình \(t-\frac{t^2-9}{2}=0\leftrightarrow t^2-2t-9=0\leftrightarrow t=1\pm\sqrt{10}\). Vì \(t\ge0\to t=1+\sqrt{10}\to\sqrt{\left(6-x\right)\left(x+3\right)}=\frac{\left(1+\sqrt{10}\right)^2-9}{2}\to\cdots\)