Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(\Leftrightarrow2x^2+10x-x^2+6x-9=x^2+6\)
=>16x-9=6
=>16x=15
hay x=15/16
\(PT\Leftrightarrow2x^2+10x-x^2+6x-9-x^2-6=0.\)
\(\Leftrightarrow16x-15=0.\\ \Leftrightarrow x=\dfrac{15}{16}.\)


c: \(12\cdot3^x+3\cdot15^x-5^{x+1}=20\)
=>\(12\cdot3^x+3\cdot3^x\cdot5^x-5^x\cdot5-20=0\)
=>\(3^x\cdot3\left(5^x+4\right)-5\left(5^x+4\right)=0\)
=>\(\left(3^{x+1}-5\right)\left(5^x+4\right)=0\)
=>\(3^{x+1}-5=0\)
=>\(3^{x+1}=5\)
=>\(x+1=log_35\)
=>\(x=log_35-1\)
f: \(25^x-2\left(3-x\right)\cdot5^x+2x-7=0\)
=>\(\left(5^x\right)^2+5^x\cdot\left(2x-6\right)+2x-7=0\)
=>\(\left(5^x\right)^2+5^x\left(2x-7\right)+5^x+2x-7=0\)
=>\(5^x\left(5^x+2x-7\right)+\left(5^x+2x-7\right)=0\)
=>\(\left(5^x+1\right)\left(5^x+2x-7\right)=0\)
=>\(5^x+2x-7=0\)
Đặt \(A\left(x\right)=5^x+2x-7\)
=>\(A'\left(x\right)=5^x\cdot ln5+2>0\forall x\)
=>A(x) đồng biến trên R
=>A(x)=0 khi và chỉ khi x=1
i: \(9^x+2\left(x-2\right)\cdot3^x+2x-5=0\)
=>\(\left(3^x\right)^2+3^x\left(2x-5\right)+3^x+2x-5=0\)
=>\(\left(3^x+2x-5\right)\left(3^x+1\right)=0\)
=>\(3^x+2x-5=0\)
Đặt \(B\left(x\right)=3^x+2x-5\)
=>\(B'\left(x\right)=3^x\cdot ln3+2>0\)
=>B(x) luôn đồng biến trên R
=>B(x)=0 khi và chỉ khi x=1

ĐKXĐ: \(x\ge3\)
\(pt\Leftrightarrow5\sqrt{x-3}+3\sqrt{x-3}-\sqrt{x-3}=7\)
\(\Leftrightarrow7\sqrt{x-3}=7\Leftrightarrow\sqrt{x-3}=1\)
\(\Leftrightarrow x-3=1\Leftrightarrow x=4\left(tm\right)\)

\(\Leftrightarrow2cos4x\left(cos2x-sin2x\right)=0\)
\(\Leftrightarrow cos4x=0\) (do \(cos4x=cos^22x-sin^22x\) đã bao hàm \(cos2x-sin2x\))
\(\Rightarrow4x=\dfrac{\pi}{2}+k\pi\)
\(\Rightarrow x=\dfrac{\pi}{8}+\dfrac{k\pi}{4}\)

\(\dfrac{140}{x}-\dfrac{140}{x-6}=\dfrac{-1}{6}\)
\(\Leftrightarrow\dfrac{140\left(x-6\right)-140x}{x\left(x-6\right)}=-\dfrac{1}{6}\)
\(\Leftrightarrow\dfrac{-840}{x\left(x-6\right)}=-\dfrac{1}{6}\)
\(\Leftrightarrow x^2-6x-5040=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=74.05\\x=-68.05\end{matrix}\right.\)
Lời giải:
\(\frac{140}{x}-\frac{140}{x-6}=\frac{-1}{6}\Leftrightarrow \frac{140(x-6-x)}{x(x-6)}=\frac{-1}{6}\)
\(\Leftrightarrow x(x-6)=5040\Leftrightarrow x^2-6x-5040=0\)
\(\Leftrightarrow (x-3)^2-5049=0\)
\(\Leftrightarrow (x-3-\sqrt{5049})(x-3+\sqrt{5049})=0\Rightarrow x=3\pm \sqrt{5049}\)

a: \(\Leftrightarrow4\left(2x+1\right)-3\left(6x-1\right)=2x+1\)
=>8x+4-18x+3=2x+1
=>-10x+7=2x+1
=>-12x=-6
hay x=1/2
b: \(\Leftrightarrow4x^2-12x+7x-21-x^2=3x^2+6x\)
=>5x-21=6x
=>-x=21
hay x=-21

\(\sqrt{x+3}-\sqrt{7-x}>\sqrt{2x-8}\)
⇔ \(\sqrt{x+3}>\sqrt{7-x}+\sqrt{2x-8}\)
⇔ \(\left\{{}\begin{matrix}4\le x\le8\\x+3>7-x+2x-8+2\sqrt{\left(7-x\right)\left(2x-8\right)}\end{matrix}\right.\)
⇔ \(\left\{{}\begin{matrix}4\le x\le8\\x+3>x-1+2\sqrt{\left(7-x\right)\left(2x+8\right)}\end{matrix}\right.\)
⇔ \(\left\{{}\begin{matrix}4\le x\le8\\4>2\sqrt{\left(7-x\right)\left(2x+8\right)}\end{matrix}\right.\)
⇔ \(\left\{{}\begin{matrix}4\le x\le8\\\sqrt{\left(7-x\right)\left(2x-8\right)}< 2\end{matrix}\right.\)
⇔ \(\left\{{}\begin{matrix}4\le x\le8\\-2x^2+22x-56< 2\end{matrix}\right.\)
⇔ \(\left\{{}\begin{matrix}4\le x\le8\\\left[{}\begin{matrix}x>\dfrac{11+\sqrt{5}}{2}\\x< \dfrac{11-\sqrt{5}}{2}\end{matrix}\right.\end{matrix}\right.\)
⇔ \(\left[{}\begin{matrix}4\le x< \dfrac{11-\sqrt{5}}{2}\\\dfrac{11+\sqrt{5}}{2}< x\le8\end{matrix}\right.\)
Các giá trị nguyên của x thỏa mãn là S = {4 ; 7 ; 8}
Ấy chết sai điều kiện XĐ rồi, bạn sửa lại điều kiện thôi nhé

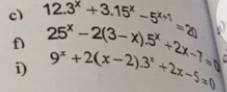
n :6 = 7(dư 3)
n = 7x6 + 3
n = 45
n : 6 = 7 ( dư 3 )
=> ( n - 3 ) : 6 = 7
=> n - 3 = 7 x 6
=> n - 3 = 42
=> n = 42 + 3
=> n = 45