Hãy giải hệ phương trình (5).
⎧⎪⎨⎪⎩x+3y−2z=−14y+3z=322z=3
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

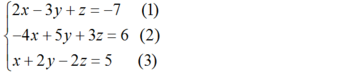
Đưa hệ phương trình về hệ dạng tam giác bằng cách khử dần các ẩn.
Nhân phương trình (1) với 2 rồi cộng với phương trình (2) và nhân phương trình (3) với 4 rồi cộng với phương trình (2) ta được:
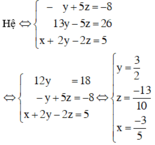
Vậy hệ phương trình có nghiệm 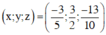



a) \(\left\{{}\begin{matrix}x+2y-3z=2\\2x+7y+z=5\\-3x+3y-2z=-7\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}x+2y-3z=2\\3y+7z=1\\-32z=-4\end{matrix}\right.\)
Đáp số : \(\left(x,y,z\right)=\left(\dfrac{55}{24},\dfrac{1}{24},\dfrac{1}{8}\right)\)
b) \(\left\{{}\begin{matrix}-x-3y+4z=3\\3x+4y-2z=5\\2x+y+2z=4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-x-3y+4z=3\\-5y+10z=14\\-5y+10z=10\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-x-3y+4z=3\\-5y+10z=14\\0y+0z=-4\end{matrix}\right.\)
Phương trình cuối vô nghiệm, suy ra hệ phương trình đã cho vô nghiệm

Bạn xem lại đề, ở pt thứ nhất là \(3x-2y+5x=14\) hay \(3x-2y+5z=14\)


\(\Leftrightarrow\left\{{}\begin{matrix}2x+2y+4z=8\\2x-y+3z=6\\2x-6y+8z=14\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x+y+2z=4\\3y+z=2\\8y-4z=-6\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x+y+2z=4\\12y+4z=8\\8y-4z=-6\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x+y+2z=4\\20y=-2\\3y+z=2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x+y+2z=4\\y=-\dfrac{1}{10}\\z=2-3y=2+\dfrac{3}{10}=\dfrac{23}{10}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-\dfrac{1}{2}\\y=-\dfrac{1}{10}\\z=\dfrac{23}{10}\end{matrix}\right.\)
\(\hept{\begin{cases}x+3y+2z=-1\left(1\right)\\4y+3x=1,5\left(2\right)\\2z=3\left(3\right)\end{cases}}\)
\(\left(3\right)\Rightarrow z=\frac{3}{2}\)Thay vào pt (1) ta được:
hệ phương trình có dạng \(\hept{\begin{cases}x+3y+3=-1\\4y+3x=1,5\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x+3y=-4\\3x+4y=1,5\end{cases}\Leftrightarrow\hept{\begin{cases}3x+9y=-12\\3x+4y=1,5\end{cases}}}\)
\(\Leftrightarrow\hept{\begin{cases}5y=-\frac{27}{2}\\x+3y=-4\end{cases}\Leftrightarrow\hept{\begin{cases}y=-\frac{27}{10}\\x=-4-3.\left(-\frac{27}{10}\right)=\frac{41}{10}\end{cases}}}\)
Vậy hệ pt có một nghiệm ( x ; y ; z ) = ( \(\frac{41}{10};-\frac{27}{10};\frac{3}{2}\))
Ta có 2z = 3
=> z = 1,5
Khi đó x + 3y + 2z = -1
<=> x + 3y + 3 = -1
<=> x + 3y = -4
<=> 3x + 9y = -12
<=> 3x + 4y + 5y = -12
<=> 1,5 + 5y = -12
<=> y = -2,7
=> x = [1,5 - 4.(-2,7)] : 3 = 4,1
Vậy x = 4,1 ; y = -2,7 ; z = 1,5