Biết x+ (1/x) =3. Giá trị của biểu thức x4+ (1/ x4) là bao nhiêu ?
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Đặt \(g\left(x\right)=f\left(x\right)-10\) (bậc 4)
\(\Leftrightarrow\left\{{}\begin{matrix}g\left(1\right)=0\\g\left(2\right)=0\\g\left(3\right)=0\end{matrix}\right.\Leftrightarrow g\left(x\right)=\left(x-1\right)\left(x-2\right)\left(x-3\right)\left(x-m\right)\) (m là hằng số)
\(\Leftrightarrow f\left(x\right)=\left(x-1\right)\left(x-2\right)\left(x-3\right)\left(x-m\right)-10\\ \Leftrightarrow f\left(9\right)=8\cdot7\cdot6\left(9-m\right)-10=336\left(9-m\right)-10\\ f\left(-5\right)=\left(-6\right)\left(-7\right)\left(-8\right)\left(-5-m\right)-10=336\left(m+5\right)-10\)
Vậy \(A=336\left(9-m\right)+336\left(m+5\right)-20=4684\)
Chúc bạn hok tốt <3

Ta có: \(M=x^4-xy^3+xy^3-y^4-1\)
\(=x^4-y^4-1\)
\(=\left(x^2-y^2\right)\left(x^2+y^2\right)-1\)
\(=\left(x+y\right)\left(x-y\right)\left(x^2+y^2\right)-1\)(1)
Thay x+y=0 vào biểu thức (1), ta được:
\(M=0-1=-1\)
Vậy: Khi x+y=0 thì M=-1
`M=x^4-xy^3+xy^3-y^4-1`
`=x(x^3+y^3)-y^3(x+y)-1`
`=x(x+y)(x^2-xy+y^2)-0-1`(do `x+y=0`)
`=0-0-1`
`=-1`

a) \(A=y\left(x^2-y^2\right)\left(x^2+y^2\right)-y\left(x^4-y^4\right)=y\left(x^4-y^4\right)-y\left(x^4-y^4\right)=0\)
b) \(B=\left(x-1\right)^3-\left(x-1\right)\left(x^2+x+1\right)-3\left(1-x\right)x=x^3-3x^2+3x-1-x^3-x^2-x+x^2+x+1-3x+3x^2=0\)
a: Ta có: \(A=y\left(x^2-y^2\right)\left(x^2+y^2\right)-y\left(x^4-y^4\right)\)
\(=y\left(x^4-y^4\right)-y\left(x^4-y^4\right)\)
=0
b: Ta có: \(B=\left(x-1\right)^3-\left(x-1\right)\left(x^2+x+1\right)-3x\left(1-x\right)\)
\(=x^3-3x^2+3x-1-x^3+1-3x+3x^2\)
=0

Ta có
1 + x 4 - 1 2 x 2 = x 8 + 2 x 4 + 1 4 x 4 ⇒ 1 + 1 + x 4 - 1 2 x 2 2 = 1 + x 4 - 1 2 x 2 = x 2 + 1 2 2 x 2
Do x > 0 nên P = x 2 + 1 x 2 . Thay x = 1 2 2 2 + 2 - 2 vào P ta được
P = 1 2 2 2 2 - 2 - 2 2 - 2 + 1 1 2 2 2 - 2 - 2 2 2 = 2 2 2 + 2 - 2 2 2 2 - 2 - 2 2
Đáp án A
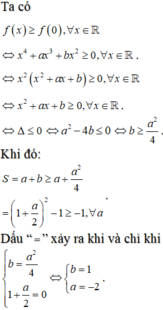
ta có: \(x+\frac{1}{x}=3\)
\(\Rightarrow\left(x+\frac{1}{x}\right)^2=3^2\)
\(\Leftrightarrow x^2+2.x.\frac{1}{x}+\left(\frac{1}{x}\right)^2=9\)
\(\Leftrightarrow x^2+2+\frac{1}{x^2}=9\)
\(\Leftrightarrow x^2+\frac{1}{x^2}=7\)
\(\Rightarrow\left(x^2+\frac{1}{x^2}\right)^2=7^2\)
\(\Leftrightarrow\left(x^2\right)^2+2.x^2.\left(\frac{1}{x^2}\right)+\left(\frac{1}{x^2}\right)^2=49\)
\(\Leftrightarrow x^4+2+\frac{1}{x^4}=49\)
\(\Leftrightarrow x^4+\frac{1}{x^4}=47\)
\(x+\frac{1}{x}=3\Rightarrow\left(x+\frac{1}{x}\right)^2=x^2+2+\frac{1}{x^2}=9\Rightarrow x^2+\frac{1}{x^2}=7\)
\(\Rightarrow\left(x^2+\frac{1}{x^2}\right)^2=x^4+2+\frac{1}{x^4}=49\Rightarrow x^4+\frac{1}{x^4}=47\)