Một ô tô đi từ A với tốc độ trung bình 45 km/h hết 10 phút, nghỉ chân tại điểm B 10 phút ở chính giữa đoạn đường sau đó lại tiếp tục đi quãng đường còn lại hết 15 phút với tốc độ trung bình 36 km/h.
a. Vẽ đồ thị quãng đường – thời gian.
b. Nhìn đồ thị cho biết quãng đường đi được dài bao nhiêu km?


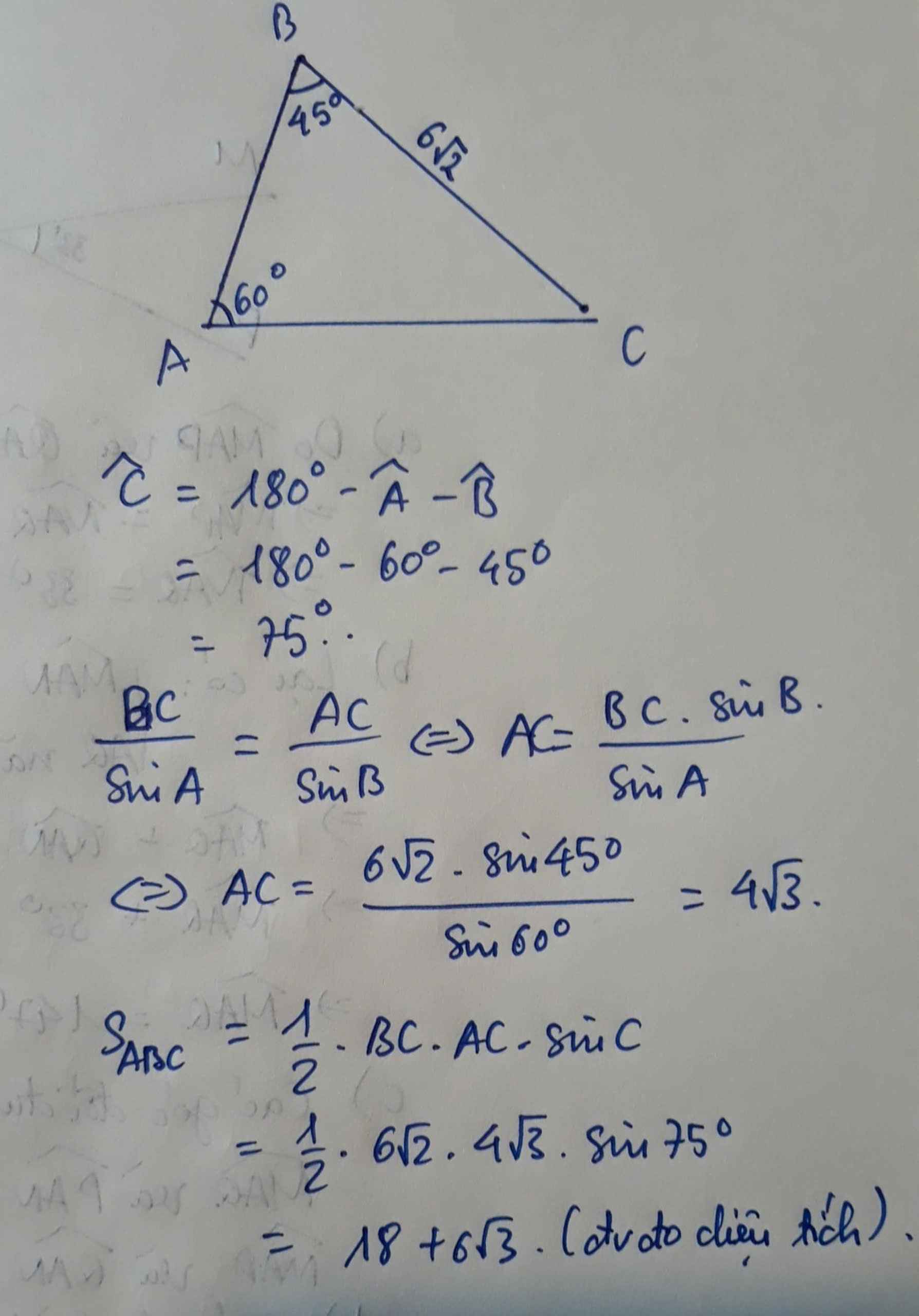
lẹ mn ơiii