Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Ta có: \(\left(x-1\right)^2\ge0\) \(\Leftrightarrow x^2-2x+1\ge0\)\(\Leftrightarrow x^2+1\ge2x\).\(\left(1\right)\)
\(\left(y-2\right)^2\ge0\Leftrightarrow y^2-4y+4\ge0\Leftrightarrow x^2+4\ge4y\).\(\left(2\right)\)
\(\left(z^2-9\right)\ge0\Leftrightarrow z^2-6z+9\ge0\Leftrightarrow z^2+9\ge6z\).\(\left(3\right)\)
Từ \(\left(1\right),\left(2\right)\)và \(\left(3\right)\) nhân vế theo vế ta được:
\(\left(x^2+1\right).\left(y^2+4\right).\left(z^2+9\right)\ge48xyz\)
mà theo đề ta có:\(\left(x^2+1\right).\left(y^2+4\right).\left(z^2+9\right)=48xyz\)
nên \(\left\{{}\begin{matrix}x^2+1=2x\\y^2+4=4y\\z^2+9=6z\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=2\\z=3\end{matrix}\right.\)
Thay \(x=1;y=2;z=3\)vào biểu thức A ta được:
\(A=\dfrac{x^3+y^3+z^3}{\left(x+y+z\right)^2}=\dfrac{1+8+27}{\left(1+2+3\right)^2}=1\)
Vậy giá trị của biểu thức \(A=\dfrac{x^3+y^3+z^3}{\left(x+y+z\right)^2}\)là 1.

Hình bạn tự vẽ nhé!!!
Ta có: \(\widehat{ACB}=180^o-\widehat{ACD}=180^o-100^o=80^o\\ \)
Xét tam giác ADC ta có: \(\widehat{DAC}+\widehat{ACD}+\widehat{ADC}=180^o\)
\(\Leftrightarrow y^o+100^o+x^o=180^o\)
\(\Leftrightarrow x^o+y^o=180^o-100^o=80^o\left(1\right)\)
Xét tam giác ABC ta có:\(\widehat{BAC}+\widehat{ABD}+\widehat{ADB}=180^o\)
\(\Leftrightarrow2y^o+2x^o+x^o=180^o\)
\(\Leftrightarrow2y^o+3x^o=180^o\left(2\right)\)
Thế (1) vào (2) ta được: \(2.\left(80-x^o\right)+3x^o=180^o\)
\(\Leftrightarrow160^o-2x^o+3x^o=180^o\)
\(\Leftrightarrow160^o+x^o=180^o\)
\(\Leftrightarrow x^o=180^o-160^o=20^o\)
Khi đó giá trị của \(x=20\)
Chúc bạn học tốt![]()

a, Theo bài ra ta có:
\(=x^3-x-2x+2\)
\(=x\left(x^2-1\right)-2\left(x-1\right)\)
\(=x\left(x+1\right)\left(x-1\right)-2\left(x-1\right)\)
\(=\left(x-1\right)\left(x^2+x-2\right)\)
b, theo bài ra ta có:
\(=x^3-3x^2-\left(2x^2-6x\right)-\left(3x-9\right)\)
\(=x^2\left(x-3\right)-2x\left(x-3\right)-3\left(x-3\right)\)
\(=\left(x^2-2x-3\right)\left(x-3\right)\)
c,Theo bài ra ta có:
\(=x^3+5x^2+3x^2+15x+2x+10\)
\(=x^2\left(x+5\right)+3x\left(x+5\right)+2\left(x+5\right)\)
\(=\left(x+5\right)\left(x^2+3x+2\right)\)
\(=\left(x+5\right)\left(x^2+x+2x+2\right)=\left(x+5\right)\left(x\left(x+1\right)+2\left(x+1\right)\right)\)
\(=\left(x+5\right)\left(x+1\right)\left(x+2\right)\)
CHÚC BẠN HỌC TỐT...........
a) \(x^3-3x+2\)
= \(x^3-x^2+x^2-x-2x+2\)
= \(x^2\left(x-1\right)+x\left(x-1\right)-2\left(x-1\right)\)
= \(\left(x-1\right)\left(x^2+x-2\right)\)
= \(\left(x-1\right)\left(x^2+2x-x-2\right)\)
= \(\left(x-1\right)\left[x\left(x+2\right)-\left(x+2\right)\right]\)
= \(\left(x-1\right)\left(x+2\right)\left(x-1\right)\)
= \(\left(x-1\right)^2\left(x+2\right)\)
b) \(x^3-5x^2+3x+9\)
= \(x^3+x^2-6x^2-6x+9x+9\)
= \(x^2\left(x+1\right)-6x\left(x+1\right)+9\left(x+1\right)\)
= \(\left(x+1\right)\left(x^2-6x+9\right)\)
= \(\left(x+1\right)\left(x-3\right)^2\)
c) \(x^3+8x^2+17x+10\)
= \(x^3+x^2+7x^2+7x+10x+10\)
= \(x^2\left(x+1\right)+7x\left(x+1\right)+10\left(x+1\right)\)
= \(\left(x+1\right)\left(x^2+7x+10\right)\)
= \(\left(x+1\right)\left(x^2+2x+5x+10\right)\)
= \(\left(x+1\right)\left[x\left(x+2\right)+5\left(x+2\right)\right]\)
= \(\left(x+1\right)\left(x+2\right)\left(x+5\right)\)
d) \(x^3-3x^2+6x+4\)
Câu này đúng là sai đề rồi, mình sửa + làm bên dưới:
\(x^3+3x^2+6x+4\)
= \(x^3+x^2+2x^2+2x+4x+4\)
= \(x^2\left(x+1\right)+2x\left(x+1\right)+4\left(x+1\right)\)
= \(\left(x+1\right)\left(x^2+2x+4\right)\)
Học tốt nhé :))

\(a,\left(x+1\right)^2-\left(x-1\right)^2-3\left(x+1\right)\left(x-1\right)\)
\(=x^2+2x+1-\left(x^2-2x+1\right)-3\left(x^2-1\right)\)
\(=x^2+2x+1-x^2+2x-1-3x^2+2=-3x^2+4x+2\)\(b,5\left(x+2\right)\left(x-2\right)-\left(2x-3\right)^2-x^2+17\)
\(=5\left(x^2-4\right)-\left(4x^2-12x+9\right)-x^2+17\)
\(=5x^2-20-4x^2+12x-9-x^2+17=12x-12\)

\(a,2x^2+8x+5\)
\(=\left(\sqrt{2}x\right)^2+2.\sqrt{2}x.\dfrac{8}{2\sqrt{2}}+\left(\dfrac{8}{2\sqrt{2}}\right)^2-\left(\dfrac{8}{2\sqrt{2}}\right)^2+5\)
\(=\left[\left(\sqrt{2}x\right)^2+2.\sqrt{2}x.\dfrac{8}{2\sqrt{2}}+\left(\dfrac{8}{2\sqrt{2}}\right)^2\right]-\left(\dfrac{8}{2\sqrt{2}}\right)^2+5\)
\(=\left(\sqrt{2}x+\dfrac{8}{2\sqrt{2}}\right)^2-3\)
Ta có :
\(\left(\sqrt{2}x+\dfrac{8}{2\sqrt{2}}\right)^2\ge0\forall x\)
\(\Rightarrow\left(\sqrt{2}x+\dfrac{8}{2\sqrt{2}}\right)^2-3\ge-3>0\)
Dấu = xảy ra khi \(\sqrt{2}x+\dfrac{8}{2\sqrt{2}}=0\Rightarrow x=-2\)
Các câu còn lại dễ rồi mk ko lm nx nha bn ,bn ko bt lm cỗ nào thì hỏi mk
\(z^4-4z^3+z^2+4z^2-4z+1\)
\(=z^4-4z^3+z^2+4z^2-4z+1\)
\(=\left(z^4-4z^3+z^2\right)+\left(4z^2-4z+1\right)\)
\(=z^2\left(z^2-4z+1\right)+\left(4z^2-4z+1\right)\)
\(=z^2\left(z^2-4z+1\right)+\left[\left(2z\right)^2-2.2z.1+1^2\right]\)
\(=z^2\left(z-1\right)^2+\left(2z-1\right)^2\)
Ta có :
\(z^2\left(z-1\right)^2\ge0;\left(2z-1\right)^2\ge0\)
\(\Rightarrow z^2\left(z-1\right)^2+\left(2z-1\right)^2\ge0\) Dấu = xảy ra khi \(\left\{{}\begin{matrix}z-1=0\\2z-1=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}z=1\\z=\dfrac{1}{2}\end{matrix}\right.\)
\(A=28x^2-20x+1\)
\(A=\left(\sqrt{28}x-\dfrac{5\sqrt{7}}{7}\right)^2-\dfrac{18}{7}\)
\(A\ge\dfrac{-18}{7}\)(dấu "=" xảy ra\(\Leftrightarrow x=\dfrac{5}{14}\))
Ta có: \(A=3x^2-8x+6x^2-2x+13x^2-8x+6x^2-2x+1\)
\(\Leftrightarrow A=28x^2-20x+1\)
\(\Leftrightarrow A=28x^2-28\cdot2\cdot\dfrac{5}{14}x+28\cdot\left(\dfrac{5}{14}\right)^2-28\cdot\left(\dfrac{5}{14}\right)^2+1\)
\(\Leftrightarrow A=28\left(x^2-2\cdot\dfrac{5}{14}+\dfrac{5}{14}^2\right)-\dfrac{18}{7}\)
\(\Leftrightarrow A=28\left(x-\dfrac{5}{14}\right)^2-\dfrac{18}{7}\ge-\dfrac{18}{7}\)
Vậy GTNN của A là: \(-\dfrac{18}{7}\)
Dấu "=" xảy ra khi: \(28\left(x-\dfrac{5}{14}\right)^2=0\Leftrightarrow x=\dfrac{5}{14}\)

\(A=7+7^2+7^3+..........+7^{4n}\)
\(\Leftrightarrow A=\left(7+7^2+7^3+7^4\right)+..........+\left(7^{4n-3}+7^{4n-2}+7^{4n-1}+7^{4n}\right)\)
\(\Leftrightarrow A=7\left(1+7+7^2+7^3\right)+.........+7^{4n-3}\left(1+7+7^2+7^3\right)\)
\(\Leftrightarrow A=7.400+7^5.400+..........+7^{4n-3}.400\)
\(\Leftrightarrow A=400\left(7+7^5+........+7^{4n-3}\right)⋮400\)
\(\Leftrightarrow A⋮400\rightarrowđpcm\)
\(A=7^1+7^2+7^3+7^4+7^{4k}\)
=\(7\left(1+7^1+7^2+7^3\right)+...+7^{4k-3}\left(1+7^1+7^2+7^3\right)\)
=\(400\left(7+...+7^{4k-3}\right)⋮400\)
Do đó:\(A⋮400\left(đpcm\right)\)

a, Xét tam giác ABC:
AD= DB
DE// BC
=> AE= EC ( tính chất đg TB)
=> AE= EC = \(\dfrac{1}{2}\)AC= \(\dfrac{1}{2}\).8= 4 cm.
b,Xét tam giác ABC : ^B= 90o
AC2= AB2 + BC2 ( Định lý Pitago)
152= 92 + BC2
=> BC2= 152 - 92 = 144
BC = 12 cm
Theo tính chất đg TB, ta có: DE// BC
=> DE= \(\dfrac{1}{2}\)BC = \(\dfrac{1}{2}\).12 = 6cm
Chúc bạn học tốt !!![]()
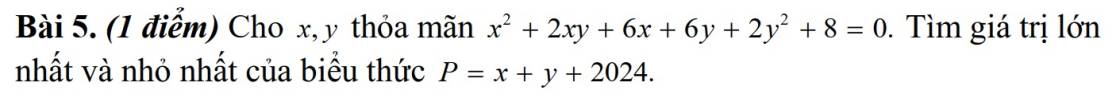 cứu t mn, nhanh nha sáng mai tui đi học rùi chậm nhất là 12h
cứu t mn, nhanh nha sáng mai tui đi học rùi chậm nhất là 12h

Lời giải:
$x^2+2xy+6x+6y+2y^2+8=0$
$\Leftrightarrow (x^2+2xy+y^2)+6x+6y+y^2+8=0$
$\Leftrightarrow (x+y)^2+6(x+y)+y^2+8=0$
$\Leftrightarrow (x+y+3)^2+y^2-1=0$
$\Leftrightarrow (x+y+3)^2=1-y^2\leq 1$
$\Rightarrow -1\leq x+y+3\leq 1$
$\Rightarrow -4\leq x+y\leq -2$
$\Rightarrow 2020\leq x+y+2024\leq 2022$
Vậy $P_{\min}=2020; P_{\max}=2022$