Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(a,2x\left(x-3\right)+5\left(x-3\right)=0\)
\(\Leftrightarrow\left(2x+5\right)\left(x-3\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}2x+5=0\\x-3=0\end{cases}}\Leftrightarrow\orbr{\begin{cases}2x=-5\\x=3\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=-\frac{5}{2}\\x=3\end{cases}}\)
Vậy .........
\(b,\left(x^2-4\right)+\left(x-2\right)\left(3-2x=0\right)\)
\(\Leftrightarrow x^2-4-2x^2+7x-6=0\)
\(\Leftrightarrow-x^2+7x-10=0\)
\(\Leftrightarrow-\left(x-5\right)\left(x-2\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x=5\\x=2\end{cases}}\)
Vậy ..................
\(c,x^3-3x^2+3x-1=0\)
\(\Leftrightarrow\left(x-1\right)^3=0\)
\(\Leftrightarrow x=1\)
\(d,x\left(2x-7\right)-4x+14=0\)
\(\Leftrightarrow2x^2-7x-4x+14=0\)
\(\Leftrightarrow2x^2-11x+14=0\)
\(\Leftrightarrow\left(2x-7\right)\left(x-2\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x=\frac{7}{2}\\x=2\end{cases}}\)
Vậy ............
\(e,\left(2x-5\right)^2-\left(x+2\right)^2=0\)
\(\Leftrightarrow4x^2-20x+25-x^2-4x-4=0\)
\(\Leftrightarrow3x^2-24x+21=0\)
\(\Leftrightarrow3\left(x-7\right)\left(x-1\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x-7=0\\x-1=0\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=7\\x=1\end{cases}}\)
Vậy .....................
\(f,x^2-x-\left(3x-3\right)=0\)
\(\Leftrightarrow x^2-x-3x+3=0\)
\(\Leftrightarrow x^2-4x+3=0\)
\(\Leftrightarrow\left(x-3\right)\left(x-1\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x-3=0\\x-1=0\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=3\\x=1\end{cases}}\)
Vậy ..............

giải phương trình:
- Nếu \(x\ge1\)phương trình trở thành : \(x^2-3x+2=x-1\Leftrightarrow x^2-4x+3=0\Leftrightarrow\orbr{\begin{cases}x=3\\x=1\end{cases}TM}\)
- Nếu \(x< 1\)\(\Rightarrow x^2-3x+2=1-x\Leftrightarrow x^2-2x+1=0\Leftrightarrow\left(x-1\right)^2=0\Leftrightarrow x=1L\)VẬY NGHIỆM PHƯƠNG TRÌNH LÀ : x=1 hoặc x=3
\(x^4+2008x^2+2007x+2008\)
\(=x\left[x\left(x^2+2008\right)+2007\right]+2008\)
\(=\left[\left(x-1\right)x+2008\right]\left(x^2+x+1\right)\)
\(=\left(x^2-x+2008\right)\left(x^2+x+1\right)\)
~(‾▿‾~)

A/ \(16x-5x^2-3=\left(15x-3\right)-\left(5x^2-x\right)=3\left(5x-1\right)-x\left(5x-1\right)=\left(5x-1\right)\left(3-x\right)\)
B/ \(x^3-3x^2+1-3x=\left(x^3-4x^2+x\right)+\left(x^2-4x+1\right)=x\left(x^2-4x+1\right)+\left(x^2-4x+1\right)\)
\(=\left(x+1\right)\left(x^2-4x+1\right)\)
C/ \(x^3-3x^2-4x+12=x^2\left(x-3\right)-4\left(x-3\right)=\left(x-3\right)\left(x-2\right)\left(x+2\right)\)
D/ \(\left(2x+1\right)^2-\left(x-1\right)^2=\left(2x+1-x+1\right)\left(2x+1+x-1\right)=3x\left(x+2\right)\)

bài 1:
a) ĐKXĐ: x khác 0; x khác -1
\(\frac{x-1}{x}+\frac{1-2x}{x^2+x}=\frac{1}{x+1}\)
<=> \(\frac{x-1}{x}+\frac{1-2x}{x\left(x+1\right)}=\frac{1}{x+1}\)
<=> (x - 1)(x + 1) + 1 - 2x = x
<=> x^2 - 2x = x
<=> x^2 - 2x - x = 0
<=> x^2 - 3x = 0
<=> x(x - 3) = 0
<=> x = 0 hoặc x - 3 = 0
<=> x = 0 hoặc x = 0 + 3
<=> x = 0 (ktm) hoặc x = 3 (tm)
=> x = 3
b) ĐKXĐ: x khác +-3; x khác -7/2
\(\frac{13}{\left(x-3\right)\left(2x+7\right)}+\frac{1}{2x+7}=\frac{6}{x^2-9}\)
<=> \(\frac{13}{\left(x-3\right)\left(2x+7\right)}+\frac{1}{2x+7}=\frac{6}{\left(x-3\right)\left(x+3\right)}\)
<=> 13(x + 3) + (x - 3)(x + 3) = 6(2x + 7)
<=> 13x + 30 + x^2 = 12x + 42
<=> 13x + 30 + x^2 - 12x - 42 = 0
<=> x - 12 + x^2 = 0
<=> (x - 3)(x + 4) = 0
<=> x - 3 = 0 hoặc x + 4 = 0
<=> x = 0 + 3 hoặc x = 0 - 4
<=> x = 3 (ktm) hoặc x = -4 (tm)
=> x = -4
c) ĐKXĐ: x khác +-1
\(\frac{x}{x-1}-\frac{2x}{\left(x-1\right)\left(x+1\right)}=0\)
<=> x(x + 1) - 2x = 0
<=> x^2 + x - 2x = 0
<=> x^2 - x = 0
<=> x(x - 1) = 0
<=> x = 0 hoặc x - 1 = 0
<=> x = 0 hoặc x = 0 + 1
<=> x = 0 (tm) hoặc x = 1 (ktm)
=> x = 0
d) \(\frac{x^2+2x}{x^2+1}-2x=0\)
<=> \(\frac{x\left(x+2\right)}{x^2+1}-2x=0\)
<=> x(x + 2) - 2x(x^2 + 1) = 0
<=> x^2 - 2x^3 = 0
<=> x^2(1 - 2x) = 0
<=> x^2 = 0 hoặc 1 - 2x = 0
<=> x = 0 hoặc -2x = 0 - 1
<=> x = 0 hoặc -2x = -1
<=> x = 0 hoặc x = 1/2
bài 2:
(x - 1)(x^2 + 3x - 2) - (x^3 - 1) = 0
<=> x^3 + 3x^2 - 2x - x^2 - 3x + 2 - x^2 + 1 = 0
<=> 2x^2 - 2x - 3x + 3 = 0
<=> 2x(x - 1) - 3(x - 1) = 0
<=> (2x - 3)(x - 1) = 0
<=> 2x - 3 = 0 hoặc x - 1 = 0
<=> 2x = 0 + 3 hoặc x = 0 + 1
<=> 2x = 3 hoặc x = 1
<=> x = 3/2 hoặc x = 1
bài 3:
(x^3 + x^2) + (x^2 + x) = 0
<=> x^3 + x^2 + x^2 + x = 0
<=> x^3 + 2x^2 + x = 0
<=> x(x^2 + 2x + 1) = 0
<=> x(x + 1)^2 = 0
<=> x = 0 hoặc x + 1 = 0
<=> x = 0 hoặc x = 0 - 1
<=> x = 0 hoặc x = -1


\(3y^3+6xy^2+3x^2y=3y\left(y^2+2xy+x^2\right)=3y\left(x+y\right)^2\)
\(x^3-3x^2-4x+12=x^2\left(x-3\right)-4\left(x-3\right)=\left(x-3\right)\left(x^2-4\right)=\left(x-3\right)\left(x-2\right)\left(x+2\right)\)
\(x^3+3x^2-3x-1=\left(x-1\right)\left(x^2+x+1\right)+3x\left(x-1\right)=\left(x-1\right)\left(x^2+x+1+3x\right)\)
\(=\left(x-1\right)\left(x^2+4x+1\right)\)
Tham khảo nhé~

Bài giải:
a) x2 – 3x + 2 = a) x2 – x - 2x + 2 = x(x - 1) - 2(x - 1) = (x - 1)(x - 2)
Hoặc x2 – 3x + 2 = x2 – 3x - 4 + 6
= x2 - 4 - 3x + 6
= (x - 2)(x + 2) - 3(x -2)
= (x - 2)(x + 2 - 3) = (x - 2)(x - 1)
b) x2 + x – 6 = x2 + 3x - 2x – 6
= x(x + 3) - 2(x + 3)
= (x + 3)(x - 2).
c) x2 + 5x + 6 = x2 + 2x + 3x + 6
= x(x + 2) + 3(x + 2)
= (x + 2)(x + 3)

a) = (x + 1)^3 - 27z^3 = (x+1 - 3z)( (x+1)^2 + 3z(x+1) + 9z^2 )
b)= x^2 + x+ 3x + 3 = x (x+1) +3 (x+1) =(x+3)(x+1)
c) = 2x^2 - 2x + 5x - 5 = 2x(x-1) + 5(x-1) = (2x+5)(x-1)
d) = (a^2 + 1 - 2a)(a^2 +2a +1) = (a-1)^2 * (a+1)^2
e) = x^3 ( x-1) - (x^2 - 1) = x^3 ( x-1) - (x+1)(x-1) = (x^3 -x -1)(x-1)
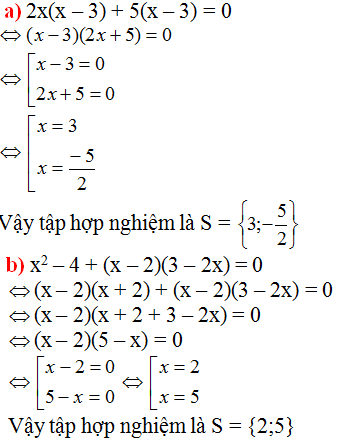
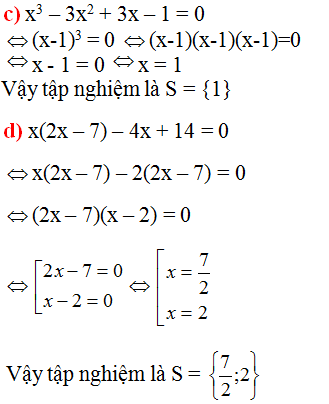
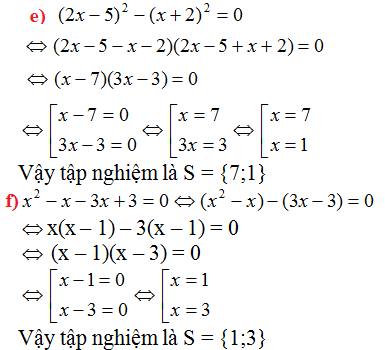
x3 – 3x2 + 3x - 1 = 0
⇔ (x – 1)3 = 0 (Hằng đẳng thức)
⇔ x – 1 = 0
⇔ x = 1.
Vậy tập nghiệm của phương trình là S={1}.