K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
TL
0
AH
Akai Haruma
Giáo viên
12 tháng 11 2017
Câu 1:
Để ý rằng \((2-\sqrt{3})(2+\sqrt{3})=1\) nên nếu đặt
\(\sqrt{2+\sqrt{3}}=a\Rightarrow \sqrt{2-\sqrt{3}}=\frac{1}{a}\)
PT đã cho tương đương với:
\(ma^x+\frac{1}{a^x}=4\)
\(\Leftrightarrow ma^{2x}-4a^x+1=0\) (*)
Để pt có hai nghiệm phân biệt \(x_1,x_2\) thì pt trên phải có dạng pt bậc 2, tức m khác 0
\(\Delta'=4-m>0\Leftrightarrow m< 4\)
Áp dụng hệ thức Viete, với $x_1,x_2$ là hai nghiệm của pt (*)
\(\left\{\begin{matrix} a^{x_1}+a^{x_2}=\frac{4}{m}\\ a^{x_1}.a^{x_2}=\frac{1}{m}\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} a^{x_2}(a^{x_1-x_2}+1)=\frac{4}{m}\\ a^{x_1+x_2}=\frac{1}{m}(1)\end{matrix}\right.\)
Thay \(x_1-x_2=\log_{2+\sqrt{3}}3=\log_{a^2}3\) :
\(\Rightarrow a^{x_2}(a^{\log_{a^2}3}+1)=\frac{4}{m}\)
\(\Leftrightarrow a^{x_2}(\sqrt{3}+1)=\frac{4}{m}\Rightarrow a^{x_2}=\frac{4}{m(\sqrt{3}+1)}\) (2)
\(a^{x_1}=a^{\log_{a^2}3+x_2}=a^{x_2}.a^{\log_{a^2}3}=a^{x_2}.\sqrt{3}\)
\(\Rightarrow a^{x_1}=\frac{4\sqrt{3}}{m(\sqrt{3}+1)}\) (3)
Từ \((1),(2),(3)\Rightarrow \frac{4}{m(\sqrt{3}+1)}.\frac{4\sqrt{3}}{m(\sqrt{3}+1)}=\frac{1}{m}\)
\(\Leftrightarrow \frac{16\sqrt{3}}{m^2(\sqrt{3}+1)^2}=\frac{1}{m}\)
\(\Leftrightarrow m=\frac{16\sqrt{3}}{(\sqrt{3}+1)^2}=-24+16\sqrt{3}\) (thỏa mãn)
AH
Akai Haruma
Giáo viên
12 tháng 11 2017
Câu 2:
Nếu \(1> x>0\)
\(2017^{x^3}>2017^0\Leftrightarrow 2017^{x^3}>1\)
\(0< x< 1\Rightarrow \frac{1}{x^5}>1\)
\(\Rightarrow 2017^{\frac{1}{x^5}}> 2017^1\Leftrightarrow 2017^{\frac{1}{x^5}}>2017\)
\(\Rightarrow 2017^{x^3}+2017^{\frac{1}{x^5}}> 1+2017=2018\) (đpcm)
Nếu \(x>1\)
\(2017^{x^3}> 2017^{1}\Leftrightarrow 2017^{x^3}>2017 \)
\(\frac{1}{x^5}>0\Rightarrow 2017^{\frac{1}{x^5}}>2017^0\Leftrightarrow 2017^{\frac{1}{5}}>1\)
\(\Rightarrow 2017^{x^3}+2017^{\frac{1}{x^5}}>2018\) (đpcm)
NV
Nguyễn Việt Lâm
Giáo viên
15 tháng 2 2019
\(I=\int\dfrac{x^3dx}{\left(x^8-4\right)^2}\)
Đặt \(x^4=t\Rightarrow x^3dx=\dfrac{1}{4}dt\Rightarrow I=\dfrac{1}{4}\int\dfrac{dt}{\left(t^2-2\right)^2}=\dfrac{1}{4}\int\dfrac{dt}{\left(t-\sqrt{2}\right)^2\left(t+\sqrt{2}\right)^2}\)
\(=\dfrac{1}{32}\int\left(\dfrac{1}{t-\sqrt{2}}-\dfrac{1}{t+\sqrt{2}}\right)^2dt=\dfrac{1}{32}\int\left(\dfrac{1}{\left(t-\sqrt{2}\right)^2}+\dfrac{1}{\left(t+\sqrt{2}\right)^2}-\dfrac{2}{\left(t+\sqrt{2}\right)\left(t-\sqrt{2}\right)}\right)dt\)
\(=\dfrac{1}{32}\int\left(\dfrac{1}{\left(t-\sqrt{2}\right)^2}+\dfrac{1}{\left(t+\sqrt{2}\right)^2}-\dfrac{1}{\sqrt{2}}\left(\dfrac{1}{t-\sqrt{2}}-\dfrac{1}{t+\sqrt{2}}\right)\right)dt\)
\(=\dfrac{1}{32}\left(\dfrac{-1}{t-\sqrt{2}}-\dfrac{1}{t+\sqrt{2}}-\dfrac{1}{\sqrt{2}}ln\left|\dfrac{t-\sqrt{2}}{t+\sqrt{2}}\right|\right)+C\)
\(=\dfrac{1}{32}\left(\dfrac{-1}{x^4-\sqrt{2}}-\dfrac{1}{x^4+\sqrt{2}}-\dfrac{1}{\sqrt{2}}ln\left|\dfrac{x^4-\sqrt{2}}{x^4+\sqrt{2}}\right|\right)+C\)
2/ \(I=\int\dfrac{\left(2x+1\right)dx}{\left(x^2+x-1\right)\left(x^2+x+3\right)}=\dfrac{1}{4}\int\left(\dfrac{1}{x^2+x-1}-\dfrac{1}{x^2+x+3}\right)\left(2x+1\right)dx\)
\(=\dfrac{1}{4}\int\left(\dfrac{2x+1}{x^2+x-1}-\dfrac{2x+1}{x^2+x+3}\right)dx\)
\(=\dfrac{1}{4}\left(\int\dfrac{d\left(x^2+x-1\right)}{x^2+x-1}-\int\dfrac{d\left(x^2+x+3\right)}{x^2+x+3}\right)\)
\(=\dfrac{1}{4}ln\left|\dfrac{x^2+x-1}{x^2+x+3}\right|+C\)
3/ Đặt \(\sqrt[3]{x}=t\Rightarrow x=t^3\Rightarrow dx=3t^2dt\)
\(\Rightarrow I=\int\dfrac{3t^2.sint.dt}{t^2}=3\int sint.dt=-3cost+C=-3cos\left(\sqrt[3]{x}\right)+C\)
4/ \(I=\int\dfrac{dx}{1+cos^2x}=\int\dfrac{\dfrac{1}{cos^2x}dx}{\dfrac{1}{cos^2x}+1}\)
Đặt \(t=tanx\Rightarrow\left\{{}\begin{matrix}dt=\dfrac{1}{cos^2x}dx\\\dfrac{1}{cos^2x}=1+tan^2x=1+t^2\end{matrix}\right.\)
\(\Rightarrow I=\int\dfrac{dt}{1+t^2+1}=\int\dfrac{dt}{t^2+2}=\dfrac{1}{2}\int\dfrac{dt}{\left(\dfrac{t}{\sqrt{2}}\right)^2+1}\)
\(=\dfrac{1}{2}.\sqrt{2}.arctan\left(\dfrac{t}{\sqrt{2}}\right)+C=\dfrac{1}{\sqrt{2}}arctan\left(\dfrac{tanx}{\sqrt{2}}\right)+C\)
5/ \(I=\int\dfrac{sinx+cosx}{4+2sinx.cosx-sin^2x-cos^2x}dx=\int\dfrac{sinx+cosx}{4-\left(sinx-cosx\right)^2}dx\)
Đặt \(sinx-cosx=t\Rightarrow\left(cosx+sinx\right)dx=dt\)
\(\Rightarrow I=\int\dfrac{dt}{4-t^2}=-\int\dfrac{dt}{\left(t-2\right)\left(t+2\right)}=\dfrac{1}{4}\int\left(\dfrac{1}{t+2}-\dfrac{1}{t-2}\right)dt\)
\(=\dfrac{1}{4}ln\left|\dfrac{t+2}{t-2}\right|+C=\dfrac{1}{4}ln\left|\dfrac{sinx-cosx+2}{sinx-cosx-2}\right|+C\)
NV
Nguyễn Việt Lâm
Giáo viên
15 tháng 2 2019
Ơ bài 1 nhầm số 4 thành số 2 rồi, bạn sửa lại 1 chút nhé :D
Còn 1 cách làm khác nữa là lượng giác hóa
Đặt \(x^4=2sint\Rightarrow x^3dx=\dfrac{1}{2}cost.dt\)
\(\Rightarrow I=\dfrac{1}{2}\int\dfrac{cost.dt}{\left(4sin^2t-4\right)^2}=\dfrac{1}{32}\int\dfrac{cost.dt}{cos^4t}=\dfrac{1}{32}\int\dfrac{dt}{cos^3t}\)
Đặt \(\left\{{}\begin{matrix}u=\dfrac{1}{cost}\\dv=\dfrac{dt}{cos^2t}\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}du=\dfrac{sint.dt}{cos^2t}\\v=tant\end{matrix}\right.\)
\(\Rightarrow32I=\dfrac{tant}{cost}-\int\dfrac{tant.sint.dt}{cos^2t}=\dfrac{sint}{cos^2t}-\int\dfrac{sin^2t.dt}{cos^3t}\)
\(=\dfrac{sint}{1-sin^2t}-\int\dfrac{1-cos^2t}{cos^3t}dt=\dfrac{sint}{1-sin^2t}-\int\dfrac{dt}{cos^3t}+\int\dfrac{1}{cosx}dx\)
Chú ý rằng \(\int\dfrac{dt}{cos^3t}=32I\)
\(\Rightarrow32I=\dfrac{sint}{1-sin^2t}-32I+\int\dfrac{cost.dt}{cos^2t}\)
\(\Rightarrow64I=\dfrac{sint}{1-sin^2t}-\int\dfrac{d\left(sint\right)}{sin^2t-1}=\dfrac{sint}{1-sin^2t}-\dfrac{1}{2}ln\left|\dfrac{sint-1}{sint+1}\right|+C\)
\(\Rightarrow I=\dfrac{1}{64}\left(\dfrac{2x^4}{4-x^8}-\dfrac{1}{2}ln\left|\dfrac{x^4-2}{x^4+2}\right|\right)+C\)
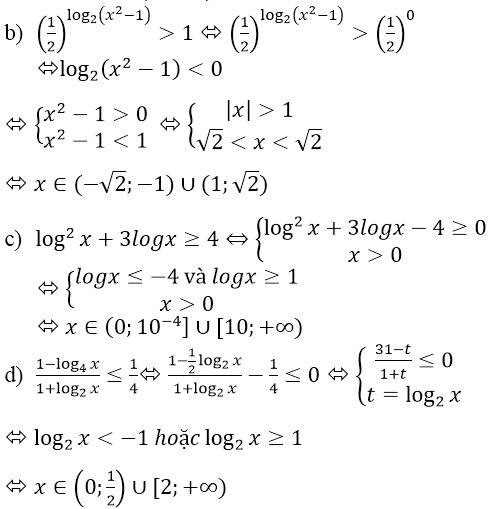
\(\Leftrightarrow1+8^{\dfrac{x}{2}}=9^{\dfrac{x}{2}}\)
\(\Leftrightarrow\left(\dfrac{1}{9}\right)^{\dfrac{x}{2}}+\left(\dfrac{8}{9}\right)^{\dfrac{x}{2}}=1\)
\(\Leftrightarrow\left(\dfrac{1}{9}\right)^{\dfrac{x}{2}}+\left(\dfrac{8}{9}\right)^{\dfrac{x}{2}}-1=0\)
Nhận thấy \(\dfrac{x}{2}=1\Leftrightarrow x=2\) là 1 nghiệm của pt đã cho
Xét hàm \(f\left(x\right)=\left(\dfrac{1}{9}\right)^{\dfrac{x}{2}}+\left(\dfrac{8}{9}\right)^{\dfrac{x}{2}}-1\)
\(f'\left(x\right)=\dfrac{1}{2}.\left(\dfrac{1}{9}\right)^{\dfrac{x}{2}}.ln\left(\dfrac{1}{9}\right)+\dfrac{1}{2}\left(\dfrac{8}{9}\right)^{\dfrac{x}{2}}.ln\left(\dfrac{8}{9}\right)< 0\)
\(\Rightarrow f\left(x\right)\) nghịch biến trên R
\(\Rightarrow f\left(x\right)\) có tối đa 1 nghiệm
\(\Rightarrow x=2\) là nghiệm duy nhất của pt đã cho