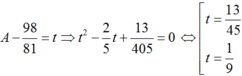Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Bài 1:
\(P=3^x\left(3+3^2+3^3+3^4\right)+...+3^{x+96}\left(3+3^2+3^3+3^4\right)\)
\(=120\left(3^x+...+3^{x+96}\right)⋮120\)


\(A=\left|x+1\right|+5\)
\(\Rightarrow\left|x+1\right|+5\ge5\)
\(\Rightarrow\left|x+1\right|\ge0\)
\(\Rightarrow x+1\ge0\)
\(\Rightarrow x\ge-1\)
Mà A đạt GTNN, suy ra \(\left|x+1\right|\) nhỏ nhất
\(\Rightarrow x=-1\)
Thay \(x=-1\) vào biểu thức ta có:
\(A=\left|-1+1\right|+5=0+5=5\)
Vậy: \(Min_A=5\)
\(B=\left(x-1\right)^2=\left|y-3\right|+2\)
\(B=a^2-2a1+1^2=\left|y-3\right|+2\)
\(B=a^2-2a1+1=\left|y-3\right|+2\)
\(\Rightarrow a^2-2a1+1+2=\left|y-3\right|\)
\(\Rightarrow a\left(a-2\right)+1+2=\left|y-3\right|\)
\(\Rightarrow a\left(a-2\right)+3=\left|y-3\right|\)
\(\Rightarrow\left[\begin{array}{nghiempt}a\left(a-2\right)+3=y-3\\a\left(a-2\right)+3=-y-3\end{array}\right.\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}a\left(a-2\right)=y-3-3\\a\left(a-2\right)=-y-3-3\end{array}\right.\)
\(\Rightarrow\left[\begin{array}{nghiempt}a\left(a-2\right)=y-6\\a\left(a-2\right)=-y-6\end{array}\right.\)
\(\Rightarrow a^2-2a=-y-6\)
\(\Rightarrow a^2-2a+y=-6\)
\(\Rightarrow a\left(a-2\right)+y=-6\) (loại do âm)
\(a\left(a-2\right)=y-6\)
\(\Rightarrow-y+6=-a\left(a-2\right)\)
\(\Rightarrow6=y-a\left(a-2\right)\) (nhận)
Vậy: \(Min_B=6\)

a)(x2-5x+6)(x2-5x+2)-5
Đặt \(x^2-5x+2=t\) ta được:
\(\left(t+4\right)t-16\)\(=t^2+4t-5\)
\(=t^2+5t-t-5\)
\(=t\left(t+5\right)-\left(t+5\right)\)
\(=\left(t-1\right)\left(t+5\right)\)\(=\left(x^2-5x+2-1\right)\left(x^2-5x+2+5\right)\)
\(=\left(x^2-5x+1\right)\left(x^2-5x+7\right)\)
b) (x2+8x-5)(x2+8x+1)-16
Đặt \(t=x^2+8x-5\) ta đc:
\(t\left(t+6\right)-16\)\(=t^2+6t-16\)
\(=t^2+8t-2t-16\)
\(=t\left(t+8\right)-2\left(t+8\right)\)
\(=\left(t-2\right)\left(t+8\right)\)\(=\left(x^2+8x-5-2\right)\left(x^2+8x-5+8\right)\)
\(=\left(x^2+8x-7\right)\left(x^2+8x+3\right)\)

a) Thay x= 1/2 và y=-1/3 vào biểu thức A, ta được:
A= 3.(1/2)2 .(-1/3)+ 6.(1/2).(-1/3)2+ 3.(1/2).(-1/3)3= -7/8
Vậy giá trị của biểu thức A tại x=1/2 và y=-1/3 là -7/8


a) \(16x^2-\left(4x-5\right)^2=15\) \(\Leftrightarrow\) \(16x^2-\left(16x^2-40x+25\right)=15\)
\(\Leftrightarrow\) \(16x^2-16x^2+40x-25=15\) \(\Leftrightarrow\) \(40x-25=15\)
\(\Leftrightarrow\) \(40x=40\) \(\Leftrightarrow\) \(x=1\) vậy \(x=1\)
b) \(\left(2x+3\right)^2-4\left(x-1\right)\left(x+1\right)=49\)
\(\Leftrightarrow\) \(4x^2+12x+9-4\left(x^2-1\right)=49\)
\(\Leftrightarrow\) \(4x^2+12x+9-4x^2+4=49\)
\(\Leftrightarrow\) \(12x+13=49\) \(\Leftrightarrow\) \(12x=36\) \(\Leftrightarrow\) \(x=\dfrac{36}{12}=3\)vậy \(x=3\)
c) \(\left(2x+1\right)\left(2x-1\right)+\left(1-2x\right)^2=18\)
\(\Leftrightarrow\) \(4x^2-1+1-4x+4x^2=18\)\(\Leftrightarrow\) \(8x^2-4x=18\)
\(\Leftrightarrow\) \(8x^2-4x-18=0\)
\(\Delta'=\left(-2\right)^2-8.\left(-18\right)=4+144=148>0\)
\(\Rightarrow\) phương trình có 2 nghiệm phân biệt
\(x_1=\dfrac{2+\sqrt{148}}{8}=\dfrac{1+\sqrt{37}}{4}\)
\(x_2=\dfrac{2-\sqrt{148}}{8}=\dfrac{1-\sqrt{37}}{4}\)
vậy \(x=\dfrac{1+\sqrt{37}}{4};x=\dfrac{1-\sqrt{37}}{4}\)
Giải:
a) \(16x^2-\left(4x-5\right)^2=15\)
\(\Leftrightarrow16x^2-16x^2-40x+25=15\)
\(\Leftrightarrow-40x+25=15\)
\(\Leftrightarrow-40x=15-25=-10\)
\(\Leftrightarrow x=-\dfrac{10}{-40}=\dfrac{1}{4}\)
Vậy \(x=\dfrac{1}{4}\)
b) \(\left(2x+3\right)^2-4\left(x-1\right)\left(x+1\right)=49\)
\(\Leftrightarrow4x^2+12x+9-4\left(x^2-1^2\right)=49\)
\(\Leftrightarrow4x^2+12x+9-4x^2+4=49\)
\(\Leftrightarrow12x+9+4=49\)
\(\Leftrightarrow12x=49-9-4\)
\(\Leftrightarrow12x=36\)
\(\Leftrightarrow x=\dfrac{36}{12}=3\)
Vậy \(x=3\)
c) \(\left(2x+1\right)\left(2x-1\right)+\left(1-2x\right)^2=18\)
\(\Leftrightarrow4x^2-1+1-4x+4x^2=18\)
\(\Leftrightarrow8x^2-4x=18\)
Mình chỉ làm được đến đây thôi, hình như là đề bị sai bạn nhé!
Chúc bạn học tốt!

B= \(\left[\left(x+\frac{1}{2}\right)^2+\frac{3}{4}\right]^2\)
ta thấy : \(\left(x+\frac{1}{2}\right)^2\ge0\)
=> \(\left(x+\frac{1}{2}\right)^2+\frac{3}{4}\ge\frac{3}{4}\)
=>\(\left[\left(x+\frac{1}{2}\right)^2+\frac{3}{4}\right]^2\ge\frac{9}{16}\)
=> min B=9/16 kh x=-1/2
C= \(x^2-2xy+y^2+1\)= \(\left(x-y\right)^2+1\)
ta có \(\left(x-y\right)^2\ge0\)=>\(\left(x-y\right)^2+1\ge1\)
=> Min C=1 khi x=y

Theo bài ra :
\(\left(x+5\right)\left(x^2-1\right)\left(3-x\right)>0\)
<=> \(\left(x+5\right)\left(x-1\right)\left(x+1\right)\left(3-x\right)>0\)
Đặt \(\left(x+5\right)\left(x-1\right)\left(x+1\right)\left(3-x\right)=A\)
Ta có bảng xét dấu :
| \(-\infty\) | -5 | -1 | 1 | 3 | \(+\infty\) | ||||
| (x+5) | - | 0 | + | + | + | + | |||
| x2-1 | + | + | 0 | - | 0 | + | + | ||
| 3-x | + | + | + | + | 0 | - | |||
| A | - (loại) | 0 (loại) | +(t.m) | 0(loại) | -(loại) | 0(loại) | +(t.m) | 0(loại) | -(loại) |
Từ bảng xét dấu trên suy ra :
\(A>0\Rightarrow\left[{}\begin{matrix}-5< x< -1\\1< x< 3\end{matrix}\right.\)

Chọn D.
Ta có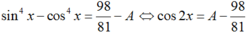
Đặt