TM
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
8 tháng 12 2016
\(dk:x\ne\left\{1,\sqrt{2},4\right\};x\ge0\)dat \(\sqrt{x}=t\)
\(A=\left(\frac{3t^2}{t^2-t-2}+\frac{1}{t-1}+\frac{1}{t-2}\right)\left(t^2-1\right)==\left(\frac{3t^2}{\left(t-2\right)\left(t-1\right)}+\frac{1}{t-1}+\frac{1}{t-2}\right)\left(t^2-1\right)\)
\(=\left(\frac{3t^2}{\left(t-2\right)\left(t-1\right)}+\frac{t-2}{t-1}+\frac{t-1}{t-2}\right)\left(t-1\right)\left(t+1\right)=3t^2+2t-3\)
\(A=3x+2\sqrt{x}-3\)
b
\(\frac{1}{A}=\frac{1}{3x+2\sqrt{x}-3}\Rightarrow\orbr{\begin{cases}3x+2\sqrt{x}-3=-1\\3x+2\sqrt{x}-3=1\end{cases}}\)tư làm tiếp
29 tháng 12 2023
c: P=A:B
\(=\dfrac{\sqrt{x}+2}{\sqrt{x}}:\dfrac{\sqrt{x}-2}{\sqrt{x}}\)
\(=\dfrac{\sqrt{x}+2}{\sqrt{x}-2}\)
=>\(P=\dfrac{\sqrt{x}-2+4}{\sqrt{x}-2}=1+\dfrac{4}{\sqrt{x}-2}\)
Để P lớn nhất thì \(\dfrac{4}{\sqrt{x}-2}\) lớn nhất
=>\(\sqrt{x}-2=1\)
=>\(\sqrt{x}=3\)
=>x=9(nhận)
11 tháng 1 2023
a: Khi x=64 thì \(A=\dfrac{3\cdot8+1}{8+2}=\dfrac{25}{10}=\dfrac{5}{2}\)
b: \(B=\dfrac{2\sqrt{x}-4-\sqrt{x}+5}{x-4}\cdot\dfrac{\sqrt{x}-2}{\sqrt{x}+1}=\dfrac{1}{\sqrt{x}+2}\)
HC
0
15 tháng 6 2023
`1/P=(sqrtx+1)/(sqrtx-3)=(sqrtx-3+4)/(sqrtx-3)=1+4/(sqrtx-3)(x>=0,x\ne9)`
Để `1/P` max thì `4/(sqrtx-3)` max
Nhận thấy nếu `x<9` thì `sqrtx-3<0` hay `4/(sqrtx-3)<0`
Nếu `x>9` thì `4/(sqrtx-3)>0`
Do đó ta xét `x>9` hay `x>=10`
`=>sqrtx-3>=sqrt10-3`
`=>4/(sqrtx-3)<=4/(sqrt10-3)`
Hay `(1/P)_(max)=1+4/(sqrt10-3)<=>x=10`
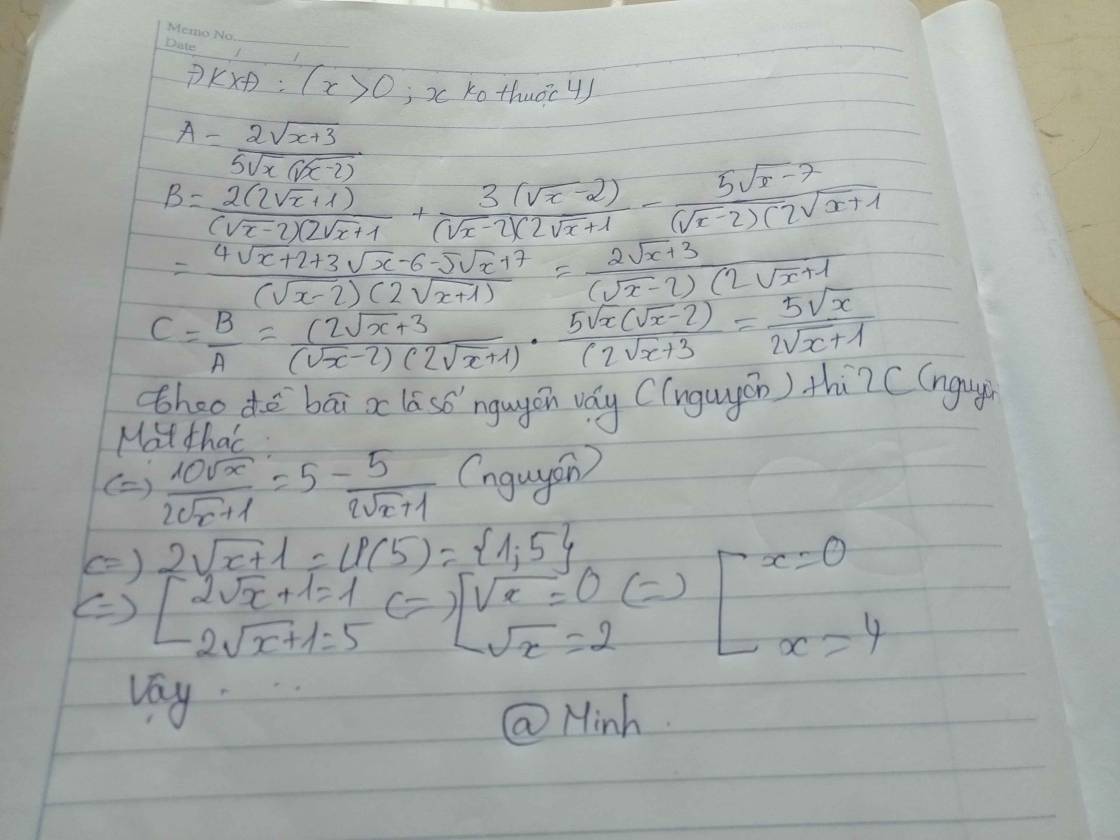
a) \(3x4^{x+1}-5.4^x=448\)
\(\Leftrightarrow4^x\left(3x4-5\right)=448\)
\(\Leftrightarrow4^x.7=448\)
\(\Leftrightarrow4^x=64=4^3\)
\(\Leftrightarrow x=3\)
b) \(5.\left(x-2\right)^3=4^3+71\left(x>1\right)\)
\(\Leftrightarrow5.\left(x-2\right)^3=64+71\)
\(\Leftrightarrow5.\left(x-2\right)^3=135\)
\(\Leftrightarrow\left(x-2\right)^3=27=3^3\)
\(\Leftrightarrow x-2=3\)
\(\Leftrightarrow x=5\)