Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

`a)TXĐ:R\\{1;1/3}`
`y'=[-4(6x-4)]/[(3x^2-4x+1)^5]`
`b)TXĐ:R`
`y'=2x. 3^[x^2-1] ln 3-e^[-x+1]`
`c)TXĐ: (4;+oo)`
`y'=[2x-4]/[x^2-4x]+2/[(2x-1).ln 3]`
`d)TXĐ:(0;+oo)`
`y'=ln x+2/[(x+1)^2].2^[[x-1]/[x+1]].ln 2`
`e)TXĐ:(-oo;-1)uu(1;+oo)`
`y'=-7x^[-8]-[2x]/[x^2-1]`
Lời giải:
a.
$y'=-4(3x^2-4x+1)^{-5}(3x^2-4x+1)'$
$=-4(3x^2-4x+1)^{-5}(6x-4)$
$=-8(3x-2)(3x^2-4x+1)^{-5}$
b.
$y'=(3^{x^2-1})'+(e^{-x+1})'$
$=(x^2-1)'3^{x^2-1}\ln 3 + (-x+1)'e^{-x+1}$
$=2x.3^{x^2-1}.\ln 3 -e^{-x+1}$
c.
$y'=\frac{(x^2-4x)'}{x^2-4x}+\frac{(2x-1)'}{(2x-1)\ln 3}$
$=\frac{2x-4}{x^2-4x}+\frac{2}{(2x-1)\ln 3}$
d.
\(y'=(x\ln x)'+(2^{\frac{x-1}{x+1}})'=x(\ln x)'+x'\ln x+(\frac{x-1}{x+1})'.2^{\frac{x-1}{x+1}}\ln 2\)
\(=x.\frac{1}{x}+\ln x+\frac{2}{(x+1)^2}.2^{\frac{x-1}{x+1}}\ln 2\\ =1+\ln x+\frac{2^{\frac{2x}{x+1}}\ln 2}{(x+1)^2}\)
e.
\(y'=-7x^{-8}-\frac{(x^2-1)'}{x^2-1}=-7x^{-8}-\frac{2x}{x^2-1}\)

a: \(y=\left(2x^2-x+1\right)^{\dfrac{1}{3}}\)
=>\(y'=\dfrac{1}{3}\left(2x^2-x+1\right)^{\dfrac{1}{3}-1}\cdot\left(2x^2-x+1\right)'\)
\(=\dfrac{1}{3}\cdot\left(4x-1\right)\left(2x^2-x+1\right)^{-\dfrac{2}{3}}\)
b: \(y=\left(3x+1\right)^{\Omega}\)
=>\(y'=\Omega\cdot\left(3x+1\right)'\cdot\left(3x+1\right)^{\Omega-1}\)
=>\(y'=3\Omega\left(3x+1\right)^{\Omega-1}\)
c: \(y=\sqrt[3]{\dfrac{1}{x-1}}\)
=>\(y'=\dfrac{\left(\dfrac{1}{x-1}\right)'}{3\cdot\sqrt[3]{\left(\dfrac{1}{x-1}\right)^2}}\)
\(=\dfrac{\dfrac{1'\left(x-1\right)-\left(x-1\right)'\cdot1}{\left(x-1\right)^2}}{\dfrac{3}{\sqrt[3]{\left(x-1\right)^2}}}\)
\(=\dfrac{-x}{\left(x-1\right)^2}\cdot\dfrac{\sqrt[3]{\left(x-1\right)^2}}{3}\)
\(=\dfrac{-x}{\sqrt[3]{\left(x-1\right)^4}\cdot3}\)
d: \(y=log_3\left(\dfrac{x+1}{x-1}\right)\)
\(\Leftrightarrow y'=\dfrac{\left(\dfrac{x+1}{x-1}\right)'}{\dfrac{x+1}{x-1}\cdot ln3}\)
\(\Leftrightarrow y'=\dfrac{\left(x+1\right)'\left(x-1\right)-\left(x+1\right)\left(x-1\right)'}{\left(x-1\right)^2}:\dfrac{ln3\left(x+1\right)}{x-1}\)
\(\Leftrightarrow y'=\dfrac{x-1-x-1}{\left(x-1\right)^2}\cdot\dfrac{x-1}{ln3\cdot\left(x+1\right)}\)
\(\Leftrightarrow y'=\dfrac{-2}{\left(x-1\right)\cdot\left(x+1\right)\cdot ln3}\)
e: \(y=3^{x^2}\)
=>\(y'=\left(x^2\right)'\cdot ln3\cdot3^{x^2}=2x\cdot ln3\cdot3^{x^2}\)
f: \(y=\left(\dfrac{1}{2}\right)^{x^2-1}\)
=>\(y'=\left(x^2-1\right)'\cdot ln\left(\dfrac{1}{2}\right)\cdot\left(\dfrac{1}{2}\right)^{x^2-1}=2x\cdot ln\left(\dfrac{1}{2}\right)\cdot\left(\dfrac{1}{2}\right)^{x^2-1}\)
h: \(y=\left(x+1\right)\cdot e^{cosx}\)
=>\(y'=\left(x+1\right)'\cdot e^{cosx}+\left(x+1\right)\cdot\left(e^{cosx}\right)'\)
=>\(y'=e^{cosx}+\left(x+1\right)\cdot\left(cosx\right)'\cdot e^u\)
\(=e^{cosx}+\left(x+1\right)\cdot\left(-sinx\right)\cdot e^u\)
a) \(y=\left(2x^2-x+1\right)^{\dfrac{1}{3}}\)
\(\Rightarrow y'=\dfrac{1}{3}.\left(2x^2-x+1\right)^{\dfrac{1}{3}-1}.\left(4x-1\right)\)
\(\Rightarrow y'=\dfrac{1}{3}.\left(2x^2-x+1\right)^{-\dfrac{2}{3}}.\left(4x-1\right)\)
b) \(y=\left(3x+1\right)^{\pi}\)
\(\Rightarrow y'=\pi.\left(3x+1\right)^{\pi-1}.3=3\pi.\left(3x+1\right)^{\pi-1}\)
c) \(y=\sqrt[3]{\dfrac{1}{x-1}}\)
\(\Rightarrow y'=\dfrac{\left(x-1\right)^{-1-1}}{3\sqrt[3]{\left(\dfrac{1}{x-1}\right)^{3-1}}}=\dfrac{\left(x-1\right)^{-2}}{3\sqrt[3]{\left(\dfrac{1}{x-1}\right)^2}}=\dfrac{1}{3.\sqrt[]{x-1}.\sqrt[3]{\left(\dfrac{1}{x-1}\right)^2}}\)
\(\Rightarrow y'=\dfrac{1}{3\left(x-1\right)^{\dfrac{1}{2}}.\left(x-1\right)^{\dfrac{2}{3}}}=\dfrac{1}{3\left(x-1\right)^{\dfrac{7}{6}}}=\dfrac{1}{3\sqrt[6]{\left(x-1\right)^7}}\)
d) \(y=\log_3\left(\dfrac{x+1}{x-1}\right)\)
\(\Rightarrow y'=\dfrac{\dfrac{1-\left(-1\right)}{\left(x-1\right)^2}}{\dfrac{x+1}{x-1}.\ln3}=\dfrac{2}{\left(x+1\right)\left(x-1\right).\ln3}\)
e) \(y=3^{x^2}\)
\(\Rightarrow y'=3^{x^2}.ln3.2x=2x.3^{x^2}.ln3\)
f) \(y=\left(\dfrac{1}{2}\right)^{x^2-1}\)
\(\Rightarrow y'=\left(\dfrac{1}{2}\right)^{x^2-1}.ln\dfrac{1}{2}.2x=2x.\left(\dfrac{1}{2}\right)^{x^2-1}.ln\dfrac{1}{2}\)
Các bài còn lại bạn tự làm nhé!

d: ĐKXĐ: \(x^2-1< >0\)
=>\(x^2\ne1\)
=>\(x\notin\left\{1;-1\right\}\)
Vậy: TXĐ là D=R\{1;-1}
b: ĐKXĐ: \(2-x^2>0\)
=>\(x^2< 2\)
=>\(-\sqrt{2}< x< \sqrt{2}\)
Vậy: TXĐ là \(D=\left(-\sqrt{2};\sqrt{2}\right)\)
a: ĐKXĐ: \(x-1>0\)
=>x>1
Vậy: TXĐ là \(D=\left(1;+\infty\right)\)
c: ĐKXĐ: \(x^2+x-6>0\)
=>\(x^2+3x-2x-6>0\)
=>\(\left(x+3\right)\left(x-2\right)>0\)
TH1: \(\left\{{}\begin{matrix}x+3>0\\x-2>0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>2\\x>-3\end{matrix}\right.\)
=>x>2
TH2: \(\left\{{}\begin{matrix}x+3< 0\\x-2< 0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x< -3\\x< 2\end{matrix}\right.\)
=>x<-3
Vậy: TXĐ là \(D=\left(2;+\infty\right)\cup\left(-\infty;-3\right)\)
e: ĐKXĐ: \(x^2-2>0\)
=>\(x^2>2\)
=>\(\left[{}\begin{matrix}x>\sqrt{2}\\x< -\sqrt{2}\end{matrix}\right.\)
Vậy: TXĐ là \(D=\left(-\infty;-\sqrt{2}\right)\cup\left(\sqrt{2};+\infty\right)\)
f: ĐKXĐ: \(\sqrt{x-1}>0\)
=>x-1>0
=>x>1
Vậy: TXĐ là \(D=\left(1;+\infty\right)\)
g: ĐKXĐ: \(x^2+x-6>0\)
=>\(\left(x+3\right)\left(x-2\right)>0\)
=>\(\left[{}\begin{matrix}x>2\\x< -3\end{matrix}\right.\)
Vậy: TXĐ là \(D=\left(2;+\infty\right)\cup\left(-\infty;-3\right)\)

`a)TXĐ: R`
`b)TXĐ: R\\{0}`
`c)TXĐ: R\\{1}`
`d)TXĐ: (-oo;-1)uu(1;+oo)`
`e)TXĐ: (-oo;-1/2)uu(1/2;+oo)`
`f)TXĐ: (-oo;-\sqrt{2})uu(\sqrt{2};+oo)`
`h)TXĐ: (-oo;0) uu(2;+oo)`
`k)TXĐ: R\\{1/2}`
`l)ĐK: {(x^2-1 > 0),(x-2 > 0),(x-1 ne 0):}`
`<=>{([(x > 1),(x < -1):}),(x > 2),(x ne 1):}`
`<=>x > 2`
`=>TXĐ: (2;+oo)`
câu l) $x^2-1 > 0$ thì giải ra 2 nghiệm $x < -1, x > 1$ mới đúng chứ nhỉ?

\(f'\left(x\right)=0\) có 3 nghiệm \(x=-1;0;2\)
Dấu của \(f'\left(x\right)\) trên trục số:
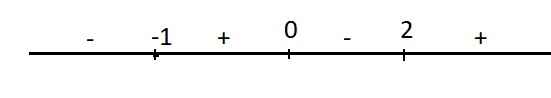
Ta thấy có 2 lần \(f'\left(x\right)\) đổi dấu từ âm sang dương nên hàm có 2 cực tiểu

2.
\(I=\int e^{3x}.3^xdx\)
Đặt \(\left\{{}\begin{matrix}u=3^x\\dv=e^{3x}dx\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}du=3^xln3dx\\v=\dfrac{1}{3}e^{3x}\end{matrix}\right.\)
\(\Rightarrow I=\dfrac{1}{3}e^{3x}.3^x-\dfrac{ln3}{3}\int e^{3x}.3^xdx=\dfrac{1}{3}e^{3x}.3^x-\dfrac{ln3}{3}.I\)
\(\Rightarrow\left(1+\dfrac{ln3}{3}\right)I=\dfrac{1}{3}e^{3x}.3^x\)
\(\Rightarrow I=\dfrac{1}{3+ln3}.e^{3x}.3^x+C\)
1.
\(I=\int\left(2x-1\right)e^{\dfrac{1}{x}}dx=\int2x.e^{\dfrac{1}{x}}dx-\int e^{\dfrac{1}{x}}dx\)
Xét \(J=\int2x.e^{\dfrac{1}{x}}dx\)
Đặt \(\left\{{}\begin{matrix}u=e^{\dfrac{1}{x}}\\dv=2xdx\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}du=-\dfrac{e^{\dfrac{1}{x}}}{x^2}dx\\v=x^2\end{matrix}\right.\)
\(\Rightarrow J=x^2.e^{\dfrac{1}{x}}+\int e^{\dfrac{1}{x}}dx\)
\(\Rightarrow I=x^2.e^{\dfrac{1}{x}}+C\)

a) Dùng phương pháp hữu tỉ hóa "Nếu \(f\left(x\right)=R\left(e^x\right)\Rightarrow t=e^x\)" ta có \(e^x=t\Rightarrow x=\ln t,dx=\frac{dt}{t}\)
Khi đó \(I_1=\int\frac{t^3}{t+2}.\frac{dt}{t}=\int\frac{t^2}{t+2}dt=\int\left(t-2+\frac{4}{t+2}\right)dt\)
\(=\frac{1}{2}t^2-2t+4\ln\left(t+2\right)+C=\frac{1}{2}e^{2x}-2e^x+4\ln\left(e^x+2\right)+C\)
b) Hàm dưới dấu nguyên hàm
\(f\left(x\right)=\frac{\sqrt{x}}{x+\sqrt[3]{x^2}}=R\left(x;x^{\frac{1}{2}},x^{\frac{2}{3}}\right)\)
q=BCNN(2;3)=6
Ta thực hiện phép hữu tỉ hóa theo :
"Nếu \(f\left(x\right)=R\left(x:\left(ã+b\right);\left(ax+b\right)^{r2},....\right),r_k=\frac{P_k}{q_k}\in Q,k=1,2,...,m\Rightarrow t=\left(ax+b\right)^{\frac{1}{q}}\),q=BCNN \(\left(q_1,q_2,...,q_m\right)\)"
=> \(t=x^{\frac{1}{6}}\Rightarrow x=t^{6,}dx=6t^5dt\)
Khi đó nguyên hàm đã cho trở thành :
\(I_2=\int\frac{t^3}{t^6-t^4}6t^{5dt}=\int\frac{6t^4}{t^2-1}dt=6\int\left(t^2+1+\frac{1}{t^2-1}\right)dt\)
\(=6\int\left(t^2+1\right)dt+2\int\frac{dt}{\left(t-1\right)\left(t+1\right)}=2t^3+6t+3\int\frac{dt}{t-1}-3\int\frac{dt}{t+1}\)
\(=2t^2+6t+3\ln\left|t-1\right|-3\ln\left|t+1\right|+C=2\sqrt{x}+6\sqrt[6]{x}+3\ln\left|\frac{\sqrt[6]{x-1}}{\sqrt[6]{x+1}}\right|+C\)
c) Hàm dưới dấu nguyên hàm có dạng :
\(f\left(x\right)=R\left(x;\left(\frac{x+1}{x-1}\right)^{\frac{2}{3}};\left(\frac{x+1}{x-1}\right)^{\frac{5}{6}}\right)\)
q=BCNN (3;6)=6
Ta thực hiện phép hữu tỉ hóa được
\(t=\left(\frac{x+1}{x-1}\right)^{\frac{1}{6}}\Rightarrow x=\frac{t^6+1}{t^6-1},dx=\frac{-12t^5}{\left(t^6-1\right)^2}dt\)
Khi đó hàm dưới dấu nguyên hàm trở thành
\(R\left(t\right)=\frac{1}{\left(\frac{t^6+1}{t^6-1}\right)^2-1}\left[t^4-t^5\right]=\frac{\left(t^6-1\right)^2}{4t^6}\left(t^4-t^5\right)\)
Do đó :
\(I_3=\int\frac{\left(t^6-1\right)^2}{4t^6}\left(t^4-t^5\right).\frac{-12t^5}{\left(t^6-1\right)}dt=3\int\left(t^4-t^3\right)dt\)
\(=\frac{5}{3}t^5-\frac{3}{4}t^4+C=\frac{3}{5}\sqrt[6]{\left(\frac{x+1}{x-1}\right)^5}-\frac{3}{4}\sqrt[3]{\left(\frac{x+1}{x-1}\right)^2}+C\)

a) \(\int\dfrac{2dx}{x^2-5x}=\int\left(\dfrac{-2}{5x}+\dfrac{2}{5\left(x-5\right)}\right)dx=-\dfrac{2}{5}ln\left|x\right|+\dfrac{2}{5}ln\left|x-5\right|+C\)
\(\Rightarrow A=-\dfrac{2}{5};B=\dfrac{2}{5}\Rightarrow2A-3B=-2\)
b) \(\int\dfrac{x^3-1}{x+1}dx=\int\dfrac{x^3+1-2}{x+1}dx=\int\left(x^2-x+1-\dfrac{2}{x+1}\right)dx=\dfrac{1}{3}x^3-\dfrac{1}{2}x^2+x-2ln\left|x+1\right|+C\)
\(\Rightarrow A=\dfrac{1}{3};B=\dfrac{1}{2};E=-2\Rightarrow A-B+E=-\dfrac{13}{6}\)

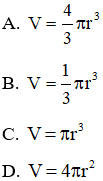
Chọn C
Đặt u = 1 - 2 x d v = e x d x ⇒ d u = - 2 d x v = e x
⇒ ∫ ( 1 - 2 x ) e x d x = ( 1 - 2 x ) e x + ∫ 2 e x d x = 1 - 2 x e x + 2 e x + C = e x 3 - 2 x + C