TZ
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
17 tháng 4 2019
\(A=\frac{x^2+x+1}{x^2+2x+2}\Leftrightarrow A\left(x^2+2x+2\right)=x^2+x+1\)
\(\Leftrightarrow Ax^2+2Ax+2A-x^2-x-1=0\)
\(\Leftrightarrow x^2\left(A-1\right)+x\left(2A-1\right)+\left(2A-1\right)=0\)
\(\Delta=\left(2A-1\right)^2-4\left(2A-1\right)\left(A-1\right)\ge0\)
\(\Rightarrow\left(2A-1\right)^2-\left(4A-4\right)\left(2A-1\right)\ge0\)
\(\Rightarrow\left(2A-1\right)\left(2A-1-4A+4\right)\ge0\)
\(\Rightarrow\left(2A-1\right)\left(3-2A\right)\ge0\Rightarrow\frac{1}{2}\le A\le\frac{3}{2}\)
TT
0
15 tháng 11 2018
2.
a/ Áp dụgn hệ quả bđt cô si,ta có :
\(A=xy+yz+zx\le\dfrac{\left(x+y+z\right)}{3}=\dfrac{a^2}{3}\)
Vậy GTLN A =a^2/3 khi x= y =z =a/3
b/Áp dụng BĐT Cô-Si dạng Engel,ta có :
\(B=\dfrac{x^2}{1}+\dfrac{y^2}{1}+\dfrac{z^2}{z}\ge\dfrac{\left(x+y+z\right)^2}{3}=\dfrac{a^2}{3}\)
Vậy GTNN của B = a^2/2 khi x=y=z =a/3
15 tháng 11 2018
\(B=\dfrac{3x}{1-x}+\dfrac{4\left(1-x\right)}{x}+7\ge2\sqrt{\dfrac{3x}{1-x}.\dfrac{4\left(1-x\right)}{x}}+7=7+4\sqrt{3}=\left(2+\sqrt{3}\right)^2\)
Vậy min B = \(\left(2+\sqrt{3}\right)^2\) khi \(\dfrac{3x}{1-x}=\dfrac{4\left(1-x\right)}{x}\Leftrightarrow x=\left(\sqrt{3}-1\right)^2\)
21 tháng 5 2015
1. x≥1 <=> \(\frac{1}{x}\le1\Leftrightarrow\frac{1}{x}+1\le2\Leftrightarrow A\le2\Rightarrow MaxA=2\Leftrightarrow x=1\)
2. Áp dụng bđt cosi cho x>0. ta có: \(x+\frac{1}{x}\ge2\sqrt{x.\frac{1}{x}}=2\Leftrightarrow P\ge2\Rightarrow MinP=2\Leftrightarrow x=\frac{1}{x}\Leftrightarrow x=1\)
21 tháng 5 2015
3: \(A=\frac{x^2+x+4}{x+1}=\frac{\left(x^2+2x+1\right)-\left(x+1\right)+4}{x+1}=x+1-1+\frac{4}{x+1}\)
áp dụng cosi cho 2 số dương ta có: \(x+1+\frac{4}{x+1}\ge2\sqrt{x+1.\frac{4}{x+1}}=2\Leftrightarrow A+1\ge2\Rightarrow A\ge3\Rightarrow MinA=3\Leftrightarrow x+1=\frac{4}{x+1}\Leftrightarrow x=1\)
CR
1
AH
Akai Haruma
Giáo viên
17 tháng 6 2019
Lời giải:
\(A=\sqrt{x^2-4x+7}=\sqrt{x^2-4x+4+3}=\sqrt{(x-2)^2+3}\)
Vì \((x-2)^2\geq 0, \forall x\in\mathbb{R}\Rightarrow A=\sqrt{(x-2)^2+3}\geq \sqrt{0+3}=\sqrt{3}\)
Vậy GTNN của $A$ là $\sqrt{3}$ khi $(x-2)^2=0$ hay $x=2$
----------------
\(B=1+\sqrt{2x-x^2+1}=1+\sqrt{2-(x^2-2x+1)}\)
\(=1+\sqrt{2-(x-1)^2}\)
Vì \((x-1)^2\geq 0, \forall x\in\mathbb{R}\Rightarrow 2-(x-1)^2\leq 2\)
\(\Rightarrow B=1+\sqrt{2-(x-1)^2}\leq 1+\sqrt{2}\)
Vậy GTLN của $B$ là $1+\sqrt{2}$. Dấu "=" xảy ra khi \((x-1)^2=0\) hay $x=1$
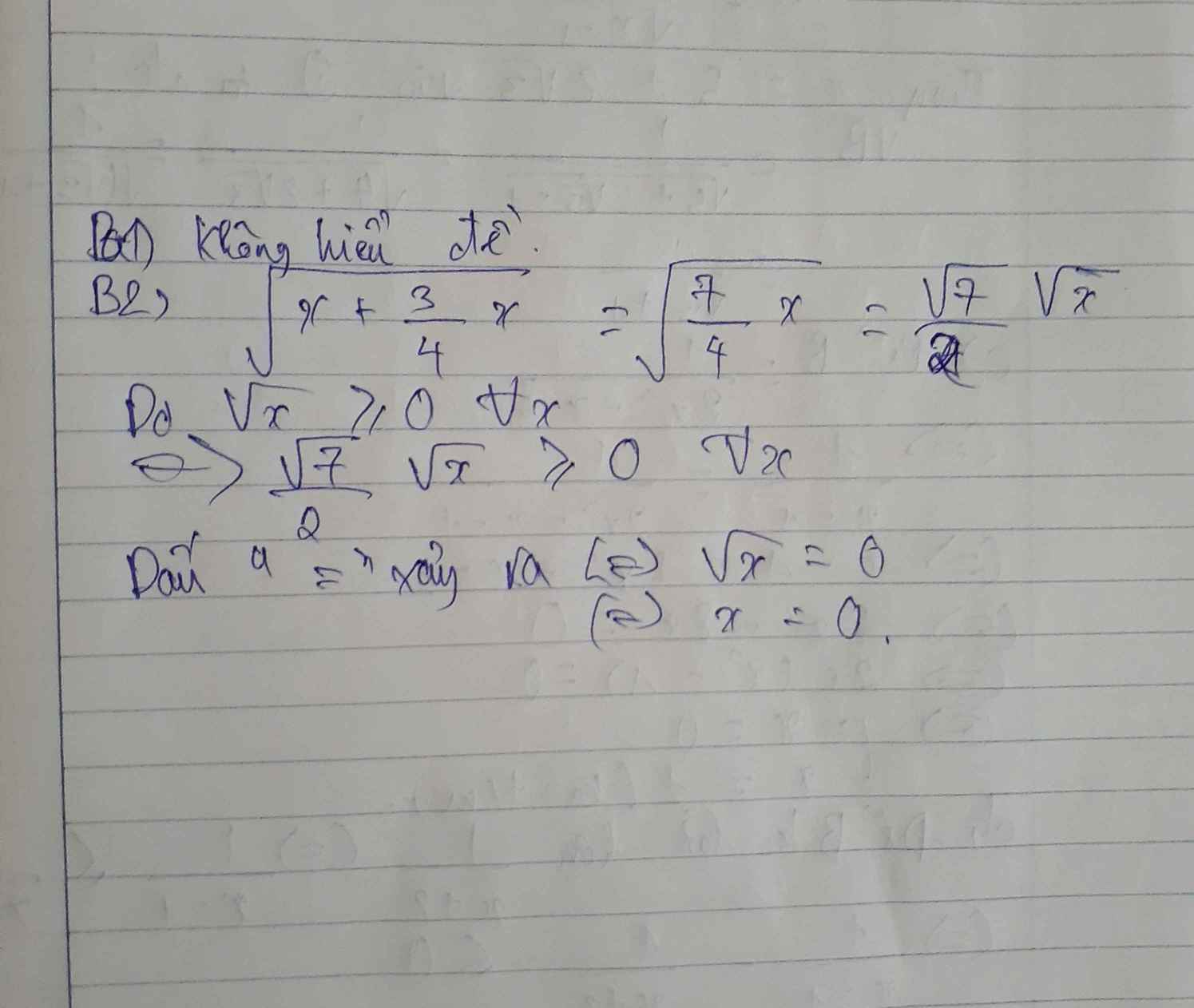
Dùng miền giá trị đi , lười làm quá