Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(a,=y\left(y-2\right)\\ b,=3x\left(x^2-2x+1\right)=3x\left(x-1\right)^2\\ c,=\left(y-1\right)\left(27x^2+9x^3\right)=9x^2\left(x+3\right)\left(y-1\right)\\ d,=y\left(y^2-2y+1\right)=y\left(y-1\right)^2\\ e,=x\left(x^2+6x+9\right)=x\left(x+3\right)^2\\ f,=x\left(x^2-2xy+y^2\right)=x\left(x-y\right)^2\\ g,=\left(2-x\right)\left(x+1\right)\\ h,=\left(x-1\right)\left(3x-6\right)=3\left(x-1\right)\left(x-2\right)\)
a: =y(y-2)
b: \(=3x^2\left(x^2-2x+1\right)=3x^2\left(x-1\right)^2\)
d: \(=y\left(y^2-2y+1\right)=y\left(y-1\right)^2\)

\(a,=\left(3x+2y\right)^3\\ b,=\left(4-x\right)^3\\ c,=\left(\dfrac{1}{2}x-3y\right)^3\)

a) A = ( x + 2 ) 3 nên x = 48 thì A = 125000.
b) B = ( 3 x – 2 y ) 3 nên x = 4; y = 6 thì B = 0.
c) C = x 2 − y − 2 3 nên x = 206; y 1 thì C = 10 6 .

Chia nhỏ ra cậu ơi :v
Cậu đặt câu hỏi free nên đặt nhỏ ra thì mới có người làm nha để như này dày cộp không ai dám làm đou =(((

`1)x^3-7x+6`
`=x^3-x-6x+6`
`=x(x-1)(x+1)-6(x-1)`
`=(x-1)(x^2+x-6)`
`=(x-1)(x^2-2x+3x-6)`
`=(x-1)[x(x-2)+3(x-2)]`
`=(x-1)(x-2)(x+3)`
`2)x^3-9x^2+6x+16`
`=x^3-2x^2-7x^2+14x-8x+16`
`=x^2(x-2)-7x(x-2)-8(x-2)`
`=(x-2)(x^2-7x-8)`
`=(x-2)(x^2-8x+x-8)`
`=(x-2)[x(x-8)+x-8]`
`=(x-2)(x-8)(x+1)`
`3)x^3-6x^2-x+30`
`=x^3+2x^2-8x^2-16x+15x+30`
`=x^2(x+2)-8x(x+2)+15(x+2)`
`=(x+2)(x^2-8x+15)`
`=(x+2)(x^2-3x-5x+15)`
`=(x+2)[x(x-3)-5(x-3)]`
`=(x+2)(x-3)(x-5)`
`4)2x^3-x^2+5x+3`
`=2x^3+x^2-2x^2-x+6x+3`
`=x^2(2x+1)-x(2x+1)+3(2x+1)`
`=(2x+1)(x^2-x+3)`
`5)27x^3-27x^2+18x-4`
`=27x^3-9x^2-18x^2+6x+12x-4`
`=9x^2(3x-1)-6x(3x-1)+4(3x-1)`
`=(3x-1)(9x^2-6x+4)`
1) Ta có: \(x^3-7x+6\)
\(=x^3-x-6x+6\)
\(=x\left(x^2-1\right)-6\left(x-1\right)\)
\(=\left(x-1\right)\left(x^2+x-6\right)\)
\(=\left(x-1\right)\left(x+3\right)\left(x-2\right)\)
2) Ta có: \(x^3-9x^2+6x+16\)
\(=x^3-2x^2-7x^2+14x-8x+16\)
\(=x^2\left(x-2\right)-7x\left(x-2\right)-8\left(x-2\right)\)
\(=\left(x-2\right)\left(x^2-7x-8\right)\)
\(=\left(x-2\right)\left(x-8\right)\left(x+1\right)\)
3) Ta có: \(x^3-6x^2-x+30\)
\(=x^3+2x^2-8x^2-16x+15x+30\)
\(=x^2\left(x+2\right)-8x\left(x+2\right)+15\left(x+2\right)\)
\(=\left(x+2\right)\left(x^2-8x+15\right)\)
\(=\left(x+2\right)\left(x-3\right)\left(x-5\right)\)

1A:
a: \(x^3+2x=x\left(x^2+2\right)\)
b: \(3x-6y=3\left(x-2y\right)\)
c: \(5\left(x+3y\right)-15x\left(x+3y\right)\)
\(=5\left(x+3y\right)\left(1-3x\right)\)
d: \(3\left(x-y\right)-5x\left(y-x\right)\)
\(=3\left(x-y\right)+5x\left(x-y\right)\)
\(=\left(x-y\right)\left(5x+3\right)\)
1A. a. x(x2+2)
b. 3(x-2y)
c. 5(x+3y)(1-3x)
d. (x-y) (3-5x)
1B. a. 2x(2x-3)
b.xy(x2-2xy+5)
c. 2x(x+1)(x+2)
d. 2x(y-1)+2y(y-1)=2(y-1)(x-y)

a) \(x^4-y^4\)
\(=\left(x^2\right)^2-\left(y^2\right)^2\)
\(=\left(x^2-y^2\right)\left(x^2+y^2\right)\)
\(=\left(x+y\right)\left(x-y\right)\left(x^2+y^2\right)\)
b) \(x^2-3y^2\)
\(=x^2-\left(y\sqrt{3}\right)^2\)
\(=\left(x-y\sqrt{3}\right)\left(x+y\sqrt{3}\right)\)
c) \(\left(3x-2y\right)^2-\left(2x-3y\right)^2\)
\(=\left(3x-2y+2x-3y\right)\left(3x-2y-3x+2y\right)\)
\(=0\cdot0\)
\(=0\)
d) \(9\left(x-y\right)^2-4\left(x+y\right)^2\)
\(=\left(3x-3y\right)^2-\left(2x+2y\right)^2\)
\(=\left(3x-3y-2x-2y\right)\left(3x-3y+2x+2y\right)\)
\(=\left(x-5y\right)\left(5x-y\right)\)
e) \(\left(4x^2-4x+1\right)-\left(x+1\right)^2\)
\(=\left(2x-1\right)^2-\left(x+1\right)^2\)
\(=\left(2x-1+x+1\right)\left(2x-1-x-1\right)\)
\(=3x\left(x-2\right)\)
f) \(x^3+27\)
\(=x^3+3^3\)
\(=\left(x+3\right)\left(x^2-3x+9\right)\)
g) \(27x^3-0,001\)
\(=\left(3x\right)^3-\left(0,1\right)^3\)
\(=\left(3x-0,1\right)\left(9x^2+0,3x+0,01\right)\)
h) \(125x^3-1\)
\(=\left(5x\right)^3-1^3\)
\(=\left(5x-1\right)\left(25x^2+5x+1\right)\)
c) \(\left(3x-2y\right)^2-\left(2x-3y\right)^2\)
\(=\left(3x-2y+2x-3y\right)\left(3x-2y-2x+3y\right)\)
\(=\left(5x-5y\right)\left(x+y\right)\)
\(=5\left(x+y\right)\left(x-y\right)\)


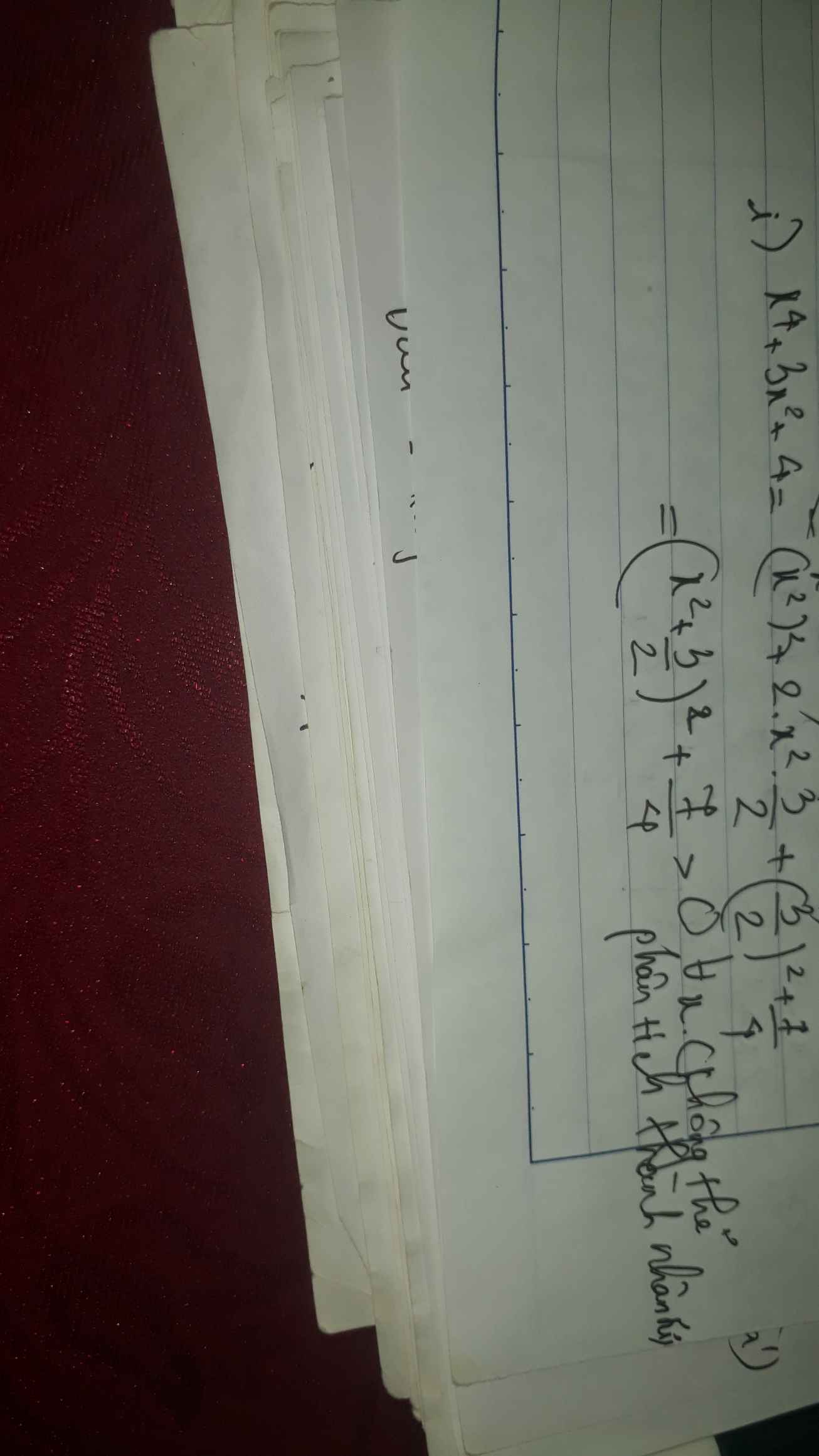
a, \(x^3-3x^2+3x-1=\left(x-1\right)^3\)
b, \(1-9x+27x^2-27x^3=-\left(3x-1\right)^3\)
Mình có làm ở câu dưới rồi . Bạn tham khảo link :
https://olm.vn/hoi-dap/detail/231817932107.html