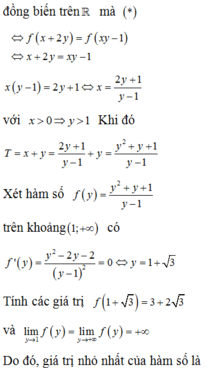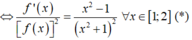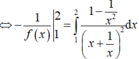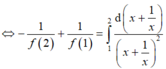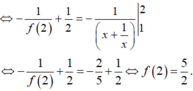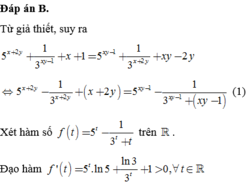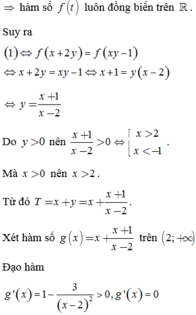Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: \(P=2\left(x-3\right)^2+5\ge5>0\forall x\)
nên P(x) vô nghiệm
b: \(Q\left(x\right)=x^4+x^2+2\ge2>0\forall x\)
nên Q(x) vô nghiệm

\(\begin{cases}\sqrt{x}+\sqrt{y}=3\left(1\right)\\\sqrt{x+5}+\sqrt{y+3}\le m\left(2\right)\end{cases}\)
Điều kiện \(\begin{cases}x\ge0\\y\ge0\end{cases}\)
Đặt \(t=\sqrt{x}\) lúc đó (1) có dạng \(\sqrt{y=3-1}\Leftrightarrow y=\left(t^2-6t+9\right)\)
Điều kiện của t : \(2\le t\)\(\le3\)
Khi đó (2) \(\Leftrightarrow\sqrt{t^2+5}+\sqrt{t^2-6t+12}\le m\)
Xét hàm số : \(f\left(t\right)=\sqrt{t^2+5}+\sqrt{t^2-6t+12}\)
- Miền xác định \(D=\left[2;3\right]\)
- Đạo hàm
\(f'\left(t\right)=\frac{t}{\sqrt{t^2+5}}+\frac{t-3}{\sqrt{t^2-6t+12}}\)
\(f'\left(t\right)=0\Leftrightarrow\frac{t}{\sqrt{t^2+5}}=\frac{3-t}{\sqrt{t^2-6t+12}}\)
\(\Leftrightarrow t\sqrt{t^2-6t+12}=\left(3-t\right)\sqrt{t^2+5}\)
\(\Leftrightarrow t^4-6t^3+12t^2=t^4-6t^3+14t^2-30t+45\)
\(\Leftrightarrow2t^2-30t+45=0\) vô nghiệm với \(x\in D\)
Mà \(f'\left(3\right)>0\Rightarrow f\left(t\right)\) đồng biến trên D do đó min \(f\left(2\right)=5\)
Để có nghiệm (x,y) thỏa mãn \(x\ge4\Leftrightarrow\) (2) có nghiệm thỏa mãn (1)
và \(x\ge4\Leftrightarrow f\left(t\right)\le m\) thỏa mãn với mọi \(2\le t\)\(\le3\)
\(\Leftrightarrow\) min \(f\left(t\right)\le m\Leftrightarrow m\ge5\)

Do \(x^2+y^2=1\), đặt \(\left\{{}\begin{matrix}x=sina\\y=cosa\end{matrix}\right.\)
\(P=\left(3-sina\right)\left(3-cosa\right)=9-3\left(sina+cosa\right)+sina.cosa\)
Đặt \(sina+cosa=t\Rightarrow t\in\left[-\sqrt{2};\sqrt{2}\right]\)
\(t^2=1+2sina.cosa\Rightarrow sina.cosa=\dfrac{t^2-1}{2}\)
\(P=9-3t+\dfrac{t^2-1}{2}=\dfrac{1}{2}t^2-3t+\dfrac{17}{2}\)
Xét hàm \(f\left(t\right)=\dfrac{1}{2}t^2-3t+\dfrac{17}{2}\) trên \(\left[-\sqrt{2};\sqrt{2}\right]\)
\(f'\left(t\right)=t-3=0\Rightarrow t=3\notin\left[-\sqrt{2};\sqrt{2}\right]\)
\(f\left(-\sqrt{2}\right)=\dfrac{19+6\sqrt{2}}{2}\) ; \(f\left(\sqrt{2}\right)=\dfrac{19-6\sqrt{2}}{2}\)
\(\Rightarrow P_{min}=f\left(\sqrt{2}\right)=\dfrac{19-6\sqrt{2}}{2}\) khi \(t=\sqrt{2}\)

Đáp án B.
Từ giả thiết, suy ra
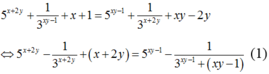
Xét hàm số f ( t ) = 5 t - 1 3 t + t trên ℝ .
Đạo hàm f ' ( t ) = 5 t . ln 5 - ln 3 3 t + 1 > 0 , ∀ t ∈ ℝ ⇒ hàm số f ( t ) luôn đồng biến trên ℝ .
Suy ra
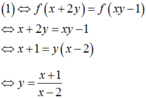
Do y > 0 nên x + 1 x - 2 > 0 ⇔ [ x > 2 x < - 1 . Mà x > 0 nên x > 2 .
Từ đó T = x + y = x + x + 1 x - 2 . Xét hàm số g ( x ) = x + x + 1 x - 2 trên 2 ; + ∞ .
Đạo hàm
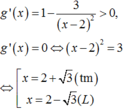
Lập bảng biến thiên của hàm số trên 2 ; + ∞ , ta thấy min g ( x ) = g ( 2 + 3 ) = 3 + 2 3 .
Vậy T m i n = 3 + 2 3 khi x = 2 + 3 và x = 1 + 3 .