LN
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NN
0
H
4 tháng 7 2021
Câu 2 : C
Câu 3 : A
Câu 4 : C
Câu 5 : C
Câu 6 : B
Câu 7 : C
Câu 8 : D
Câu 9 : B
4 tháng 7 2021
Câu 2: C
Pt\(\Leftrightarrow\left\{{}\begin{matrix}x-2\ge0\\x^2+5x-2=\left(x-2\right)^2\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}x\ge2\\9x=6\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}x\ge2\\x=\dfrac{6}{9}\end{matrix}\right.\)\(\Rightarrow x\in\varnothing\)
Câu 3: A
\(\Delta:3x+4y-11=0\)
\(d_{\left(M;\Delta\right)}=\dfrac{\left|3.1+4.-1-11\right|}{\sqrt{3^2+4^2}}=\dfrac{12}{5}\)
Câu 4: Ko có đ/a
Do \(\dfrac{\pi}{2}< \alpha< \pi\Rightarrow tan\alpha< 0;cot\alpha< 0;cos\alpha< 0\)
\(1+cot^2\alpha=\dfrac{1}{sin^2\alpha}\)\(\Rightarrow cot\alpha=\dfrac{-\sqrt{21}}{2}\)
Câu 5:C
Câu 6:B
Câu 7: A
Có nghiệm khi \(\left(m;+\infty\right)\cup\left[-2;2\right]\ne\varnothing\)
\(\Leftrightarrow m< 2\)
Câu 8:D
Câu 9: B
\(cos2\alpha=2cos^2\alpha-1=-\dfrac{23}{25}\)
Câu 10:D
NN
0
LN
2
NV
Nguyễn Việt Lâm
Giáo viên
25 tháng 7 2021
3.
Do \(sin\left(x+k2\pi\right)=sinx\Rightarrow sin\left(x+2020\pi\right)=sinx\)
\(sin\left(\dfrac{\pi}{2}+x\right)=cos\left(\dfrac{\pi}{2}-\dfrac{\pi}{2}-x\right)=cos\left(-x\right)=cosx\)
\(A=\dfrac{sinx+sin3x+sin5x}{cosx+cos3x+cos5x}=\dfrac{sinx+sin5x+sin3x}{cosx+cos5x+cos3x}\)
\(=\dfrac{2sin3x.cosx+sin3x}{2cos3x.cosx+cos3x}=\dfrac{sin3x\left(2cosx+1\right)}{cos3x\left(2cosx+1\right)}\)
\(=\dfrac{sin3x}{cos3x}=tan3x\)
NV
Nguyễn Việt Lâm
Giáo viên
25 tháng 7 2021
4.
a.
\(\overrightarrow{CB}=\left(2;-2\right)=2\left(1;-1\right)\)
Do đường thẳng d vuông góc BC nên nhận \(\left(1;-1\right)\) là 1 vtpt
Phương trình đường thẳng d đi qua \(A\left(-1;2\right)\) và có 1 vtpt là \(\left(1;-1\right)\) là:
\(1\left(x+1\right)-1\left(y-2\right)=0\Leftrightarrow x-y+3=0\)
b.
Gọi \(I\left(a;b\right)\) là tâm đường tròn, ta có \(\left\{{}\begin{matrix}\overrightarrow{AI}=\left(a+1;b-2\right)\\\overrightarrow{BI}=\left(a-3;b-2\right)\\\overrightarrow{CI}=\left(a-1;b-4\right)\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}AI^2=\left(a+1\right)^2+\left(b-2\right)^2\\BI^2=\left(a-3\right)^2+\left(b-2\right)^2\\CI^2=\left(a-1\right)^2+\left(b-4\right)^2\end{matrix}\right.\)
Do I là tâm đường tròn qua 3 điểm nên: \(\left\{{}\begin{matrix}AI=BI\\AI=CI\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}AI^2=BI^2\\AI^2=CI^2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left(a+1\right)^2+\left(b-2\right)^2=\left(a-3\right)^2+\left(b-2\right)^2\\\left(a+1\right)^2+\left(b-2\right)^2=\left(a-1\right)^2+\left(b-4\right)^2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}8a=8\\4a+4b=12\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}a=1\\b=2\end{matrix}\right.\) \(\Rightarrow I\left(1;2\right)\)
\(\overrightarrow{AI}=\left(2;0\right)\Rightarrow R=AI=\sqrt{2^2+0^2}=2\)
Pt đường tròn có dạng:
\(\left(x-1\right)^2+\left(y-2\right)^2=4\)
NV
Nguyễn Việt Lâm
Giáo viên
20 tháng 7 2021
2.
Xét BPT: \(\left(x+3\right)\left(4-x\right)>0\Leftrightarrow-3< x< 4\) \(\Rightarrow D_1=\left(-3;4\right)\)
Xét BPT: \(x< m-1\) \(\Rightarrow D_2=\left(m-1;+\infty\right)\)
Hệ có nghiệm khi và chỉ khi \(D_1\cap D_2\ne\varnothing\)
\(\Leftrightarrow m-1< 4\)
\(\Leftrightarrow m< 5\)
3.
\(\dfrac{\pi}{24}=\dfrac{180^0}{24}=7^030'\)
4.
\(x^2+y^2-x+y+4=0\) không phải đường tròn
Do \(\left(\dfrac{1}{2}\right)^2+\left(-\dfrac{1}{2}\right)^2-4< 0\)
NV
Nguyễn Việt Lâm
Giáo viên
20 tháng 7 2021
5.
\(f\left(x\right)=ax^2+bx+c\) có \(\left\{{}\begin{matrix}a\ne0\\\Delta=b^2-4ac< 0\end{matrix}\right.\) thì \(f\left(x\right)\) không đổi dấu trên R
6.
\(sin2020a=sin\left(2.1010a\right)=2sin1010a.cos1010a\)
7.
Công thức B sai
\(cos^2a+sin^2a=1\) , không phải \(cos2a\)
NV
0
NV
4
HP
27 tháng 3 2021
1.
\(cosA=\dfrac{b^2+c^2-a^2}{2bc}=\dfrac{1}{2}\Rightarrow\widehat{A}=60^o\)
\(S=\dfrac{1}{2}bc.sinA=\dfrac{1}{2}.8.5.sin60^o=10\sqrt{3}\)
\(S=\dfrac{1}{2}a.h_a=\dfrac{1}{2}.7.h_a=10\sqrt{3}\Rightarrow h_a=\dfrac{20\sqrt{3}}{7}\)
\(2R=\dfrac{a}{sinA}=\dfrac{7}{\dfrac{\sqrt{3}}{2}}=\dfrac{14\sqrt{3}}{3}\Rightarrow R=\dfrac{7\sqrt{3}}{3}\)
\(S=pr=\dfrac{a+b+c}{2}.r=10r=10\sqrt{3}\Rightarrow r=\sqrt{3}\)
\(m_a^2=\dfrac{b^2+c^2}{2}-\dfrac{a^2}{4}=\dfrac{129}{4}\Rightarrow m_a=\dfrac{\sqrt{129}}{2}\)
HP
27 tháng 3 2021
6.
a, Công thức trung tuyến:
\(AM^2=c^2=\dfrac{b^2+c^2}{2}-\dfrac{a^2}{4}=\dfrac{2b^2+2c^2-a^2}{4}\Rightarrow a^2=2\left(b^2-c^2\right)\)
b, \(a^2=2\left(b^2-c^2\right)\Rightarrow\dfrac{2\left(b^2-c^2\right)}{a^2}=1\)
\(\Leftrightarrow2\left(\dfrac{b^2}{a^2}-\dfrac{c^2}{a^2}\right)=1\)
\(\Leftrightarrow2\left(\dfrac{b^2}{a^2}.sin^2A-\dfrac{c^2}{a^2}.sin^2A\right)=sin^2A\)
\(\Leftrightarrow2\left(sin^2B-sin^2C\right)=sin^2A\)
Hay \(sin^2A=2\left(sin^2B-sin^2C\right)\)
NV
Nguyễn Việt Lâm
Giáo viên
24 tháng 6 2021
1.
\(x^2+y^2-2x+4y+1=0\)
\(\Leftrightarrow\left(x-1\right)^2+\left(y+2\right)^2=4\)
Đường tròn tâm \(I\left(1;-2\right)\) bán kính \(R=2\)
2.
\(\left\{{}\begin{matrix}3x-7>0\\x+8>0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x>\dfrac{7}{3}\\x>-8\end{matrix}\right.\)
Lấy giao của 2 tập trên ta được nghiệm của BĐT là:
\(\left(\dfrac{7}{3};+\infty\right)\)
3.
Pt đã cho có 2 nghiệm trái dấu khi và chỉ khi:
\(ac< 0\Leftrightarrow1.\left(1-3m\right)< 0\)
\(\Leftrightarrow m>\dfrac{1}{3}\)
NV
Nguyễn Việt Lâm
Giáo viên
24 tháng 6 2021
4.
Lập bảng xét dấu:
Từ bảng xét dấu ta được nghiệm của BPT:
\(\left(-\infty;-2\right)\cup[1;+\infty)\)
5.
Hàm số có 2 nghiệm \(x=\left\{1;2\right\}\) đồng thời 2 khoảng chứa vô cực mang dấu âm nên có dạng:
\(f\left(x\right)=-\left(x-1\right)\left(x-2\right)=\left(x-1\right)\left(-x+2\right)\)
NV
Nguyễn Việt Lâm
Giáo viên
29 tháng 7 2021
18.
Do D thuộc trục hoành nên tọa độ có dạng: \(D\left(a;0;0\right)\)
\(\Rightarrow\left\{{}\begin{matrix}\overrightarrow{AD}=\left(a-3;4;0\right)\\\overrightarrow{BC}=\left(4;0;-3\right)\end{matrix}\right.\)
\(AD=BC\Leftrightarrow\left(a-3\right)^2+4^2=4^2+\left(-3\right)^2\)
\(\Rightarrow\left(a-3\right)^2=9\Rightarrow\left[{}\begin{matrix}a=0\\a=6\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}D\left(0;0;0\right)\\D\left(6;0;0\right)\end{matrix}\right.\)
19.
\(cos\left(\overrightarrow{a};\overrightarrow{b}\right)=\dfrac{2.\left(-1\right)+1.0+0.\left(-2\right)}{\sqrt{2^2+1^2+0^2}.\sqrt{\left(-1\right)^2+0^2+\left(-2\right)^2}}=-\dfrac{2}{5}\)
20.
\(\overrightarrow{OA}=\left(2;2;1\right)\Rightarrow OA=\sqrt{2^2+2^2+1^2}=3\)
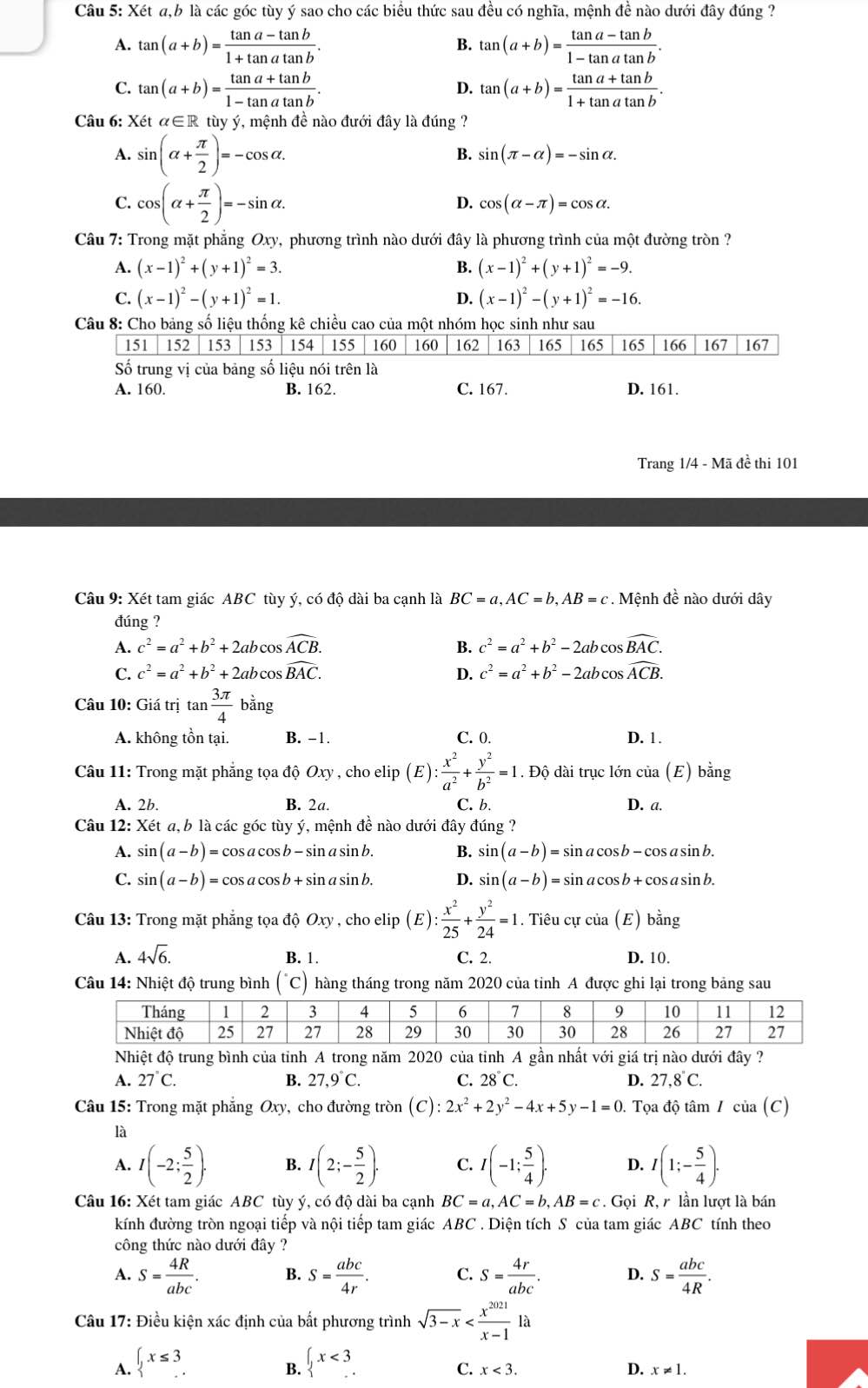
5C (công thức trong SGK, ko có gì cần tự luận ở đây)
6C: \(cos\left(a+\dfrac{\pi}{2}\right)=sin\left[\dfrac{\pi}{2}-\left(a+\dfrac{\pi}{2}\right)\right]=sin\left(-a\right)=-sina\)
7A: lý thuyết SGK, pt đường tròn có dạng \(\left(x-a\right)^2+\left(y-b\right)^2=R^2\)
8A
Viết lại mẫu theo thứ tự và loại đi các mẫu lặp:
151 152 153 154 155 160 162 163 165 166 167
Từ đây ta thấy số trung vị là 160
9B: công thức định lý hàm cos trong SGK
10B (bấm máy)
11B (lý thuyết elip SGK)
12B (công thức lượng giác SGK)
13C.
Từ pt (E) ta thấy \(\left\{{}\begin{matrix}a^2=25\\b^2=24\end{matrix}\right.\) \(\Rightarrow c^2=a^2-b^2=1\Rightarrow c=1\)
Tiêu cự \(=2c=2\)
14D
\(\overline{t}=\dfrac{25+27+27+28+29+30+30+30+28+26+27+27}{12}\approx27,8\)
15D
\(\Leftrightarrow x^2+y^2-2x+\dfrac{5}{2}y-\dfrac{1}{2}=0\)
\(\Rightarrow I\left(1;-\dfrac{5}{4}\right)\)
16D (công thức SGK)