K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
3 tháng 7 2021
a) Ta có: \(P=\dfrac{a\sqrt{a}-1}{a-\sqrt{a}}-\dfrac{a\sqrt{a}+1}{a+\sqrt{a}}+\left(\sqrt{a}-\dfrac{1}{\sqrt{a}}\right)\left(\dfrac{3\sqrt{a}}{\sqrt{a}-1}-\dfrac{\sqrt{a}+2}{\sqrt{a}+1}\right)\)
\(=\dfrac{\left(\sqrt{a}-1\right)\left(a+\sqrt{a}+1\right)}{\sqrt{a}\left(\sqrt{a}-1\right)}-\dfrac{\left(\sqrt{a}+1\right)\left(a-\sqrt{a}+1\right)}{\sqrt{a}\left(\sqrt{a}+1\right)}+\dfrac{a-1}{\sqrt{a}}\cdot\dfrac{3\sqrt{a}\left(\sqrt{a}+1\right)-\left(\sqrt{a}+2\right)\left(\sqrt{a}-1\right)}{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}\)
\(=\dfrac{a+\sqrt{a}+1-a+\sqrt{a}-1}{\sqrt{a}}+\dfrac{3a+3\sqrt{a}-\left(a-\sqrt{a}+2\sqrt{a}-2\right)}{\sqrt{a}}\)
\(=2+\dfrac{3a+3\sqrt{a}-a+\sqrt{a}-2\sqrt{a}+2}{\sqrt{a}}\)
\(=\dfrac{2\sqrt{a}+2a+2\sqrt{a}+2}{\sqrt{a}}\)
\(=\dfrac{2\left(a+2\sqrt{a}+1\right)}{\sqrt{a}}\)
\(=\dfrac{2\left(\sqrt{a}+1\right)^2}{\sqrt{a}}\)
b) Ta có: \(P-6=\dfrac{2\left(\sqrt{a}+1\right)^2-6\sqrt{a}}{\sqrt{a}}\)
\(=\dfrac{2a+4\sqrt{a}+2-6\sqrt{a}}{\sqrt{a}}\)
\(=\dfrac{2\left(a-\sqrt{a}+1\right)}{\sqrt{a}}>0\forall a\) thỏa mãn ĐKXĐ
hay P>6
AH
Akai Haruma
Giáo viên
7 tháng 7 2021
Lời giải:
$A=4n^3-36n^2+56n=4n(n^2-9n+14)=4n(n-2)(n-7)$
Vì $n-2,n-7$ khác tính chẵn lẻ nên 1 trong 2 số sẽ là số chẵn.
$\Rightarrow n(n-2)(n-7)\vdots 2$
$\Rightarrow =4n(n-2)(n-7)\vdots 8(*)$
Lại có:
Nếu $n$ chia hết cho $3$ thì $A=4n(n-2)(n-7)\vdots 3$
Nếu $n$ chia $3$ dư $1$ thì $n-7\vdots 3\Rightarrow A\vdots 3$
Nếu $n$ chia $3$ dư $2$ thì $n-2\vdots 3\Rightarrow A\vdots 3$
Tóm lại $A\vdots 3(**)$
Từ $(*); (**)$ mà $(3,8)=1$ nên $A\vdots 24$.
Ta có đpcm.
XO
3 tháng 10 2020
Ta có : \(\hept{\begin{cases}\left|x^2+x-2\right|\ge0\forall x\\\left|x^2-1\right|\ge0\forall x\end{cases}}\Rightarrow\left|x^2+x-2\right|+\left|x^2-1\right|\ge0\forall x\)
Đẳng thức |x2 + x - 2| + |x2 - 1| = 0 xảy ra
<=> \(\hept{\begin{cases}x^2+x-2=0\\x^2-1=0\end{cases}}\Rightarrow\hept{\begin{cases}x^2+2x-x-2=0\\x^2=1\end{cases}}\Rightarrow\hept{\begin{cases}\left(x+2\right)\left(x-1\right)=0\\x^2=1\end{cases}}\)
+) Nếu : (x + 2)(x - 1) = 0
=> \(\orbr{\begin{cases}x+2=0\\x-1=0\end{cases}}\Rightarrow\orbr{\begin{cases}x=-2\\x=1\end{cases}}\)
+) Nếu x2 = 1
=> \(\orbr{\begin{cases}x=1\\x=-1\end{cases}}\)
Vậy x = 1
NP
1
25 tháng 12 2020
\(\Leftrightarrow\left\{{}\begin{matrix}x^3+2y^2-4y+3=0\\2x^2+2x^2y^2-4y=0\left(1\right)\end{matrix}\right.\Rightarrow}x^3+2y^2-4y-2x^2-2x^2y^2+4y=0\Rightarrow x^3+1-2x^2y^2+2y^2-2x^2+2=0\Rightarrow\left(x+1\right)\left(x^2-x+1\right)-2y^2\left(x-1\right)\left(x+1\right)-2\left(x-1\right)\left(x+1\right)=0\Rightarrow\left(x+1\right)\left(x^2-x+1-2xy^2+2y^2-2x+2\right)=0\Rightarrow x=-1\)Thay x=-1 vào (1) ta được y2-2y+1=0⇒ (y-1)2=0⇒y-1=0⇒y=1
Do đó Q=x2+y2=(-1)2+12=2
V
3
2 tháng 7 2017
=(2^2-1)*(2^2+1)*(2^4+1)*...*(2^64+1)
=(2^4-1)(2^2+1)*(2^4+1)*...*(2^64+1)
=(2^8-1)(2^8+1)*...*(2^64+1)
=...
=(2^64-1)(2^64+1)
=2^128-1
mk vua lm ra do!!!!!!
VT
2
17 tháng 9 2019
\(\left(x^2+2x\right)^2-2x^2-4x=4\)
\(\Leftrightarrow\left(x^2+2x\right)^2-2\left(x^2+2x\right)=4\)
Đặt \(x^2+2x=t\)
pt <=> \(t^2-2t=4\)
\(\Leftrightarrow t^2-2t-4=0\)
...
KY
17 tháng 9 2019
\(\left(x^2+2x\right)^2-2x^2-4x=4\)
\(\Leftrightarrow\left(x^2+2x\right)^2-2\left(x^2+2\right)=4\)
Đặt \(x^2+2x=a\)
\(\Rightarrow pt\Leftrightarrow a^2-2a=4\Leftrightarrow a^2-2a-4=0\)
\(\cdot\Delta=\left(-2\right)^2-4.\left(-4\right)=20,\sqrt{\Delta}=\sqrt{20}\)
Vậy pt ẩn phụ có 2 nghiệm phân biệt
\(a_1=\frac{2+\sqrt{20}}{2}=\sqrt{5}+1\);\(a_2=\frac{2-\sqrt{20}}{2}=1-\sqrt{5}\)
Thay vào \(x^2+2x=a\),dùng delta giải.
M
a) | x-1 | + | x^2+3 | = 0
b) | x-1 | + | x^2 -1 | = 0
c) | x^2 -4 | + | x | = 2
mn ưi giúp mk vs ạ :((
2
NC
4 tháng 10 2020
a) Ta có: \(\left|x-1\right|+\left|x^2+3\right|=0\)
\(\Leftrightarrow\left|x-1\right|=-\left|x^2+3\right|\)
Mà \(\hept{\begin{cases}\left|x-1\right|\ge0\\-\left|x^2+3\right|\le0\end{cases}\left(\forall x\right)}\)
Dấu "=" xảy ra khi: \(\left|x-1\right|=-\left|x^2+3\right|=0\)
\(\Rightarrow x^2=-3\) => vô lý
Vậy PT vô nghiệm
NC
4 tháng 10 2020
b) Ta có: \(\left|x-1\right|+\left|x^2-1\right|=0\)
\(\Leftrightarrow\left|x-1\right|=-\left|x^2-1\right|\)
Mà \(\hept{\begin{cases}\left|x-1\right|\ge0\\-\left|x^2-1\right|\le0\end{cases}\left(\forall x\right)}\)
Dấu "=" xảy ra khi: \(\left|x-1\right|=-\left|x^2-1\right|=0\)
\(\Leftrightarrow\hept{\begin{cases}x=1\\x^2=1\end{cases}}\Rightarrow x=1\)
Vậy x = 1
28 tháng 2 2020
viết lại đề nhé
suy ra M=\(\frac{\left(2-x\right)^2}{\left(x-1\right)^2\left(2-x\right)^2}+\frac{\left(x-1\right)^2}{\left(x-1\right)^2\left(2-x\right)^2}+\frac{1}{\left(x-1\right)^2\left(2-x\right)^2}\)
=\(\frac{\left(2-x\right)^2+\left(x-1\right)^2+1}{\left(x-1\right)^2\left(2-x\right)^2}\)
suy ra \(\left(2-x\right)^2+\left(x-1\right)^2+1>hoặc=1\)
vậy MinM=1 khi 2-x=x-1
11 tháng 3 2022
Bài 2
a, bạn tự vẽ
b, Hoành độ giao điểm tm pt
\(2x^2-2x+3=0\)
\(\Delta'=1-3.2=-5< 0\)
Vậy pt vô nghiệm hay (d) ko cắt (P)
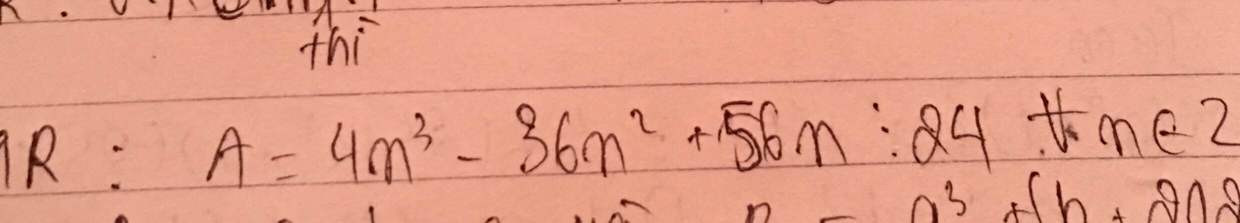
Vì \(\dfrac{1}{2}\ne\dfrac{2}{-3}\)
nên hệ luôn có nghiệm duy nhất
\(\left\{{}\begin{matrix}x+2y=m+3\\2x-3y=m\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2x+4y=2m+6\\2x-3y=m\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2x+4y-2x+3y=2m+6-m=m+6\\x+2y=m+3\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}7y=m+6\\x=m+3-2y\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=\dfrac{m+6}{7}\\x=m+3-2\cdot\dfrac{m+6}{7}\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=\dfrac{m+6}{7}\\x=\dfrac{7m+21-2m-12}{7}=\dfrac{5m+9}{7}\end{matrix}\right.\)
x+y=-3
=>\(\dfrac{5m+9+m+6}{7}=-3\)
=>6m+15=-21
=>6m=-36
=>m=-6