Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

TA CÓ: OI LÀ TIA NẰM GIỮA OA; OB
\(\Rightarrow\widehat{AOI}+\widehat{IOB}=\widehat{AOB}\)
MÀ: \(\widehat{AOI}=\frac{1}{4}\widehat{IOB}\)
THAY SỐ: \(\frac{1}{4}\widehat{IOB}+\widehat{IOB}=70^0\)
\(\Rightarrow\left(\frac{1}{4}+1\right)\widehat{IOB}=70^0\)
\(\frac{5}{4}\widehat{IOB}=70^0\)
\(\widehat{IOB}=70^0:\frac{5}{4}\)
\(\Rightarrow\widehat{IOB}=56^0\)
MÀ \(\widehat{AOI}=\frac{1}{4}\widehat{IOB}\)
THAY SỐ: \(\widehat{AOI}=\frac{1}{4}.56^0=14^0\)
\(\Rightarrow\widehat{AOI}=14^0\)
CHÚC BN HỌC TỐT!!!!!

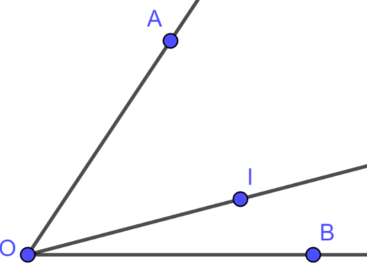
Ta có: \(\widehat{BOI}=\dfrac{1}{4}\cdot\widehat{AOB}\)(gt)
\(\Leftrightarrow\widehat{BOI}=\dfrac{1}{4}\cdot60^0\)
hay \(\widehat{BOI}=15^0\)
Ta có: tia OI nằm giữa hai tia OA và OB(gt)
nên \(\widehat{AOI}+\widehat{BOI}=\widehat{AOB}\)
\(\Leftrightarrow\widehat{AOI}=\widehat{AOB}-\widehat{BOI}=60^0-15^0\)
hay \(\widehat{AOI}=45^0\)
Vậy: \(\widehat{BOI}=15^0\); \(\widehat{AOI}=45^0\)


Ta có: \(\widehat{AOB}\)= 600 và \(\widehat{BOI}\)= \(\frac{1}{4}\)\(\widehat{AOB}\)
\(=>\widehat{BOI}\)= \(\frac{1}{4}.60\)
\(=>\widehat{BOI}\)= 150
Ta lại có: \(\widehat{BOI}\)\(+\widehat{AOI}\)= \(\widehat{AOB}\)
Hay: 150 + \(\widehat{AOI}\)= 600
=> \(\widehat{AOI}\)= 450
Vẽ hình hơi xấu, thông cảm nhé ^^
Hk tốt

\(\widehat{BOI}=\dfrac{1}{5}\cdot60^0=12^0\)
\(\widehat{AOI}=60^0-12^0=48^0\)

\(\widehat{BOI}=\frac{1}{4}\widehat{AOB}=>\widehat{BOI}=\frac{1}{4}\cdot60^O\)\(=>\widehat{BOI}=15^O\)
Vì tia OI nằm giữa hai tia OA và OB
\(=>\widehat{AOI}+\widehat{BOI}=\widehat{AOB}\)
Thay \(\widehat{BOI}=15^o,\widehat{AOB}=60^o\),ta có:
\(\widehat{AOI}+15^o=60^o\)
\(=>\widehat{AOI}=60^o-15^o\)
\(=>\widehat{AOI}=45^o\)
Vậy \(\widehat{BOI}=15^o;\widehat{AOI}=45^o\)
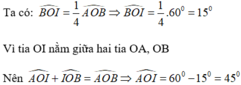
Vì góc AOI = 1/4 IOB và AOB = \(^{70^o}\)
⇒AOI= (70:5).1
⇒AOI= \(14^o\)
Tia OI nằm giữa OA; OB => góc AOI +IOB =AOB
<=> \(\left\{{}\begin{matrix}\widehat{AOI}+\widehat{IOB}=70^0\\\widehat{AOI}=\dfrac{1}{4}.\widehat{IOB}\end{matrix}\right.\) \(\Leftrightarrow\widehat{AOI}+4\widehat{AOI}=70^0\Rightarrow\widehat{AOI}=\dfrac{70^0}{5}=14^0\)