Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: 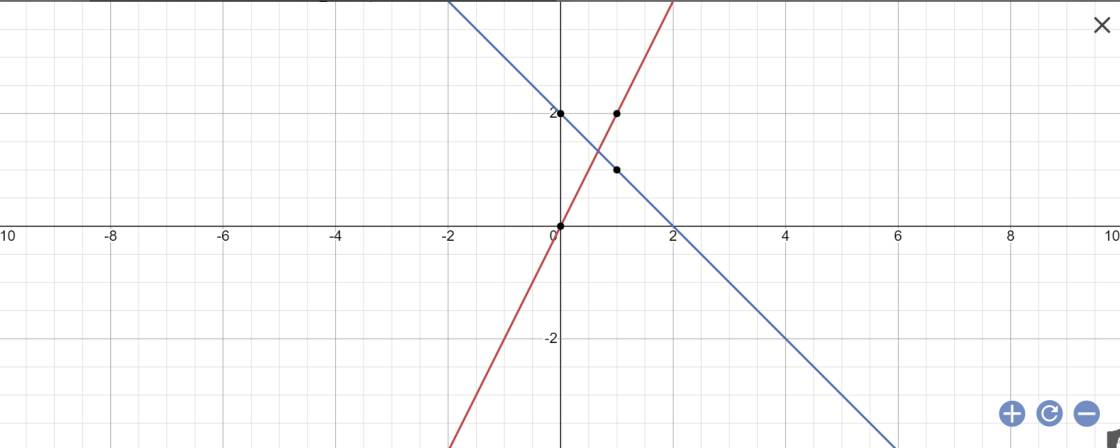
b: Vì (d3)//(d2) nên \(\left\{{}\begin{matrix}a=-1\\b\ne2\end{matrix}\right.\)
Vậy: (d3): y=-x+b
Thay x=1 vào (d1), ta được:
\(y=2\cdot1=2\)
Thay x=1 và y=2 vào y=-x+b, ta được:
\(b-1=2\)
=>b=2+1=3

\(b,\left(d_3\right)\text{//}\left(d_1\right)\Leftrightarrow\left\{{}\begin{matrix}a=1\\b\ne4\end{matrix}\right.\Leftrightarrow\left(d_3\right):y=x+b\)
PT hoành độ giao điểm \(\left(d_2\right);\left(d_3\right)\) là \(x+b=-2x-2\)
Mà 2 đt cắt tại hoành độ \(-3\) nên \(x=-3\)
\(\Leftrightarrow b-3=4\Leftrightarrow b=7\)
Vậy \(\left(d_3\right):y=x+7\)

d3//d2 \(\Rightarrow a=-1\)
d3 cắt d1 tại điểm có hoành độ bằng 1
\(\Rightarrow a+b=2\)
Ta có hệ
\(\left\{{}\begin{matrix}a=-1\\a+b=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=-1\\b=3\end{matrix}\right.\)

b, PT giao điểm (d3) và (d1) là \(\dfrac{1}{3}x+3=2x-2\Leftrightarrow\dfrac{5}{3}x=5\Leftrightarrow x=3\Leftrightarrow y=4\Leftrightarrow A\left(3;4\right)\)
PT giao điểm (d3) và (d2) là \(\dfrac{1}{3}x+3=-\dfrac{4}{3}x-2\Leftrightarrow\dfrac{5}{3}x=-5\Leftrightarrow x=-3\Leftrightarrow y=2\Leftrightarrow B\left(-3;2\right)\)

\(b,\text{PT hoành độ giao điểm: }-2x+5=x-1\Leftrightarrow x=2\Leftrightarrow y=1\Leftrightarrow A\left(2;1\right)\\ \text{Vậy }A\left(2;1\right)\text{ là giao điểm }\left(d_1\right)\text{ và }\left(d_2\right)\\ c,\text{Gọi }\left(d_3\right):y=ax+b\left(a\ne0\right)\text{ là đt cần tìm}\\ \left(d_3\right)\text{//}\left(d_1\right)\text{ và }M\left(-2;1\right)\in\left(d_3\right)\Leftrightarrow\left\{{}\begin{matrix}a=-2\\b\ne5\\-2a+b=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=-2\\b=-1\end{matrix}\right.\\ \Leftrightarrow\left(d_3\right):y=-2x-1\)

Bài 2:
b: Tọa độ A là:
4-2x=x+1 và y=x+1
=>-3x=-3 và y=x+1
=>x=1 và y=2
c: Thay x=1 và y=2 vào (d3), ta được:
2m+3=2
=>2m=-1
=>m=-1/2
Câu 1:
a: \(A=4\sqrt{24}-3\sqrt{54}+5\sqrt{6}-\sqrt{150}\)
\(=4\cdot2\sqrt{6}-3\cdot3\sqrt{6}+5\sqrt{6}-5\sqrt{6}\)
\(=8\sqrt{6}-9\sqrt{6}=-\sqrt{6}\)
b: \(B=\sqrt{14+4\cdot\sqrt{10}}-\dfrac{1}{\sqrt{10}+3}\)
\(=\sqrt{10+2\cdot\sqrt{10}\cdot2+4}-\dfrac{\left(\sqrt{10}-3\right)}{10-9}\)
\(=\sqrt{\left(\sqrt{10}+2\right)^2}-\sqrt{10}+3\)
\(=\sqrt{10}+2-\sqrt{10}+3=5\)
Câu 2:
a:
b: Vì (d3)//(d2) nên \(\left\{{}\begin{matrix}a=-1\\b\ne2\end{matrix}\right.\)
Vậy: (d3): y=-x+b
Thay x=1 vào (d1), ta được:
\(y=2\cdot1=2\)
Thay x=1 và y=2 vào y=-x+b, ta được:
b-1=2
=>b=3
vậy: (d3): y=-x+3