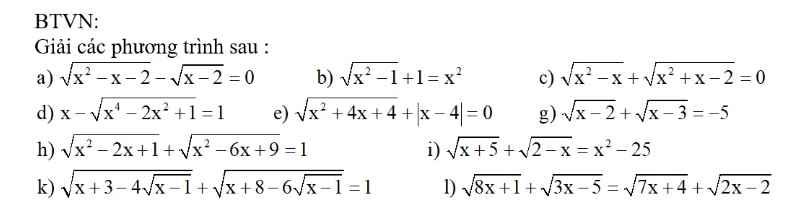Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bài 5:
a: Xét ΔBEC và ΔADC có
\(\widehat{C}\) chung
\(\widehat{EBC}=\widehat{DAC}\)
Do đó: ΔBEC\(\sim\)ΔADC

Bài 7:
a: \(A=x+\sqrt{x}\ge0\forall x\)
Dấu '=' xảy ra khi x=0

a) ĐKXĐ: \(\left\{{}\begin{matrix}x\le-1\\x\ge2\end{matrix}\right.\)
\(\sqrt{x^2-x-2}-\sqrt{x-2}=0\\ \Leftrightarrow\sqrt{x^2-x-2}=\sqrt{x-2}\\ \Leftrightarrow x^2-x-2=x-2\\ \Leftrightarrow x^2-2x=0\\ \Leftrightarrow x\left(x-2\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\left(ktm\right)\\x=2\left(tm\right)\end{matrix}\right.\)
\(a,ĐK:x\ge2\\ PT\Leftrightarrow x^2-x-2=x-2\\ \Leftrightarrow x^2-2x=0\\ \Leftrightarrow\left[{}\begin{matrix}x=2\left(tm\right)\\x=0\left(ktm\right)\end{matrix}\right.\Leftrightarrow x=2\\ b,ĐK:\left[{}\begin{matrix}x\le-1\\x\ge1\end{matrix}\right.\\ PT\Leftrightarrow\sqrt{x^2-1}=x^2-1\\ \Leftrightarrow x^2-1=\left(x^2-1\right)^2\\ \Leftrightarrow\left(x^2-1\right)\left(x^2-1-1\right)=0\\ \Leftrightarrow\left(x-1\right)\left(x+1\right)\left(x-\sqrt{2}\right)\left(x+\sqrt{2}\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=1\left(tm\right)\\x=-1\left(tm\right)\\x=\sqrt{2}\left(tm\right)\\x=-\sqrt{2}\left(tm\right)\end{matrix}\right.\)
\(c,ĐK:\left[{}\begin{matrix}x\le-2\\x\ge1\end{matrix}\right.\\ PT\Leftrightarrow\sqrt{x^2-x}=-\sqrt{x^2+x-2}\\ \Leftrightarrow x^2-x=x^2+x-2\\ \Leftrightarrow2x=2\\ \Leftrightarrow x=1\left(tm\right)\)


8.31:
a: Xét ΔABD có AM/AB=AQ/AD
nên MQ//BD và MQ=BD/2
Xét ΔCBD có CN/CB=CP/CD
nên NP//BD và NP=BD/2
=>MQ//NP và MQ=NP
XétΔBAC có BM/BA=BN/BC
nên MN//AC
=>MN vuông góc BD
=>MN vuông góc MQ
Xét tứ giác MNPQ có
MQ//NP
MQ=NP
góc NMQ=90 độ
=>MNPQ là hình chữ nhật
=>M,N,P,Q cùng nằm trên 1 đường tròn

\(3,\\ A=\dfrac{1}{x^2-4x+9}=\dfrac{1}{\left(x-2\right)^2+5}\)
Vì \(\left(x-2\right)^2+5\ge5\Leftrightarrow A\le\dfrac{1}{5}\)
\(A_{max}=\dfrac{1}{5}\Leftrightarrow x=2\)
\(B=\dfrac{1}{x^2-6x+17}=\dfrac{1}{\left(x-3\right)^2+8}\)
Vì \(\left(x-3\right)^2+8\ge8\Leftrightarrow B\le\dfrac{1}{8}\)
\(B_{max}=\dfrac{1}{8}\Leftrightarrow x=3\)

Bài 3:
\(a,\) Gọi \(\left(d\right):y=ax+b\) là đt cần tìm
\(\Leftrightarrow\left\{{}\begin{matrix}a=2\\0a+b=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=2\\b=1\end{matrix}\right.\Leftrightarrow\left(d\right):y=2x+1\)
\(b,\) PT hoành độ giao điểm:
\(-x^2=2x+1\Leftrightarrow\left(x+1\right)^2=0\Leftrightarrow x=-1\Leftrightarrow y=-1\Leftrightarrow A\left(-1;-1\right)\)
Vậy \(A\left(-1;-1\right)\) là tọa độ giao điểm (P) và (d)
Bài 4:
PT có 2 nghiệm \(\Leftrightarrow\Delta'=16-3m\ge0\Leftrightarrow m\le\dfrac{16}{3}\)
Áp dụng Viét: \(\left\{{}\begin{matrix}x_1+x_2=\dfrac{8}{3}\\x_1x_2=\dfrac{m}{3}\end{matrix}\right.\)
Mà \(x_1^2+x_2^2=\dfrac{82}{9}\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=\dfrac{82}{9}\)
\(\Leftrightarrow\dfrac{64}{9}-\dfrac{2m}{3}=\dfrac{82}{9}\\ \Leftrightarrow\dfrac{2m}{3}=-2\Leftrightarrow m=-3\left(tm\right)\)

\(x^2-x+1-m=0\left(1\right)\\ \text{PT có 2 nghiệm }x_1,x_2\\ \Leftrightarrow\Delta=1-4\left(1-m\right)\ge0\\ \Leftrightarrow4m-3\ge0\Leftrightarrow m\ge\dfrac{3}{4}\\ \text{Vi-ét: }\left\{{}\begin{matrix}x_1+x_2=1\\x_1x_2=1-m\end{matrix}\right.\\ \text{Ta có }5\left(\dfrac{1}{x_1}+\dfrac{1}{x_2}\right)-x_1x_2+4=0\\ \Leftrightarrow5\cdot\dfrac{x_1+x_2}{x_1x_2}-x_1x_2+4=0\\ \Leftrightarrow\dfrac{5}{1-m}+m-1+4=0\\ \Leftrightarrow\dfrac{5}{1-m}+m+3=0\\ \Leftrightarrow5+\left(1-m\right)\left(m+3\right)=0\\ \Leftrightarrow m^2+2m-8=0\\ \Leftrightarrow m^2-2m+4m-8=0\\ \Leftrightarrow\left(m-2\right)\left(m+4\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}m=2\left(n\right)\\m=-4\left(l\right)\end{matrix}\right.\)
Vậy $m=2$
 Ai giúp em với ạ em cần rất gấp bài 4 thôi ạ
Ai giúp em với ạ em cần rất gấp bài 4 thôi ạ