K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
23 tháng 12 2021
c)\(\left\{{}\begin{matrix}u_1+u_3=3\\u_1^2+u_3^2=5\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}u_1+u_3=3\\\left(u_1+u_3\right)^2-2u_1u_3=5\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}u_1+u_3=3\\u_1u_3=2\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}u_1=2\\u_3=1\end{matrix}\right.\\\left\{{}\begin{matrix}u_1=1\\u_3=2\end{matrix}\right.\end{matrix}\right.\)
Làm nốt (sử dụng công thức: \(u_n=u_1+\left(n-1\right)d\) để tìm được công sai
\(S_n=nu_1+\dfrac{n\left(n-1\right)}{2}d\) để tính tổng 15 số hạng đầu)
d)\(\left\{{}\begin{matrix}u_1+u_2+u_3=14\\u_1u_2u_3=64\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}u_2-d+u_2+u_2+d=14\\\left(u_2-d\right)u_2\left(u_2+d\right)=64\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}u_2=\dfrac{14}{3}\\\left(u_2^2-d^2\right)u_2=64\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}\dfrac{14}{3}=u_2=u_1+d\\d=\dfrac{2\sqrt{889}}{21}\end{matrix}\right.\\\left\{{}\begin{matrix}\dfrac{14}{3}=u_1+d\\d=\dfrac{-2\sqrt{889}}{21}\end{matrix}\right.\end{matrix}\right.\)
(Làm nốt,số xấu quá)
e)\(\left\{{}\begin{matrix}u_1+u_2+u_3=7\\u_1^2+u_2^2+u_3^2=21\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}u_1+u_2+u_3=7\\u_1u_2u_3=\dfrac{21-\left(u_1+u_2+u_3\right)^2}{2}=-14\end{matrix}\right.\)
Làm như ý d)
NV
Nguyễn Việt Lâm
Giáo viên
18 tháng 6 2021
\(\Leftrightarrow sin\left(2x+\dfrac{\pi}{3}\right)=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}2x+\dfrac{\pi}{3}=\dfrac{\pi}{6}+k2\pi\\2x+\dfrac{\pi}{3}=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{\pi}{12}+k\pi\\x=\dfrac{\pi}{4}+k\pi\end{matrix}\right.\)
Nghiệm dương nhỏ nhất là \(x=\dfrac{\pi}{4}\approx0.79\)
Đáp án C
HP
8 tháng 9 2021
1.
c, \(sin\left(\dfrac{\pi}{3}-x\right)=-\dfrac{1}{4}\)
\(\Leftrightarrow\left[{}\begin{matrix}\dfrac{\pi}{3}-x=arcsin\left(-\dfrac{1}{4}\right)+k.360^o\\\dfrac{\pi}{3}-x=\pi-arcsin\left(-\dfrac{1}{4}\right)+k.360^o\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{3}-arcsin\left(-\dfrac{1}{4}\right)+k.360^o\\x=-\dfrac{2\pi}{3}+arcsin\left(-\dfrac{1}{4}\right)+k.360^o\end{matrix}\right.\)
d, \(sin4x=\dfrac{2}{3}\)
\(\Leftrightarrow\left[{}\begin{matrix}4x=arcsin\dfrac{2}{3}+k2\pi\\4x=\pi-arcsin\dfrac{2}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{4}arcsin\dfrac{2}{3}+\dfrac{k\pi}{2}\\x=\dfrac{\pi}{4}-\dfrac{1}{4}arcsin\dfrac{2}{3}+\dfrac{k\pi}{2}\end{matrix}\right.\)
HP
8 tháng 9 2021
1.
e, \(2sin2x+\sqrt{2}=0\)
\(\Leftrightarrow sin2x=-\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow sin2x=sin\left(-\dfrac{\pi}{4}\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=-\dfrac{\pi}{4}+k2\pi\\2x=\dfrac{5\pi}{4}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{\pi}{8}+k\pi\\x=\dfrac{5\pi}{8}+k\pi\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
7 tháng 9 2021
Tất cả \(k\in Z\)
1.
a. \(\Leftrightarrow\dfrac{1}{2}sinx+\dfrac{\sqrt{3}}{2}cosx=1\)
\(\Leftrightarrow sin\left(x+\dfrac{\pi}{3}\right)=1\)
\(\Leftrightarrow x+\dfrac{\pi}{3}=\dfrac{\pi}{2}+k2\pi\)
\(\Leftrightarrow x=\dfrac{\pi}{6}+k2\pi\)
Đáp án trong đề bị sai
NV
Nguyễn Việt Lâm
Giáo viên
7 tháng 9 2021
b.
\(\Leftrightarrow\dfrac{1}{2}cos7x-\dfrac{\sqrt{3}}{2}sin7x=-\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow cos\left(7x+\dfrac{\pi}{3}\right)=cos\left(\dfrac{3\pi}{4}\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}7x+\dfrac{\pi}{3}=\dfrac{3\pi}{4}+k2\pi\\7x+\dfrac{\pi}{3}=-\dfrac{3\pi}{4}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}7x=\dfrac{5\pi}{12}+k2\pi\\7x=-\dfrac{13\pi}{12}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5\pi}{84}+\dfrac{k2\pi}{7}\\x=-\dfrac{13\pi}{84}+\dfrac{k2\pi}{7}\end{matrix}\right.\)
Do \(\dfrac{2\pi}{5}\le x\le\dfrac{6\pi}{7}\Rightarrow\left[{}\begin{matrix}\dfrac{2\pi}{5}\le\dfrac{5\pi}{84}+\dfrac{k2\pi}{7}\le\dfrac{6\pi}{7}\\\dfrac{2\pi}{5}\le-\dfrac{13\pi}{84}+\dfrac{k2\pi}{7}\le\dfrac{6\pi}{7}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}\dfrac{143}{120}\le k\le\dfrac{67}{24}\\\dfrac{233}{120}\le k\le\dfrac{85}{24}\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}k=1\\k=\left\{2;3\right\}\end{matrix}\right.\)
\(\Rightarrow x=\left\{\dfrac{53\pi}{84};\dfrac{5\pi}{12};\dfrac{59\pi}{84}\right\}\)
29 tháng 3 2021
\(y'=\left(m-1\right)\cos2x\cdot2-2\cdot\sin x-2m=0\)
\(\Leftrightarrow\left(m-1\right)\left(1-2\sin^2x\right)-\sin x-m=0\)
\(\Leftrightarrow2\left(1-m\right)\sin^2x-\sin x-1=0\)
bạn tự làm nốt nha
GM
1
AW
2
NV
Nguyễn Việt Lâm
Giáo viên
20 tháng 3 2022
17.
Gọi số vi khuẩn ban đầu là x
Sau 5 phút số vi khuẩn là: \(x.2^5=64000\Rightarrow x=2000\)
Sau k phút:
\(2000.2^k=2048000\Rightarrow2^k=1024=2^{10}\)
\(\Rightarrow k=10\)
NV
Nguyễn Việt Lâm
Giáo viên
20 tháng 3 2022
18.
\(S_{2019}=\left(\dfrac{1}{2}\right)^1+1+\left(\dfrac{1}{2}\right)^2+1+...+\left(\dfrac{1}{2}\right)^{2019}+1\)
\(=\left(\dfrac{1}{2}\right)^1+\left(\dfrac{1}{2}\right)^2+...+\left(\dfrac{1}{2}\right)^{2019}+2019\)
Xét \(S=\left(\dfrac{1}{2}\right)^1+\left(\dfrac{1}{2}\right)^2+...+\left(\dfrac{1}{2}\right)^{2019}\) là tổng cấp số nhân với \(\left\{{}\begin{matrix}u_1=\dfrac{1}{2}\\q=\dfrac{1}{2}\\n=2019\end{matrix}\right.\)
\(\Rightarrow S=\dfrac{1}{2}.\dfrac{\left(\dfrac{1}{2}\right)^{2019}-1}{\dfrac{1}{2}-1}=1-\dfrac{1}{2^{2019}}\)
\(\Rightarrow S_{2020}=2019+S=2020-\dfrac{1}{2^{2019}}\)
19. C là khẳng định sai, ví dụ: \(u_n=2\) ; \(v_n=-\dfrac{1}{n}\)
PA
1
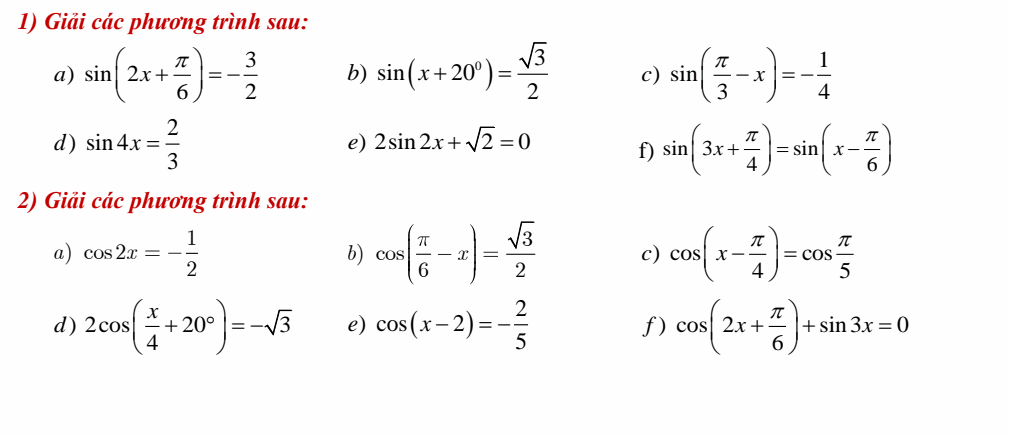
\(\left\{{}\begin{matrix}SO\perp BC\\SO\perp CA\end{matrix}\right.\) \(\Rightarrow SO\perp\left(ABC\right)\)
\(AA'=\dfrac{a\sqrt{3}}{2}\) (trung tuyến tam giác đều) \(\Rightarrow AO=\dfrac{2}{3}AA'=\dfrac{a\sqrt{3}}{3}\)
\(\Rightarrow M\) nằm trên đoạn thẳng OA'
Qua M kẻ đường thẳng song song BC cắt AB và AC lần lượt tại D và E
Trong mp (SAA'), qua M kẻ đường thẳng song song SO cắt SA' tại F
Trong mp (SBC), qua F kẻ đường thẳng song song BC cắt SB và SC lần lượt tại G và H
\(\Rightarrow\) Hình thang DEHG là thiết diện của (P) và chóp
\(FM||SO\Rightarrow FM\perp\left(ABC\right)\Rightarrow FM\perp ED\)
Áp dụng định lý Talet cho tam giác ABC:
\(\dfrac{DE}{BC}=\dfrac{AM}{AA'}\Rightarrow DE=\dfrac{BC.AM}{AA'}=\dfrac{a.x}{\dfrac{a\sqrt{3}}{2}}=\dfrac{2x\sqrt{3}}{3}\)
Talet tam giác SOA':
\(\dfrac{FM}{SO}=\dfrac{MA'}{OA'}\Rightarrow FM=\dfrac{SO.MA'}{OA'}=\dfrac{2a.\left(\dfrac{a\sqrt{3}}{2}-x\right)}{\dfrac{a\sqrt{3}}{6}}=6a-4\sqrt{3}x\)
Talet tam giác SBC:
\(\dfrac{GH}{BC}=\dfrac{SF}{SA'}=1-\dfrac{FA'}{SA'}=1-\dfrac{FM}{SO}=1-\dfrac{6a-4\sqrt{3}x}{2a}=\dfrac{2\sqrt{3}x-2a}{a}\)
\(\Rightarrow GH=2\sqrt{3}x-2a\)
\(S_{DEHG}=\dfrac{1}{2}\left(DE+GH\right).FM=\dfrac{1}{2}\left(\dfrac{2x\sqrt{3}}{3}+2\sqrt{3}x-2a\right)\left(6a-4\sqrt{3}x\right)\)
\(=\dfrac{1}{3}\left(4\sqrt{3}x-3a\right)\left(6a-4\sqrt{3}x\right)\le\dfrac{1}{12}\left(4\sqrt{3}x-3a+6a-4\sqrt{3}x\right)^2=\dfrac{9a^2}{12}\)
Dấu "=" xảy ra khi \(4\sqrt{3}x-3a=6a-4\sqrt{3}x\Leftrightarrow x=\dfrac{9a}{8\sqrt{3}}=\dfrac{3a\sqrt{3}}{8}\)