 giúp mình nhé cảm ơn :((
giúp mình nhé cảm ơn :((
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a.
\(\Leftrightarrow\left\{{}\begin{matrix}\left(2x-3y\right)\left(2x-y\right)=0\\6x^2+7xy-5y^2=0\end{matrix}\right.\)
TH1: \(2x-3y=0\Rightarrow y=\dfrac{2}{3}x\) thay vào pt dưới
\(\Rightarrow6x^2+7x.\left(\dfrac{2}{3}x\right)-5\left(\dfrac{2}{3}x\right)^2=0\)
\(\Leftrightarrow\dfrac{76}{9}x^2=0\Rightarrow x=0\Rightarrow y=0\)
TH2: \(2x-y=0\Rightarrow y=2x\)
Tương tự ta cũng được \(x=0;y=0\)
Vậy hệ có nghiệm duy nhất \(\left(x;y\right)=\left(0;0\right)\)
b.
\(\Leftrightarrow\left\{{}\begin{matrix}13x^2-39xy+13y^2=-13\\2x^2+xy+3y^2=13\end{matrix}\right.\)
Cộng vế với vế
\(\Rightarrow15x^2-38xy+16y^2=0\)
\(\Leftrightarrow\left(x-2y\right)\left(15x-8y\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=2y\\x=\dfrac{8}{15}y\end{matrix}\right.\)
Thay vào pt đầu:
- Với \(x=2y\Rightarrow4y^2-6y^2+y^2=-1\)
\(\Rightarrow y^2=1\Rightarrow\left[{}\begin{matrix}y=1\Rightarrow x=2\\y=-1\Rightarrow x=-2\end{matrix}\right.\)
- Với \(x=\dfrac{8}{15}y\)
\(\Rightarrow\left(\dfrac{8}{15}y\right)^2-3\left(\dfrac{8}{15}y\right).y+y^2=-1\)
\(\Leftrightarrow-\dfrac{71}{225}y^2=-1\Rightarrow y^2=\dfrac{225}{71}\)
\(\Rightarrow\left[{}\begin{matrix}y=\dfrac{15}{\sqrt{71}}\Rightarrow x=\dfrac{8}{\sqrt{71}}\\y=-\dfrac{15}{\sqrt{71}}\Rightarrow x=-\dfrac{8}{\sqrt{71}}\end{matrix}\right.\)

Gọi số cần tìm là x
Theo đề, ta có: \(\dfrac{-9}{5}< \dfrac{-7}{x}< \dfrac{-9}{6}\)
=>\(\dfrac{9}{5}>\dfrac{7}{x}>\dfrac{9}{6}\)
=>\(\dfrac{63}{35}>\dfrac{63}{9x}>\dfrac{63}{42}\)
=>35<9x<42
=>9x=36
=>x=4
Gọi mẫu số của phân số cần tìm là \(x\) ( 0 ≠ \(x\) \(\in\) Z)
\(\dfrac{-9}{5}\) < \(\dfrac{-7}{x}\) < \(\dfrac{-9}{6}\)
⇒ \(\dfrac{-9.\left(-1\right)}{5.}\) > \(\dfrac{-7.\left(-1\right)}{x}\) > \(\dfrac{-9.\left(-1\right)}{6}\) (nhân cả hai vế của bất đẳng thức với một số âm thì bất đẳng thức đổi chiều)
⇒\(\dfrac{9}{5}\) > \(\dfrac{7}{x}\) > \(\dfrac{9}{6}\)
⇒ \(\dfrac{9.7}{5.7}\) > \(\dfrac{7.9}{x.9}\) > \(\dfrac{9.7}{6.7}\)
⇒ \(\dfrac{63}{35}\) > \(\dfrac{63}{9x}\) > \(\dfrac{63}{42}\)
⇒ 35 < 9\(x\) < 42
\(\dfrac{35}{9}\) < \(x\) < \(\dfrac{42}{9}\)
3\(\dfrac{8}{9}\) < \(x\) < 4\(\dfrac{6}{9}\)
Vậy \(x=4\)

1: \(3^{x+2}+3^x=10\)
=>\(9\cdot3^x+3^x=10\)
=>\(10\cdot3^x=10\)
=>\(3^x=1\)
=>x=0
2: \(2^{x+1}-2^x=32\)
=>\(2^x\cdot2-2^x=32\)
=>\(2^x=32=2^5\)
=>x=5
3: \(4^{x+2}-4^x=60\)
=>\(4^x\cdot16-4^x=60\)
=>\(15\cdot4^x=15\cdot4\)
=>\(4^x=4\)
=>x=1
4: \(2^{x+2}-2^x=96\)
=>\(4\cdot2^x-2^x=96\)
=>\(3\cdot2^x=3\cdot32\)
=>\(2^x=32\)
=>x=5
5: \(2^{x+3}+2^x=144\)
=>\(2^x\cdot8+2^x=144\)
=>\(9\cdot2^x=9\cdot16\)
=>\(2^x=16\)
=>x=4
6: \(3^{x+3}-3^x=234\)
=>\(3^x\cdot27-3^x=234\)
=>\(26\cdot3^x=234\)
=>\(3^x=9=3^2\)
=>x=2
7:
\(5^x+5^{x+1}=750\)
=>\(5^x+5\cdot5^x=750\)
=>\(6\cdot5^x=750\)
=>\(5^x=125=5^3\)
=>x=3
8: \(2^x+2^{x+2}=320\)
=>\(2^x+2^x\cdot4=320\)
=>\(5\cdot2^x=320\)
=>\(2^x=64=2^6\)
=>x=6
9: \(5^x+5^{x+2}=650\)
=>\(5^x+5^x\cdot25=650\)
=>\(26\cdot5^x=650\)
=>\(5^x=\dfrac{650}{26}=25=5^2\)
=>x=2

a: \(x^3=64\)
=>\(x^3=4^3\)
=>x=4
b: \(x^2=2^3+3^2+4^3\)
=>\(x^2=8+9+64=64+17=81\)
mà x>0
nên \(x=\sqrt{81}=9\)
c: \(3x^2+123=231\)
=>\(3x^2=231-123=108\)
=>\(x^2=36\)
mà x>0
nên x=6
d: \(145-2x^2=136:8\)
=>\(145-2x^2=17\)
=>\(2x^2=128\)
=>\(x^2=64\)
mà x>0
nên x=8


a: \(5\cdot5^2\cdot5^4\cdot5^8=5^{1+2+4+8}=5^{15}\)
b: \(2^3\cdot2^4\cdot2^5:2^6=2^{3+4+5-6}=2^6\)
c: \(x^2\cdot x^3:x^4\cdot x^7=x^{2+3-4+7}=x^8\)
d: \(\left(7^3:7^2\right)\cdot\left(7^2\cdot7^4\right):\left(7^2\cdot7\right)\)
\(=7\cdot7^6:7^3\)
\(=7^7:7^3=7^4\)

Bài tập về nhà bạn tự làm đi chớ, lúc câu hỏi nào mà khó quá á thì hỏi, còn cái này bài tập về nhà, của ai nấy làm
1. He has a son, Tan
ð Tan is his son
2. Mrs.Mai and Mr.Hung have a daughter, Linh
ð Mrs.Mai and Mr.Hung are Linh's parents
3. Hoa is Mrs.Lan’s niece
ð Mrs.Lan is Hoa's aunt
4. I have a daughter, Jane
ð Jane is........my daughter.........
5. Long is Khue’s brother
ð Khue..is Long's brother...........

Thể tích nước buổi sáng bơm vào là:
\(2\cdot1,6\cdot1,5\cdot80\%=3,2\cdot1,2=3,84\left(m^3\right)=3840\left(lít\right)\)
Lượng nước còn lại sau khi dùng là:
\(3840\left(1-80\%\right)=768\left(lít\right)\)
2m=20dm; 1,6m=16dm; 1,5m=15dm
Thể tích tối đa của bể là: \(20\cdot16\cdot15=300\cdot16=4800\left(lít\right)\)
Lượng nước cần bơm vào là:
4800-768=4032(lít)

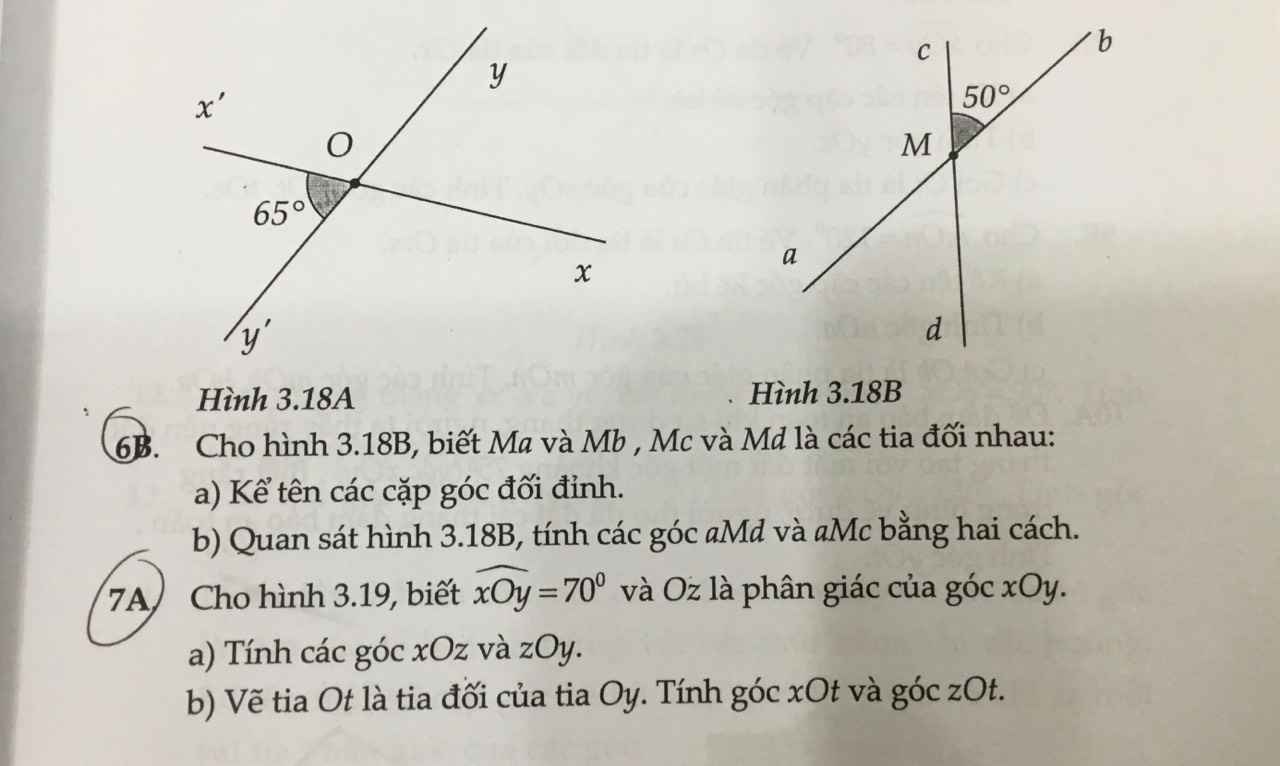
6B:
a: Các cặp góc đối đỉnh là: \(\widehat{cMb};\widehat{aMd}\); \(\widehat{aMc};\widehat{bMd}\)
b:
Cách 1: \(\widehat{aMc}+\widehat{cMb}=180^0\)(hai góc kề bù)
=>\(\widehat{aMc}=180^0-50^0=130^0\)
Ta có: \(\widehat{aMc}+\widehat{aMd}=180^0\)(hai góc kề bù)
=>\(\widehat{aMd}=180^0-130^0=50^0\)
Cách 2:
Ta có: \(\widehat{aMd}=\widehat{cMb}\)(hai góc đối đỉnh)
mà \(\widehat{cMb}=50^0\)
nên \(\widehat{aMd}=50^0\)
Ta có: \(\widehat{aMd}+\widehat{aMc}=180^0\)(hai góc kề bù)
=>\(\widehat{aMc}+50^0=180^0\)
=>\(\widehat{aMc}=130^0\)
7A:
a: Oz là phân giác của góc xOy
=>\(\widehat{xOz}=\widehat{zOy}=\dfrac{\widehat{xOy}}{2}=35^0\)
b: Ta có: \(\widehat{xOy}+\widehat{xOt}=180^0\)(hai góc kề bù)
=>\(\widehat{xOt}+70^0=180^0\)
=>\(\widehat{xOt}=110^0\)
Ta có: \(\widehat{zOt}+\widehat{zOy}=180^0\)(hai góc kề bù)
=>\(\widehat{zOt}+35^0=180^0\)
=>\(\widehat{zOt}=145^0\)