với x,y là các số dương thỏa mãn điều kiện x>=2y tìm giá trị nhỏ nhất của M=\(\frac{x^2+y^2}{xy}\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NT
1
DD
Đoàn Đức Hà
Giáo viên
23 tháng 5 2021
\(x\ge2y\Rightarrow x-y\ge y\Rightarrow x\left(x-y\right)\ge2y^2\Rightarrow x^2-xy-2y^2\ge0\).
\(\left(x-2y\right)^2\ge0\Leftrightarrow x^2-4xy+4y^2\ge0\)
\(\Rightarrow\left(x^2-xy-2y^2\right)+\left(x^2-4xy+4y^2\right)\ge0\)
\(\Leftrightarrow x^2+y^2\ge\frac{5}{2}xy\)
\(A=\frac{x^2+y^2}{xy}\ge\frac{\frac{5}{2}xy}{xy}=\frac{5}{2}\)
Dấu \(=\)xảy ra khi \(x=2y>0\).
1 tháng 6 2015
sorry lam lon
M=(x^2+y^2/xy=x^2/xy+y^2/xy=x^2/4xy +x^2/4xy +x^2/4xy+x^2/4xy + 4y^2/4xy
Do x,y > 0 nên áp dụng cô si cho 5 số dương ta có :
M ≥ 5 . Căn 5 của (x^2/4xy . x^2/4xy .x^2/4xy.4y^2/4xy)=5.căn 5 của (x^3/256y^3) (*)
Mặt khác do x ≥ 2y =>x^3 ≥ 8y^3 nên từ (*) ta có :
M ≥ 5.can 5 cua (8y^3/256y^3)=5.can 5 cua (1/32)=5.1/2 =5/2
Dau " ≥ " khi
{x^2/4xy = 4y^2/4xy
{x^3=8y^3
=>x ≥ 2y
Vậy :x ≥ 2y
T
15 tháng 3 2021
Ta có:
\(2\left(2x^2+xy+2y^2\right)=3\left(x^2+y^2\right)+\left(x+y\right)^2\ge\dfrac{3}{2}\left(x+y\right)^2+1\left(x+y\right)^2=\dfrac{5}{2}\left(x+y\right)^2\)
\(\Rightarrow\sqrt{2x^2+xy+2y^2}\ge\dfrac{\sqrt{5}}{2}\left(x+y\right)\)
Gợi ý. Dùng cái trên.
20 tháng 9 2019
\(P=\sqrt{\frac{1}{36}\left(11a+7b\right)^2+\frac{59\left(a-b\right)^2}{36}}+\sqrt{\frac{1}{36}\left(7a+11b\right)+\frac{59\left(a-b\right)^2}{36}}\)
\(=\sqrt{\frac{1}{16}\left(3a+5b\right)^2+\frac{5\left(a-b\right)^2}{16}}+\sqrt{\frac{1}{16}\left(5a+3b\right)^2+\frac{5\left(a-b\right)^2}{16}}\)
\(\ge\frac{1}{6}\left(11a+7b\right)+\frac{1}{6}\left(7a+11b\right)+\frac{1}{4}\left(3a+5b\right)+\frac{1}{4}\left(5a+3b\right)\)
\(=5\left(a+b\right)=5.2016=10080\)
CM
9 tháng 4 2018
Ta có
P = x 2 4 + 8 y + y 2 1 + x = x 2 4 + 8 y + 2 y 2 4 + 4 x ≥ x + 2 y 2 8 + 4 x + 2 y
Dấu “=” xảy ra khi x = 2y
Đặt t = x + 2y; t ≥ 8 . Khi đó P ≥ t 2 8 + 4 t
Xét hàm số f t = t 2 8 + 4 t , t ∈ [ 8 ; + ∞ )
Suy ra f(t) đồng biến trên [ 8 ; + ∞ ) nên f t ≥ f 8 = 8 5 Vậy m a x P = 8 5 ⇔ x = 4 ; y = 2
Đáp án A
CM
26 tháng 4 2018
Đáp án B.
Từ giả thiết, suy ra
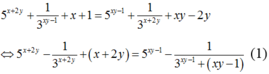
Xét hàm số f ( t ) = 5 t - 1 3 t + t trên ℝ .
Đạo hàm f ' ( t ) = 5 t . ln 5 - ln 3 3 t + 1 > 0 , ∀ t ∈ ℝ ⇒ hàm số f ( t ) luôn đồng biến trên ℝ .
Suy ra
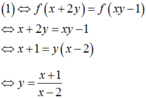
Do y > 0 nên x + 1 x - 2 > 0 ⇔ [ x > 2 x < - 1 . Mà x > 0 nên x > 2 .
Từ đó T = x + y = x + x + 1 x - 2 . Xét hàm số g ( x ) = x + x + 1 x - 2 trên 2 ; + ∞ .
Đạo hàm
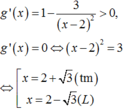
Lập bảng biến thiên của hàm số trên 2 ; + ∞ , ta thấy min g ( x ) = g ( 2 + 3 ) = 3 + 2 3 .
Vậy T m i n = 3 + 2 3 khi x = 2 + 3 và x = 1 + 3 .
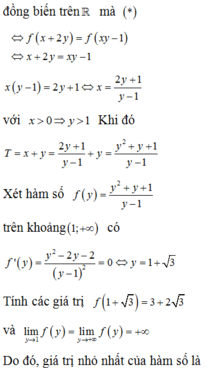
\(M=\frac{x^2}{xy}+\frac{y^2}{xy}=\frac{x}{y}+\frac{y}{x}\)
\(x\ge2y\Rightarrow\frac{x}{y}\ge2;\frac{y}{x}\ge\frac{1}{2}\)
\(\Rightarrow M\ge2+\frac{1}{2}=\frac{5}{2}\)
\(\text{Dấu "=" xảy ra khi x=1;y=}\frac{1}{2}\)
\(\text{Vậy....}\)