mọi người ơi giúp em bài này với ạ, e đang cần gấp, tks mọi người nhiều
K
Khách
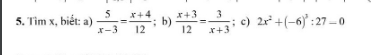
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
13 tháng 10 2021
đúng rồi ạ, giúp em với ạ hoặc làm đc câu nào giúp em cũng được ạ
HK
1
KA
1
12 tháng 8 2021
nSO3=8/80=0,1(mol)
pthh: SO3 + H2O -> H2SO4
nH2SO4=nSO3=0,1(mol) => mH2SO4(tạo sau)= 0,1.98=9,8(g)
mH2SO4(tổng)= 100.9,8% + 9,8=19,6(g)
mddH2SO4(sau)=8+100=108(g)
=>C%ddH2SO4(sau)= (19,6/108).100=18,148%
PA
1
17 tháng 7 2021
1) Áp dụng định lí Pytago vào ΔABC vuông tại A, ta được:
\(BC^2=AB^2+AC^2\)
\(\Leftrightarrow BC^2=6^2+8^2=100\)
hay BC=10(cm)
Áp dụng hệ thức lượng trong tam giác vuông vào ΔABC vuông tại A có AH là đường cao ứng với cạnh huyền BC, ta được:
\(AH\cdot BC=AB\cdot AC\)
\(\Leftrightarrow AH\cdot10=6\cdot8=48\)
hay AH=4,8(cm)
HK
1
TH
0
MA
2
30 tháng 9 2021
\(5,\\ a,=x^4+4x^2+4-4x^2=\left(x^2+2\right)^2-4x^2=\left(x^2-2x+2\right)\left(x^2+2x+2\right)\\ b,=x^4+16x^2+64-16x^2=\left(x^2+8\right)^2-16x^2=\left(x^2-4x+8\right)\left(x^2+4x+8\right)\\ c,=x^8+x^7+x^6-x^6+x^5-x^5+x^4-x^4+x^3-x^3+x^2-x^2+x-x+1\\ =x^6\left(x^2+x+1\right)-x^4\left(x^2+x+1\right)+x^3\left(x^2+x+1\right)-x\left(x^2+x+1\right)+\left(x^2+x+1\right)\\ =\left(x^6-x^4+x^3-x+1\right)\left(x^2+x+1\right)\)
30 tháng 9 2021
\(d,=x^8+2x^4+1-x^4=\left(x^4+1\right)^2-x^4=\left(x^4-x^2+1\right)\left(x^4+x^2+1\right)\\ =\left(x^4-x^2+1\right)\left(x^4+2x^2+1-x^2\right)\\ =\left(x^4-x^2+1\right)\left(x^2-x+1\right)\left(x^2+x+1\right)\\ e,=x^5+x^4-x^4+x^3-x^3+x^2-x^2+x+1\\ =x^3\left(x^2+x+1\right)-x^2\left(x^2+x+x\right)+\left(x^2+x+1\right)\\ =\left(x^3-x^2+1\right)\left(x^2+x+1\right)\\ f,=x^3+2x^2-x^2-2x+2x+4\\ =\left(x+2\right)\left(x^2-x+2\right)\\ g,=x^4+2x^2+1-25=\left(x^2+1\right)^2-25\\ =\left(x^2+1-5\right)\left(x^2-1-5\right)=\left(x^2-4\right)\left(x^2-6\right)=\left(x-2\right)\left(x+2\right)\left(x^2-6\right)\)
\(h,=x^3-2x^2+2x^2-4x+2x-4=\left(x-2\right)\left(x^2+2x+2\right)\\ i,=a^4-4a^2b^2+4b^4-4a^2b^2=\left(a^2-2b^2\right)^2-4a^2b^2\\ =\left(a^2-2ab-2b^2\right)\left(a^2+2ab-2b^2\right)\)
a) \(\Rightarrow\left(x-3\right)\left(x+4\right)=5.12\)
\(\Rightarrow x^2+x-72=0\)
\(\Rightarrow\left(x-8\right)\left(x+9\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=8\\x=-9\end{matrix}\right.\)
b) \(\Rightarrow\left(x+3\right)^2=36\)
\(\Rightarrow\left[{}\begin{matrix}x+3=6\\x+3=-6\end{matrix}\right.\)\(\Rightarrow\left[{}\begin{matrix}x=3\\x=-9\end{matrix}\right.\)
c) \(\Rightarrow2x^2=8\Rightarrow x^2=4\)
\(\Rightarrow\left[{}\begin{matrix}x=2\\x=-2\end{matrix}\right.\)
em cảm ơn nhiều ạ!