A = cos2x. cot2x + 3cos2x - cot2x - 2sin2x
Chứng minh biểu thức sau không phụ thuộc vào x.
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Chọn A.
Ta có: A = cos2x.cot2x + 3cos2x - cot2x + 2sin2x
=( cos2x.cot2x - cot2x) + (2sin2x + 2cos2x) + cos2x
= cot2x( cos2x - 1) + 2 + cos2x
= - cot2x. sin2x + 2 + cos2x
= -cos2x + 2 + cos2x = 2

a: \(VT=\dfrac{cot^2x}{1+cot^2x}\cdot\dfrac{1+tan^2x}{tan^2x}\)
\(=\dfrac{cot^2x}{\dfrac{1}{sin^2x}}\cdot\dfrac{\dfrac{1}{cos^2x}}{tan^2x}\)
\(=\dfrac{cot^2x}{tan^2x}\cdot\dfrac{1}{cos^2x}:\dfrac{1}{sin^2x}\)
\(=\dfrac{cot^2x}{tan^2x}\cdot\dfrac{sin^2x}{cos^2x}\)
\(=cot^2x\)
\(VP=\dfrac{tan^2x+cot^2x}{1+tan^4x}=\dfrac{\dfrac{sin^2x}{cos^2x}+\dfrac{cos^2x}{sin^2x}}{1+\dfrac{sin^4x}{cos^4x}}\)
\(=\dfrac{sin^4x+cos^4x}{sin^2x\cdot cos^2x}:\dfrac{cos^4x+sin^4x}{cos^4x}\)
\(=\dfrac{sin^4x+cos^4x}{sin^2x\cdot cos^2x}\cdot\dfrac{cos^4x}{cos^4x+sin^4x}=\dfrac{cos^2x}{sin^2x}=cot^2x\)
=>VT=VP
b:
\(\dfrac{tan^2x-cos^2x}{sin^2x}+\dfrac{cot^2x-sin^2x}{cos^2x}\)
\(=\dfrac{\left(\dfrac{sinx}{cosx}\right)^2-cos^2x}{sin^2x}+\dfrac{\left(\dfrac{cosx}{sinx}\right)^2-sin^2x}{cos^2x}\)
\(=\dfrac{sin^2x-cos^4x}{cos^2x\cdot sin^2x}+\dfrac{cos^2x-sin^4x}{sin^2x\cdot cos^2x}\)
\(=\dfrac{sin^2x+cos^2x-cos^4x-sin^4x}{cos^2x\cdot sin^2x}\)
\(=\dfrac{1-\left(cos^2x+sin^2x\right)^2+2\cdot cos^2x\cdot sin^2x}{cos^2x\cdot sin^2x}\)
\(=\dfrac{2\cdot cos^2x\cdot sin^2x}{cos^2x\cdot sin^2x}=2\)

A = cos 6 x + 3 sin 2 x . cos 2 x + 2 sin 4 α . cos 2 x + sin 4 α
= cos 6 x + 3.(1 - cos 2 x ) cos 4 x + 2 sin 4 α . cos 2 x + sin 4 α
= cos 6 x + 3 cos 4 x - 3 cos 6 x + 2 sin 4 α - 2 sin 6 x + sin 4 α
= -2.( cos 6 x + sin 6 x ) + 3 cos 4 x + 3 sin 4 α
= -2.( cos 6 x + sin 6 x ) + 3.( cos 4 x + sin 4 α ) = 1
Vậy biểu thức A không phụ thuộc vào x.

\(\frac{1}{sin2a}=\frac{sina}{sina.sin2a}=\frac{sin\left(2a-a\right)}{sina.sin2a}=\frac{sin2a.cosa-cos2a.sina}{sina.sin2a}\)
\(=\frac{sin2a.cosa}{sina.sin2a}-\frac{cos2a.sina}{sina.cos2a}=\frac{cosa}{sina}-\frac{cos2a}{sin2a}=cota-cot2a\)
Áp dụng vào bài toán:
\(A=\frac{1}{sin2y}+\frac{1}{sin2\left(2y\right)}+\frac{1}{sin2\left(4y\right)}-coty+cot8y\)
\(=coty-cot2y+cot2y-cot4y+cot4y-cot8y-coty+cot8y\)
\(=0\)
\(B=\frac{1}{sin2\left(2x\right)}+\frac{1}{sin2\left(2x\right)}+\frac{1}{sin2\left(8x\right)}-cot2x+cot16x\)
\(=cot2x-cot4x+cot4x-cot8x+cot8x-cot16x-cot2x+cot16x\)
\(=0\)

Đáp án: C
Ta có:
A = (1 - sin 2 x ) c o t 2 x + (1 - c o t 2 x ) = c o t 2 x - sin 2 x . c o t 2 x + 1 - c o t 2 x
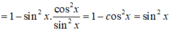

Chọn A.
Ta có C = (1-sin2x) cot2x + 1 - cot2x.
= (1 - sin2x - 1) cot2x + 1
= -sin2x.cot2x + 1 = -cos2x + 1 = sin2x.
bạn ơi hình như chỗ -2sin^2x phải là +2sin^2x thì phải
nếu đúng là +2sin^2x thì biểu thức =2