Giúp em bài 2 và bài 3 này với ạ em đang cần gấp!
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



1 Having slept
2 not being invited
3 Having had
4 having
5 talking
6 succeeded - launching
7 Having travelled
8 Have - considered - trying
9 Having seen - had - to go
10 Being invited
11 Being found
12 having
13 taken - being photographed
14 to fix
15 living
16 Having waited - to deliver - decided to cancel
17 Having photocopied
18 to have happen
19 to give
20 spoiling

a) \(\left\{{}\begin{matrix}2x-7>0.\\5x+1>0.\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}2x>7.\\5x>-1.\end{matrix}\right.\) \(\left\{{}\begin{matrix}x>\dfrac{7}{2}.\\x>\dfrac{-1}{5}.\end{matrix}\right.\)
\(\Rightarrow x>\dfrac{7}{2}.\) \(\Rightarrow x\in\left(\dfrac{7}{2};+\infty\right).\)
Kết luận: Tập nghiệm của hệ bất phương trình trên là \(x\in\left(\dfrac{7}{2};+\infty\right).\)
b) \(\left\{{}\begin{matrix}\left(2x+3\right)\left(x-1\right)>0.\\7x-5< 0.\end{matrix}\right.\) \(\Leftrightarrow\text{}\text{}\)\(\left\{{}\begin{matrix}\left(2x+3\right)\left(x-1\right)>0.\left(1\right)\\x< \dfrac{5}{7}.\left(2\right)\end{matrix}\right.\)
Xét (1):
\(2x+3=0.\Leftrightarrow x=\dfrac{-3}{2}.\\ x-1=0.\Leftrightarrow x=1.\)
Bảng xét dấu:
\(x\) \(-\infty\) \(\dfrac{-3}{2}\) \(1\) \(+\infty\)
\(2x+3\) - \(0\) + | +
\(x-1\) - | - \(0\) +
\(\left(2x+3\right)\left(x-1\right)\) + \(0\) - \(0\) +
Vậy \(\left(2x+3\right)\left(x-1\right)>0.\Leftrightarrow\dfrac{-3}{2}< x< 1.\)
Kết hợp với (2).
\(\Rightarrow\) \(\dfrac{-3}{2}< x< \dfrac{5}{7}.\)
\(\Rightarrow x\in\left(\dfrac{-3}{2};\dfrac{5}{7}\right).\)
Kết luận: Tập nghiệm của hệ bất phương trình trên là \(x\in\left(\dfrac{-3}{2};\dfrac{5}{7}\right).\)

a) \(\dfrac{A}{x-2}=\dfrac{x^2+3x+2}{x^2-4}\)
\(\Leftrightarrow\dfrac{A}{x-2}=\dfrac{\left(x+2\right)\left(x+1\right)}{\left(x-2\right)\left(x+2\right)}\)
\(\Leftrightarrow\dfrac{A}{x-2}=\dfrac{x+1}{x-2}\Leftrightarrow A=x+1\)
b) \(\dfrac{M}{x-1}=\dfrac{x^2+3x+2}{x+1}\)
\(\Leftrightarrow\dfrac{M}{x-1}=\dfrac{\left(x+1\right)\left(x+2\right)}{x+1}\)
\(\Leftrightarrow\dfrac{M}{x-1}=x+2\Leftrightarrow M=\left(x-1\right)\left(x+2\right)=x^2+x-2\)

3) Ta có: \(\text{Δ}=\left[-2\left(m-1\right)\right]^2-4\cdot1\cdot\left(m^2-6\right)\)
\(=\left(2m-2\right)^2-4\left(m^2-6\right)\)
\(=4m^2-8m+4-4m^2+24\)
\(=-8m+28\)
Để phương trình có hai nghiệm phân biệt x1;x2 thì Δ>0
\(\Leftrightarrow-8m+28>0\)
\(\Leftrightarrow-8m>-28\)
hay \(m< \dfrac{7}{2}\)
Áp dụng hệ thức Vi-et, ta được:
\(\left\{{}\begin{matrix}x_1+x_2=\dfrac{2\left(m-1\right)}{1}=2m-2\\x_1x_2=m^2-6\end{matrix}\right.\)
Ta có: \(x_1^2+x_2^2=16\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=16\)
\(\Leftrightarrow\left(2m-2\right)^2-2\left(m^2-6\right)-16=0\)
\(\Leftrightarrow4m^2-8m+4-2m^2+12-16=0\)
\(\Leftrightarrow2m^2-8m=0\)
\(\Leftrightarrow2m\left(m-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m=0\left(nhận\right)\\m=4\left(loại\right)\end{matrix}\right.\)
 giúp em 3 bài này với ạ em đang cần gấp chiều em hc ròi ạ ai làm đc bài nào thì gửi luôn giúp em ạ
giúp em 3 bài này với ạ em đang cần gấp chiều em hc ròi ạ ai làm đc bài nào thì gửi luôn giúp em ạ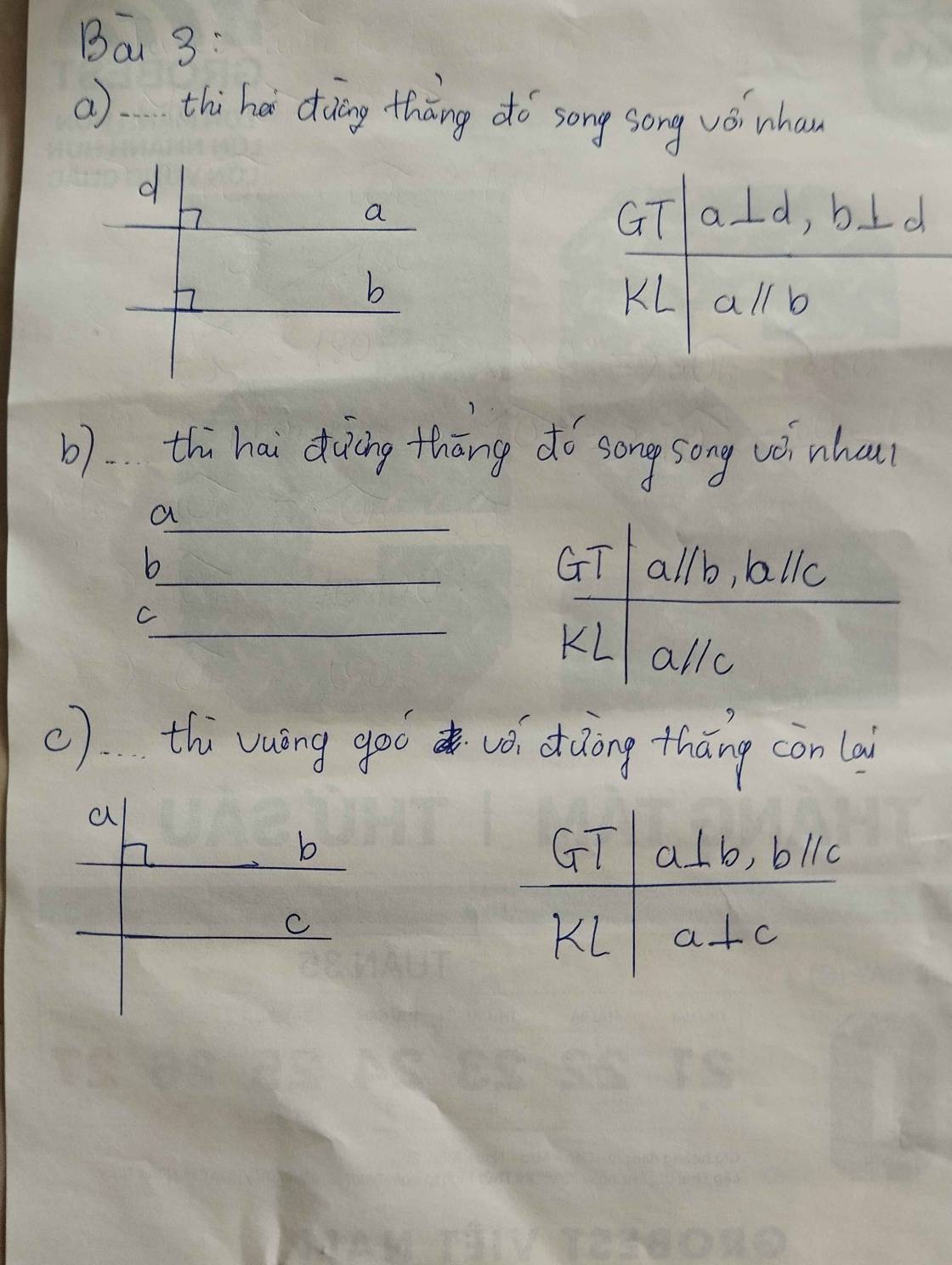







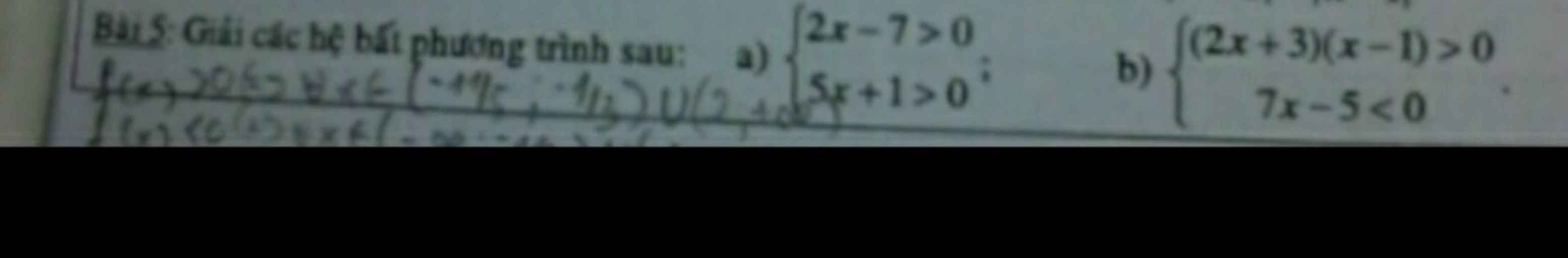
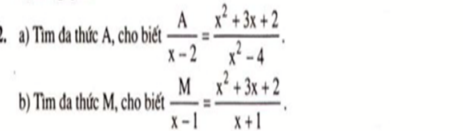 mọi người giải giúp em bài này với ạ em đang cần gấp ạ
mọi người giải giúp em bài này với ạ em đang cần gấp ạ
