Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(y'=3mx^2-4mx-\left(m+1\right)\)
- Với \(m=0\Rightarrow y'=-1< 0\) hàm nghịch biến trên R (thỏa)
- Với \(m\ne0\) hàm nghịch biến trên R khi:
\(\left\{{}\begin{matrix}3m< 0\\\Delta'=4m^2+3m\left(m+1\right)\le0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m< 0\\7m^2+3m\le0\\\end{matrix}\right.\)
\(\Leftrightarrow-\dfrac{3}{7}\le m< 0\)
Vậy \(-\dfrac{3}{7}\le m\le0\Rightarrow m=0\)
S có 1 phần tử


Vì \(f\left(b\right)\) đồng biến nên nếu \(f\left(-8\right)>0\Rightarrow f\left(b\right)>0;\forall b>-8\)
\(\Rightarrow f\left(b\right)\le0\) có nhiều nhất 3 nghiệm nguyên thuộc (-12;12) là -11;-10;-9 (ktm yêu cầu đề bài)
Do đó \(f\left(-8\right)\le0\)
Hiểu đơn giản thì đếm từ -11 trở đi thêm 4 số nguyên ta sẽ chạm tới mốc -8


Gọi \(M\left(x;y\right)\) là điểm biểu diễn z trên mặt phẳng tọa độ
Gọi \(A\left(-2;1\right)\) ; \(B\left(2;3\right)\) ; \(C\left(-1;2\right)\)
\(\Rightarrow\overrightarrow{AB}=\left(4;2\right)\Rightarrow AB=2\sqrt{5}\)
Từ \(\left|z+2-i\right|+\left|z-2-3i\right|=2\sqrt{5}\Leftrightarrow MA+MB=2\sqrt{5}\)
\(\Leftrightarrow MA+MB=AB\Leftrightarrow\) M nằm trên đoạn thẳng AB
\(\left|z+i-2i\right|=MC\) đạt GTNN khi và chỉ khi M là hình chiếu vuông góc của C lên AB
Phương trình đường thẳng AB:
\(1\left(x+2\right)-2\left(y-1\right)=0\Leftrightarrow x-2y+4=0\)
Phương trình đường thẳng d qua C và vuông góc AB:
\(2\left(x+1\right)+1\left(y-2\right)=0\Leftrightarrow2x+y=0\)
Tọa độ M là nghiệm: \(\left\{{}\begin{matrix}x-2y+4=0\\2x+y=0\end{matrix}\right.\) \(\Rightarrow M\left(-\dfrac{4}{5};\dfrac{8}{5}\right)\)
\(\Rightarrow\overrightarrow{MC}=\left(-\dfrac{1}{5};-\dfrac{2}{5}\right)\Rightarrow MC=\dfrac{\sqrt{5}}{5}\)
Đáp án B

\(y'=-3mx^2+2x-3\)
Hàm nghịch biến trên khoảng đã cho khi với mọi \(x\in\left(-3;0\right)\) ta có:
\(-3mx^2+2x-3\le0\)
\(\Leftrightarrow2x-3\le3mx^2\)
\(\Leftrightarrow\dfrac{2x-3}{3x^2}\le m\)
\(\Rightarrow m\ge\max\limits_{\left(-3;0\right)}\left(\dfrac{2x-3}{3x^2}\right)\)
Xét hàm \(f\left(x\right)=\dfrac{2x-3}{3x^2}\Rightarrow f'\left(x\right)=\dfrac{2\left(3-x\right)}{3x^3}< 0;\forall x\in\left(-3;0\right)\)
\(\Rightarrow f\left(x\right)>f\left(-3\right)=-\dfrac{1}{3}\)
\(\Rightarrow m\ge-\dfrac{1}{3}\)

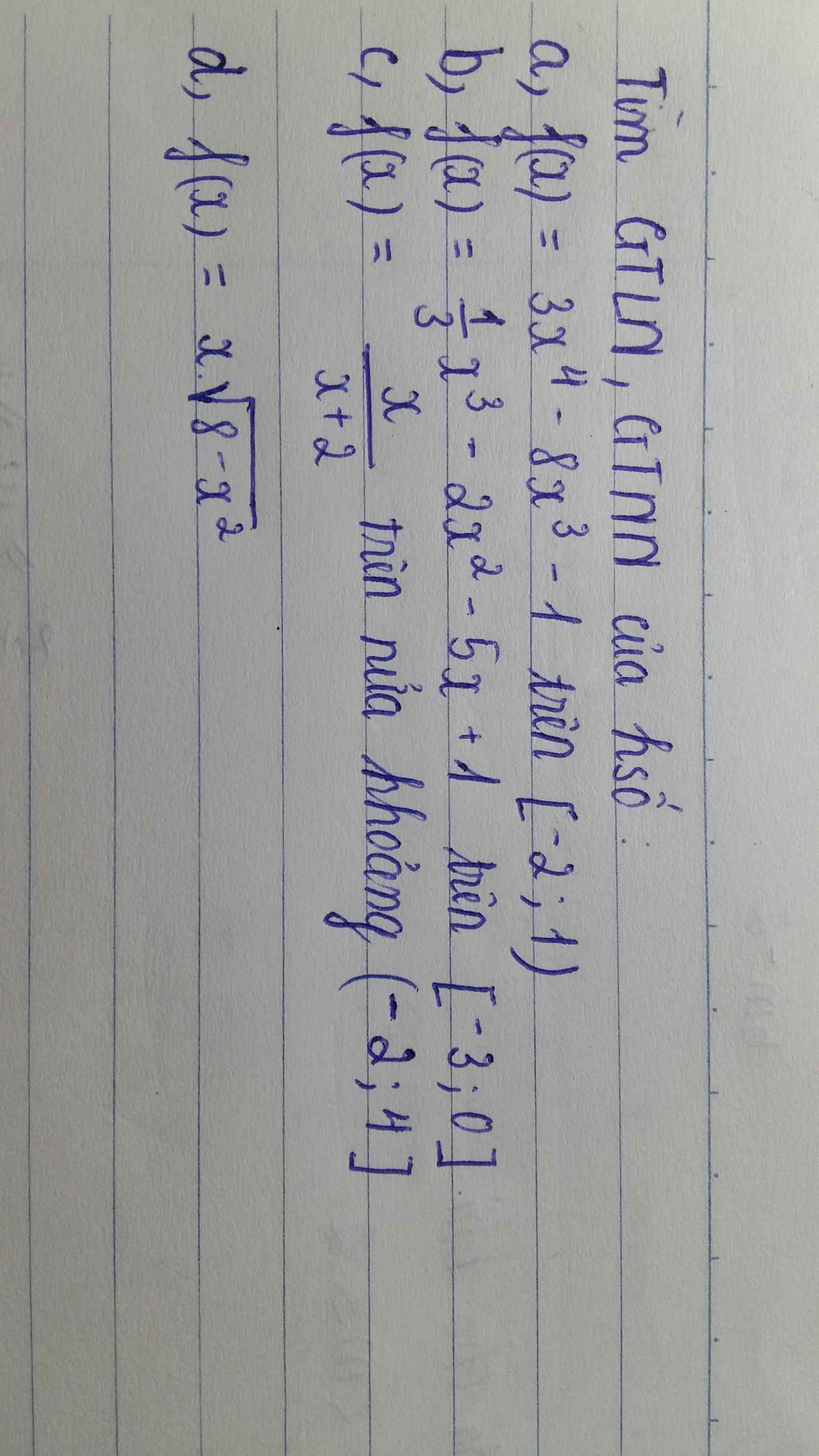






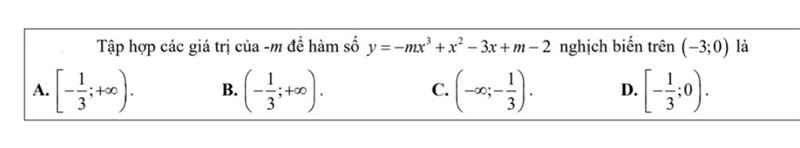
a. \(f\left(x\right)_{max}=f\left(-2\right)=111\) ; \(f\left(x\right)_{min}=f\left(1\right)=-6\)
b. \(f\left(x\right)_{max}=f\left(-3\right)=7\) ; \(f\left(x\right)_{min}=f\left(0\right)=1\)
c. \(f\left(x\right)_{max}=f\left(4\right)=\dfrac{2}{3}\) ; \(f\left(x\right)_{min}\) ko tồn tại
d.
Miền xác định: \(D=\left[-2\sqrt{2};2\sqrt{2}\right]\)
\(y'=\dfrac{2\left(4-x^2\right)}{\sqrt{8-x^2}}=0\Rightarrow\left[{}\begin{matrix}x=-2\\x=2\end{matrix}\right.\)
\(f\left(-2\sqrt{2}\right)=f\left(2\sqrt{2}\right)=0\)
\(f\left(-2\right)=-4\) ; \(f\left(2\right)=4\)
\(f\left(x\right)_{max}=f\left(2\right)=4\) ; \(f\left(x\right)_{min}=f\left(-2\right)=-4\)