Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(\lim\limits_{x\rightarrow1}\dfrac{x^3-3x+2}{x^4-4x+3}=\lim\limits_{x\rightarrow1}\dfrac{\left(x+2\right)\left(x-1\right)^2}{\left(x^2+2x+3\right)\left(x-1\right)^2}=\lim\limits_{x\rightarrow1}\dfrac{x+2}{x^2+2x+3}=\dfrac{1}{2}\)
\(\lim\limits_{x\rightarrow2^-}\dfrac{x^3+x^2-4x-4}{x^2-4x+4}=\lim\limits_{x\rightarrow2^-}\dfrac{\left(x-2\right)\left(x^2+3x+2\right)}{\left(x-2\right)^2}=\lim\limits_{x\rightarrow2^-}\dfrac{x^2+3x+2}{x-2}=-\infty\)
\(\lim\limits_{x\rightarrow2}\dfrac{\left(x^2-x-2\right)^{20}}{\left(x^3-12x+16\right)^{10}}=\lim\limits_{x\rightarrow2}\dfrac{\left(x+1\right)^{20}\left(x-2\right)^{20}}{\left(x+4\right)^{10}\left(x-2\right)^{20}}=\lim\limits_{x\rightarrow2}\dfrac{\left(x+1\right)^{20}}{\left(x+4\right)^{10}}=\dfrac{3^{10}}{2^{10}}\)
\(\lim\limits_{x\rightarrow0^-}\dfrac{4x^2+5x}{x^2}=\lim\limits_{x\rightarrow0^-}\dfrac{4x+5}{x}=-\infty\)
\(\lim\limits_{x\rightarrow-1}\dfrac{\sqrt{x+2}-1}{\sqrt{x+5}-2}=\lim\limits_{x\rightarrow-1}\dfrac{\left(x+1\right)\left(\sqrt{x+5}+2\right)}{\left(x+1\right)\left(\sqrt{x+2}+1\right)}=\lim\limits_{x\rightarrow-1}\dfrac{\sqrt{x+5}+2}{\sqrt{x+2}+1}=2\)

a) Cách 1: y' = (9 -2x)'(2x3- 9x2 +1) +(9 -2x)(2x3- 9x2 +1)' = -2(2x3- 9x2 +1) +(9 -2x)(6x2 -18x) = -16x3 +108x2 -162x -2.
Cách 2: y = -4x4 +36x3 -81x2 -2x +9, do đó
y' = -16x3 +108x2 -162x -2.
b) y' = .(7x -3) +
(7x -3)'=
(7x -3) +7
.
c) y' = (x -2)'√(x2 +1) + (x -2)(√x2 +1)' = √(x2 +1) + (x -2) = √(x2 +1) + (x -2)
= √(x2 +1) +
=
.
d) y' = 2tanx.(tanx)' - (x2)' =
.
e) y' = sin
=
sin
.
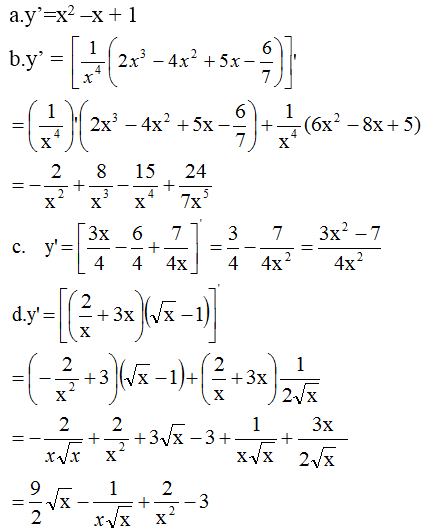
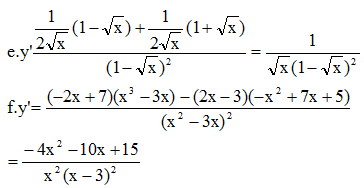
a: \(\left(\sqrt{3}\right)^x=243\)
=>\(3^{\dfrac{1}{2}\cdot x}=3^5\)
=>\(\dfrac{1}{2}\cdot x=5\)
=>x=10
b: \(0,1^x=1000\)
=>\(\left(\dfrac{1}{10}\right)^x=1000\)
=>\(10^{-x}=10^3\)
=>-x=3
=>x=-3
c: \(\left(0,2\right)^{x+3}< \dfrac{1}{5}\)
=>\(\left(0,2\right)^{x+3}< 0,2\)
=>x+3>1
=>x>-2
d: \(\left(\dfrac{3}{5}\right)^{2x+1}>\left(\dfrac{5}{3}\right)^2\)
=>\(\left(\dfrac{3}{5}\right)^{2x+1}>\left(\dfrac{3}{5}\right)^{-2}\)
=>2x+1<-2
=>2x<-3
=>\(x< -\dfrac{3}{2}\)
e: \(5^{x-1}+5^{x+2}=3\)
=>\(5^x\cdot\dfrac{1}{5}+5^x\cdot25=3\)
=>\(5^x=\dfrac{3}{25,2}=\dfrac{1}{8,4}=\dfrac{10}{84}=\dfrac{5}{42}\)
=>\(x=log_5\left(\dfrac{5}{42}\right)=1-log_542\)