Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

x^4 -x ^3 + 6x^2 - x + n x^2-x+5 x^2+1 - x^4-x^3+5x^2 x^2-x+n - x^2-x+n 0
ĐỂ x4 - x3 + 6x2 -x \(⋮x^2-x+5\)
\(\Rightarrow x-5=0\Rightarrow x=5\)
b , ta có : \(3x^3+10x^2-5⋮3x+1\)
\(\Rightarrow3x^3+x^2+9x^2+3x-3x-1-4⋮3x+1\)
\(\Rightarrow x\left(3x+1\right)+3x\left(3x+1\right)-\left(3x+1\right)-4⋮3x+1\)
mà : \(\left(3x+1\right)\left(4x-1\right)⋮3x+1\)
\(\Rightarrow4⋮3x+1\Rightarrow3x+1\inƯ\left(4\right)=\left\{\pm1;\pm2;\pm4\right\}\)
Nếu : 3x + 1 = 1 => x = 0 ( TM )
3x + 1 = -1 => x = -2/3 ( loại )
3x + 1 = 2 => x = 1/3 ( loại )
3x + 1 = -2 => x = -1 ( TM )
3x + 1 = 4 => x = 1 ( TM )
3x + 1 = -1 => x = -5/3 ( loại )
\(\Rightarrow x\in\left\{0;\pm1\right\}\)

a) Áp dụng định lý Bézout ( Bê-du ) , dư của \(f\left(x\right)=x^3+x^2-x+a\)cho x + 2 = x - (-2) là \(f\left(-2\right)\)
Để f(x) chia hết cho x + 2 thì f(-2)=0
\(\Rightarrow\left(-2\right)^3+\left(-2\right)^2-\left(-2\right)+a=0\)
\(-8+4+2+a=0\)
\(a-2=0\)
\(a=2\)
Vậy ...
c) \(\frac{n^3+n^2-n+5}{n+2}=\frac{n^3+2n^2-n^2-2n+n+2+3}{n+2}\)nguyên để \(n^3+n^2-n+5⋮n+2\)
\(\Rightarrow\frac{n^2\left(n+2\right)-n\left(n+2\right)+\left(n+2\right)+3}{n+2}\in Z\)
\(\Rightarrow n^2-n+1+\frac{3}{n+2}\in Z\)
\(n^2,n,1\in Z\Rightarrow\frac{3}{n+2}\in Z\)
\(\Rightarrow n+2\inƯ\left(3\right)=\left\{-3;-1;1;3\right\}\)
\(\Rightarrow n\in\left\{-5;-3;-1;1\right\}\)
Vậy ...

b: \(\Leftrightarrow2n^2+n-2n-1+3⋮2n+1\)
\(\Leftrightarrow2n+1\in\left\{1;-1;3;-3\right\}\)
hay \(n\in\left\{0;-1;1;-2\right\}\)

mk gửi cho link:
https://lazi.vn/edu/exercise/tim-n-de-da-thuc-x4-x3-6x2-x-n-chia-het-cho-da-thuc-x2-x-5
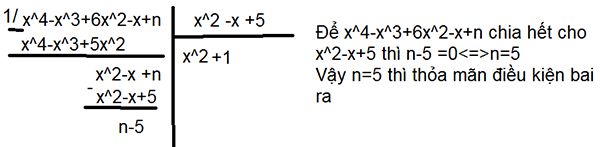
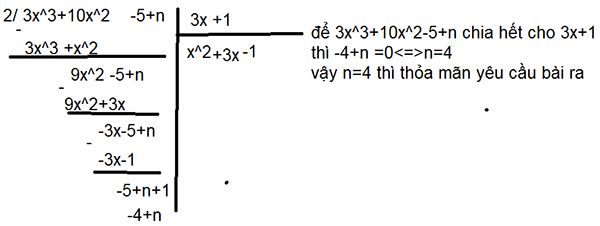
a) đề x3+x2-x +a chia hét cho (x-1)2 ?
x3+x2-x +a=x(x2-2x+1)+3(x2-2x+1)+4x-3+a đề sai nhé
b)A(2)=0=> 8-12+10+m=0 => m=6
c)2n2-n+2=2n(n+1)-3(n+1) +5 chia het cho n+1 khi n+1 là ước của 5
n+1=-1;1;-5;5
n=-2;0;-6;4