NM
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
26 tháng 10 2021
1. Ta có: hằng đẳng thức: \(x^3+y^3+z^3=3xyz\) nếu x+y+z=0
đặt b-c=x, c-a=y, a-b=z⇒x+y+z=0
\(\Rightarrow\left(b-c\right)^3+\left(c-a\right)^3+\left(a-b\right)^3=3\left(a-b\right)\left(c-a\right)\left(b-c\right)\)
2. \(x^3+y^3+z^3-3xyz=\left(x+y+z\right)\left(x^2+y^2+z^2-xy-yz-zx\right)+3xyz-3xyz=\left(x+y+z\right)\left(x^2+y^2+z^2-xy-yz-zx\right)\)
3. Tham khảo: https://hoc247.net/hoi-dap/toan-8/phan-tich-da-thuc-x-y-5-x-5-y-5-thanh-nhan-tu-faq447273.html
26 tháng 10 2021
\(5,=x^3+2x^2y-7x^2y-14xy^2\\ =x^2\left(x+2y\right)-7xy\left(x+2y\right)\\ =x\left(x-7y\right)\left(x+2y\right)\)
NT
0
TL
1
CM
13 tháng 10 2017
x 2 y + x y 2 + x 2 z + x z 2 + y 2 z + y z 2 + 3xyz.
= ( x 2 y + x 2 z + xyz) + (x y 2 + y 2 z + xyz) + (x z 2 + y z 2 + xyz)
= x(xy + xz + yz) + y(xy + yz + xz) + z(xz + yz + xy)
= (x + y + z)(xy + xz + yz).
NC
14 tháng 12 2020
\(x^2y+xy^2+x^2z+xz^2+y^2z+yz^2+3xyz\)
\(=\left(x^2y+x^2z+xyz\right)+\left(xz^2+yz^2+xyz\right)+\left(xy^2+y^2z+xyz\right)\)
\(=x\left(xy+xz+yz\right)+z\left(xz+yz+xy\right)+y\left(xy+yz+xz\right)\)
\(=\left(x+y+z\right)\left(xy+yz+xz\right)\)
20 tháng 10 2021
b: \(x^2-2xy+y^2-z^2\)
\(=\left(x-y\right)^2-z^2\)
\(=\left(x-y-z\right)\left(x-y+z\right)\)
d: \(x^2+4x+3=\left(x+3\right)\left(x+1\right)\)
NV
Nguyễn Việt Lâm
Giáo viên
3 tháng 8 2021
\(=\left(x^2-2x+1\right)-\left(y^2-2yz+z^2\right)\)
\(=\left(x-1\right)^2-\left(y-z\right)^2\)
\(=\left(x-1-y+z\right)\left(x-1+y-z\right)\)
3 tháng 8 2021
\(x^2-2x+1-y^2+2yz-z^2\)
\(=\left(x-1\right)^2-\left(y-z\right)^2\)
\(=\left(x-1-y+z\right)\left(x-1+y-z\right)\)
PT
1
CM
15 tháng 4 2017
x2 – 2xy + y2 – z2 + 2zt – t2
(Nhận thấy x2 – 2xy + y2 và z2 – 2zt + t2 là các hằng đẳng thức)
= (x2 – 2xy + y2) – (z2 – 2zt + t2)
= (x – y)2 – (z – t)2 (xuất hiện hằng đẳng thức (3))
= [(x – y) – (z – t)][(x – y) + (z – t)]
= (x – y – z + t)(x – y + z –t)
HT
2
25 tháng 8 2021
a: Ta có: \(x^2-xy-3x+3y\)
\(=x\left(x-y\right)-3\left(x-y\right)\)
\(=\left(x-y\right)\left(x-3\right)\)
b: Ta có: \(5x^2+5xy-x-y\)
\(=5x\left(x+y\right)-\left(x+y\right)\)
\(=\left(x+y\right)\left(5x-1\right)\)
c: Ta có: \(x^2-2xy+y^2-z^2\)
\(=\left(x-y\right)^2-z^2\)
\(=\left(x-y-z\right)\left(x-y+z\right)\)
1 tháng 11 2021
\(1,\\ a,=\left(x-y\right)^2-z^2=\left(x-y-z\right)\left(x-y+z\right)\\ b,=a^2\left(a-x\right)-y\left(a-x\right)=\left(a^2-y\right)\left(a-x\right)\\ c,=\left(x-y\right)^2-z\left(x-y\right)=\left(x-y\right)\left(x-y-z\right)\\ d,=x\left(x-2y\right)+t\left(x-2y\right)=\left(x+t\right)\left(x-2y\right)\\ 2,\\ \Rightarrow x^2-4x+4-x^2+9=6\\ \Rightarrow-4x=-7\Rightarrow x=\dfrac{7}{4}\\ 3,\\ a,x^2+2x+2=\left(x+1\right)^2+1\ge1>0\\ b,-x^2+4x-5=-\left(x-2\right)^2-1\le-1< 0\)
PT
1
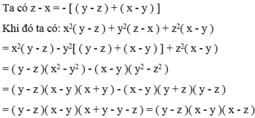
thanks nhìu