Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Chọn D.
x 4 + 8x = x( x 3 +8)= x( x 3 + 2 3 ) = x(x + 2)( x 2 − 2x + 4)

a/ \(\left(x+y\right)^2-8\left(x+y\right)+12\)
\(=\left(x+y\right)\left(x+y-8+12\right)\)
\(=\left(x+y\right)\left(x+y+4\right)\)
==========
b/\(\left(x^2+2x\right)^2-2x^2-4x-3\)
\(=\left(x^2+2x\right)^2-\left(2x^2+4x\right)-3\)
\(=\left(x^2+2x\right)^2-2\left(x^2+2x\right)-3\)
\(=\left(x^2+2x\right)\left(x^2+2x-5\right)\)
===========
c/ \(\left(x^2+x\right)^2-2\left(x^2+x\right)-15\)
\(=\left(x^2+x\right)\left(x^2+x-2-15\right)\)
\(=\left(x^2+x\right)\left(x^2+x-17\right)\)
[---]

Bài 3
a) x² + 10x + 25
= x² + 2.x.5 + 5²
= (x + 5)²
b) 8x - 16 - x²
= -(x² - 8x + 16)
= -(x² - 2.x.4 + 4²)
= -(x - 4)²
c) x³ + 3x² + 3x + 1
= x³ + 3.x².1 + 3.x.1² + 1³
= (x + 1)³
d) (x + y)² - 9x²
= (x + y)² - (3x)²
= (x + y - 3x)(x + y + 3x)
= (y - 2x)(4x + y)
e) (x + 5)² - (2x - 1)²
= (x + 5 - 2x + 1)(x + 5 + 2x - 1)
= (6 - x)(3x + 4)
Bài 4
a) x² - 9 = 0
x² = 9
x = 3 hoặc x = -3
b) (x - 4)² - 36 = 0
(x - 4 - 6)(x - 4 + 6) = 0
(x - 10)(x + 2) = 0
x - 10 = 0 hoặc x + 2 = 0
*) x - 10 = 0
x = 10
*) x + 2 = 0
x = -2
Vậy x = -2; x = 10
c) x² - 10x = -25
x² - 10x + 25 = 0
(x - 5)² = 0
x - 5 = 0
x = 5
d) x² + 5x + 6 = 0
x² + 2x + 3x + 6 = 0
(x² + 2x) + (3x + 6) = 0
x(x + 2) + 3(x + 2) = 0
(x + 2)(x + 3) = 0
x + 2 = 0 hoặc x + 3 = 0
*) x + 2 = 0
x = -2
*) x + 3 = 0
x = -3
Vậy x = -3; x = -2

a) (x - 1)(x + l)(x - 2)(x - 4). b) (x - 2)( x 2 + 4).
c) 2y(3 x 2 + y 2 ). d) 2(x + y + z) ( a - b ) 2 .
a. \(x^2\left(x-3\right)^2-\left(x-3\right)^2-x^2+1\)
\(=\left(x-3\right)^2\left(x^2-1\right)-\left(x^2-1\right)\)
\(=\left[\left(x-3\right)^2-1\right]\left(x^2-1\right)\)
\(=\left(x-3+1\right)\left(x-3-1\right)\left(x+1\right)\left(x-1\right)\)
\(=\left(x-2\right)\left(x-4\right)\left(x+1\right)\left(x-1\right)\)
b. \(x^3-2x^2+4x-8\)
\(=\left(x^3+4x\right)-\left(2x^2+8\right)\)
\(=x\left(x^2+4\right)-2\left(x^2+4\right)\)
\(=\left(x-2\right)\left(x^2+4\right)\)
c. \(\left(x+y\right)^3-\left(x-y\right)^3\)
\(=\left(x^3+3x^2y+3xy^2+y^3\right)-\left(x^3-3x^2y+3xy^2-y^3\right)\)
\(=x^3+3x^2y+3xy^2+y^3-x^3+3x^2y-3xy^2+y^3\)
\(=6x^2y+2y^3\)
\(=2y\left(3x^2+y^2\right)\)
d. \(2a^2\left(x+y+z\right)-4ab\left(x+y+z\right)+2b^2\left(x+y+z\right)\)
\(=\left(2a^2-4ab+2b^2\right)\left(x+y+z\right)\)
\(=2\left(a^2-2ab+b^2\right)\left(x+y+z\right)\)
\(=2\left(a-b\right)^2\left(x+y+z\right)\)
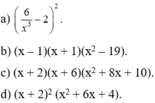


a, Đặt \(x^2+4x+8=a,x=b\)
\(\left(a\right)\)\(\Leftrightarrow a^2+3ab+2b^2\)\(=\)\(\left(a+b\right)\left(a+2b\right)\)\(=\left(x^2+5x+8\right)\left(x^2+6x+8\right)\)
b, Đặt \(x^2+x+1=t\)
\(\left(b\right)=t.\left(t+1\right)-12=t^2+t-12\)\(=\left(t-3\right)\left(t+4\right)\)
\(=\left(x^2+x-2\right)\left(x^2+x+5\right)\)
c, Tương tự câu b
d,
\(\left(d\right)=\left(x^2+7x+10\right)\left(x^2+7x+12\right)-24\)
Đặt \(x^2+7x+10=t\)
\(\left(d\right)=t\left(t+2\right)-24=t^2+2t-24=\left(t-4\right)\left(t+6\right)\)
\(=\left(x^2+7x+6\right)\left(x^2+7x+16\right)\)