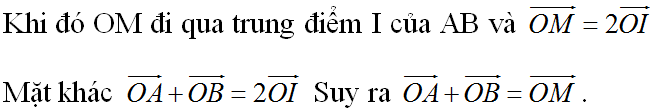Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: \(\overrightarrow{AM}+\overrightarrow{BN}=\dfrac{1}{2}\overrightarrow{AB}+\dfrac{1}{2}\overrightarrow{BC}=\dfrac{1}{2}\overrightarrow{AC}\)
b: \(=\dfrac{1}{2}\overrightarrow{AC}+\dfrac{1}{2}\overrightarrow{AC}+\dfrac{1}{2}\overrightarrow{BA}\)
\(=\overrightarrow{AC}+\dfrac{1}{2}\overrightarrow{BA}\)
c: \(\overrightarrow{AM}+\overrightarrow{BN}+\overrightarrow{CP}\)
\(=\dfrac{1}{2}\overrightarrow{AB}+\dfrac{1}{2}\overrightarrow{BC}+\dfrac{1}{2}\overrightarrow{CA}\)
\(=\dfrac{1}{2}\left(\overrightarrow{AC}+\overrightarrow{CA}\right)=\overrightarrow{0}\)

Ta có:
\(\overrightarrow{AM}=\dfrac{1}{2}\left(\overrightarrow{AO}+\overrightarrow{AB}\right)\)
\(\Leftrightarrow\overrightarrow{AM}=\dfrac{1}{2}\left(\overrightarrow{AO}+\overrightarrow{AO}+\overrightarrow{OB}\right)\)
\(\Leftrightarrow\overrightarrow{AM}=\dfrac{1}{2}\left(2\overrightarrow{AO}+\overrightarrow{OB}\right)\)
\(\Leftrightarrow\overrightarrow{AM}=\overrightarrow{AO}+\dfrac{1}{2}\overrightarrow{OB}\)
\(\Leftrightarrow\overrightarrow{AM}=\dfrac{1}{2}\overrightarrow{OB}-\overrightarrow{OA}\)
\(\RightarrowĐPCM\)
Câu b ) Bạn làm tương tự câu a , ta có vecto BN = 1/2 (BO +BC ) , rồi là như câu a
chúc bạn hok tốt

câu 2 ( các kí hiệu vecto khi lm bài thỳ b tự viết nhé mk k viết kí hiệu để trả lời cho nhanh hỳ hỳ )
OA+ OB + OC = OA'+ OB' + OC'
<=> OA - OA' + OB - OB' + OC - OC' = 0
<=> A'A + B'B + C'C = 0
<=> 2 ( BA + CB + AC ) = 0
<=> 2 ( CB + BA + AC ) = 0
<=> 2 ( CA + AC ) = 0
<=> 0 = 0 ( luôn đúng )
câu 1 ( các kí hiệu vecto b cx tự viết nhá )
VT = OD + OC = OA + AD + OB + BC = OA + OB + AD + BC = BO + OB + AD + BC = 0 + AD + BC = AD + BC = VP ( đpcm)

b)
O B A M N
\(\overrightarrow{AN}=\dfrac{1}{2}\overrightarrow{AO}=-\dfrac{1}{2}\overrightarrow{OA}\)
Vậy \(m=-\dfrac{1}{2};n=0\).
c)
\(\overrightarrow{MN}=\dfrac{1}{2}\overrightarrow{AB}=\dfrac{1}{2}\left(\overrightarrow{AO}+\overrightarrow{OB}\right)=-\dfrac{1}{2}\overrightarrow{OA}+\dfrac{1}{2}\overrightarrow{OB}\).
Vậy \(m=-\dfrac{1}{2};n=\dfrac{1}{2}\).
d)
\(\overrightarrow{MB}=\dfrac{1}{2}\overrightarrow{OB}\)
Vậy \(m=0;n=\dfrac{1}{2}\).

A B C P N M
a)
Có: \(3\overrightarrow{OC}-\overrightarrow{OB}=3\left(\overrightarrow{OM}+\overrightarrow{MC}\right)-\left(\overrightarrow{OM}+\overrightarrow{MB}\right)\)
\(=2\overrightarrow{OM}+3\overrightarrow{MC}-\overrightarrow{MB}\)\(=2\overrightarrow{OM}+\overrightarrow{MB}-\overrightarrow{MB}=2\overrightarrow{OM}\). (Đpcm).
b)
Gọi G là trọng tâm tam giác ABC, ta chứng minh G cũng là trọng tâm tam giác MNP.
Ta có: \(\overrightarrow{GA}+\overrightarrow{GB}+\overrightarrow{GC}=\overrightarrow{0}\).
Ta cần chứng minh: \(\overrightarrow{GN}+\overrightarrow{GM}+\overrightarrow{GP}=\overrightarrow{0}\).
Thật vậy \(\overrightarrow{GN}+\overrightarrow{GM}+\overrightarrow{GP}=\overrightarrow{GC}+\overrightarrow{CN}+\overrightarrow{GB}+\overrightarrow{BM}+\overrightarrow{GA}+\overrightarrow{AP}\)
\(=\left(\overrightarrow{GA}+\overrightarrow{GB}+\overrightarrow{GC}\right)+\overrightarrow{CN}+\overrightarrow{BM}+\overrightarrow{AP}\)
\(=\overrightarrow{0}+\overrightarrow{CN}+\overrightarrow{BM}+\overrightarrow{AP}\)
\(=\dfrac{3}{4}\overrightarrow{CA}+\dfrac{3}{4}\overrightarrow{BC}+\dfrac{3}{4}\overrightarrow{AB}\)
\(=\dfrac{3}{4}\left(\overrightarrow{CA}+\overrightarrow{AB}\right)+\dfrac{3}{4}\overrightarrow{BC}\)
\(=\dfrac{3}{4}\overrightarrow{CB}+\dfrac{3}{4}\overrightarrow{BC}=\overrightarrow{0}\).
Vậy G cũng là trọng tâm tam giác MNP. (Đpcm).