Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

2x3 + 3x2 + 6x + 5 = 02
<=> 2x3 + x2 + 5x + 2x2 + x + 5 = 0
<=> x(2x2 + x + 5) + (2x2 + x + 5) = 0
<=> (2x2 + x + 5)(x + 1) = 0
<=> x + 1 = 0 (vì 2x2 + x + 5 \(\ge\) 4,875 > 0 \(\forall\) x)
<=> x = - 1
Vậy tập nghiệm của pt là \(S=\left\{-1\right\}\)
b) 4x4 + 12x3 + 5x2 - 6x - 15 = 0
<=> 4x4 + 10x3 + 2x3 + 5x2 - 6x - 15 = 0
<=> 2x3(2x + 5) + x2(2x + 5) - 3(2x + 5) = 0
<=> (2x + 5)(2x3 + x2 - 3) = 0
<=> (2x + 5)(2x3 - 2x2 + 3x2 - 3) = 0
<=> (2x + 5)(x - 1)(2x2 + 3x + 3) = 0
<=> (2x + 5)(x - 1)[x2 + (x + 3/2)2 + 3/4]= 0
Mà x2 + (x + 3/2)2 + 3/4 > 0\(\forall x\)
\(\Rightarrow\left[\begin{matrix}2x+5=0\\x-1=0\end{matrix}\right.\)\(\Leftrightarrow\left[\begin{matrix}x=-\frac{5}{2}\\x=1\end{matrix}\right.\)
Vậy ...

\(DKXD:x\ne\pm2;x\ne3;x\ne\frac{3}{2};x\ne0\)
\(A=\left(\frac{2+x}{2-x}+\frac{4x^2}{x^2-4}-\frac{2-x}{2+x}\right):\left(\frac{x^2-3x}{2x^2-3x}\right)\)
\(=\frac{\left(2+x\right)^2-4x^2-\left(2-x\right)^2}{\left(2-x\right)\left(2+x\right)}\cdot\frac{2x^2-3x}{x^2-3x}\)
\(=\frac{4+4x+x^2-4x^2-4+4x-x^2}{\left(2-x\right)\left(2+x\right)}\cdot\frac{x\left(2x-3\right)}{x\left(x-3\right)}\)
\(=\frac{8x-4x^2}{\left(2-x\right)\left(2+x\right)}\cdot\frac{2x-3}{x-3}\)
\(=\frac{4x\left(2x-3\right)}{\left(2+x\right)\left(x-3\right)}\)
b
Xét hơi bị nhiều TH nhá:(
Để \(A>0\) thì \(\frac{4x\left(2x-3\right)}{\left(2+x\right)\left(x-3\right)}>0\)
TH1:\(4x\left(2x-3\right)>0;\left(2+x\right)\left(x-3\right)>0\)
\(TH2:4x\left(2x-3\right)< 0;\left(2+x\right)\left(x-3\right)< 0\)
Bạn tự xét nốt nhá!
c
\(\left|x-7\right|=4\Rightarrow x-7=4;x-7=-4\)
\(\Rightarrow x=11;x=3\)
Thay vào .....

\(a,\left(2x^2+1\right)+4x>2x\left(x-2\right)\)
\(\Leftrightarrow2x^2+1+4x>2x^2-4x\)
\(\Leftrightarrow4x+4x>-1\)
\(\Leftrightarrow8x>-1\)
\(\Leftrightarrow x>-\frac{1}{8}\)
\(b,\left(4x+3\right)\left(x-1\right)< 6x^2-x+1\)
\(\Leftrightarrow4x^2-4x+3x-3< 6x^2-x+1\)
\(\Leftrightarrow4x^2-x-3< 6x^2-x+1\)
\(\Leftrightarrow4x^2-6x^2< 1+3\)
\(\Leftrightarrow-2x^2< 4\)
\(\Leftrightarrow x^2>2\)
\(\Leftrightarrow x>\pm\sqrt{2}\)

a)\(4x^4+y^4=\left(4x^4+y^4+4x^2y^2\right)-4x^2y^2\)
\(=\left(2x^2+y^2\right)^2-\left(2xy\right)^2\)
\(=\left(2x^2+y^2-2xy\right)\left(2x^2+y^2+2xy\right)\)
b)\(\left(x^2-3x-1\right)^2-12\left(x^2-3x-1\right)+27\)
Đặt x^2 - 3x - 1 = A
\(\Rightarrow A^2-12A+27=\left(A^2-12A+36\right)-9\)
\(=\left(A-6\right)^2-9=\left(A-6-3\right)\left(A-6+3\right)\)
\(=\left(A-9\right)\left(A-3\right)\)
Hay \(=\left(x^2-3x-1-9\right)\left(x^2-3x-1-3\right)\)
\(=\left(x^2-3x-10\right)\left(x^2-3x-4\right)\)
\(=\left(x-5\right)\left(x+2\right)\left(x-4\right)\left(x+1\right)\)
c)\(x^3-x^2-5x+125\)
\(=\left(x^3+5^3\right)-\left(x^2+5x\right)\)
\(=\left(x+5\right)\left(x^2-5x+25\right)-x\left(x+5\right)\)
\(=\left(x+5\right)\left(x^2-5x+25-x\right)\)
\(=\left(x+5\right)\left(x^2-6x+25\right)\)
d)\(xy\left(x+y\right)+yz\left(y+z\right)+zx\left(z+x\right)+2xyz\)
\(=\left(x+y\right)\left(y+z\right)\left(x+z\right)\)
Mình có việc bận nên chỉ đưa được kết quả ý d) thật lòng mong các bạn tự tham khảo và giải

a) Đk: x > 0 và x khác +-1
Ta có: A = \(\left(\frac{x+1}{x}-\frac{1}{1-x}-\frac{x^2-2}{x^2-x}\right):\frac{x^2+x}{x^2-2x+1}\)
A = \(\left[\frac{\left(x-1\right)\left(x+1\right)+x-x^2+2}{x\left(x-1\right)}\right]:\frac{x\left(x+1\right)}{\left(x-1\right)^2}\)
A = \(\frac{x^2-1+x-x^2+2}{x\left(x-1\right)}\cdot\frac{\left(x-1\right)^2}{x\left(x+1\right)}\)
A = \(\frac{x+1}{x}\cdot\frac{x-1}{x\left(x+1\right)}=\frac{x-1}{x^2}\)
b) Ta có: A = \(\frac{x-1}{x^2}=\frac{1}{x}-\frac{1}{x^2}=-\left(\frac{1}{x^2}-\frac{1}{x}+\frac{1}{4}\right)+\frac{1}{4}=-\left(\frac{1}{x}-\frac{1}{2}\right)^2+\frac{1}{4}\le\frac{1}{4}\forall x\)
Dấu "=" xảy ra <=> 1/x - 1/2 = 0 <=> x = 2 (tm)
Vậy MaxA = 1/4 <=> x = 2

a: \(A=2x^2-2xy-y^2+2xy=2x^2-y^2\)
\(=2\cdot\dfrac{4}{9}-\dfrac{1}{9}=\dfrac{7}{9}\)
b: \(B=5x^2-20xy-4y^2+20xy=5x^2-4y^2\)
\(=5\cdot\dfrac{1}{25}-4\cdot\dfrac{1}{4}\)
=1/5-1=-4/5
c \(C=x^3+6x^2+12x+8=\left(x+2\right)^3=\left(-9\right)^3=-729\)
d: \(D=20x^3-10x^2+5x-20x^2+10x+4\)
\(=20x^3-30x^2+15x+4\)
\(=20\cdot5^3-30\cdot5^2+15\cdot2+4=1784\)
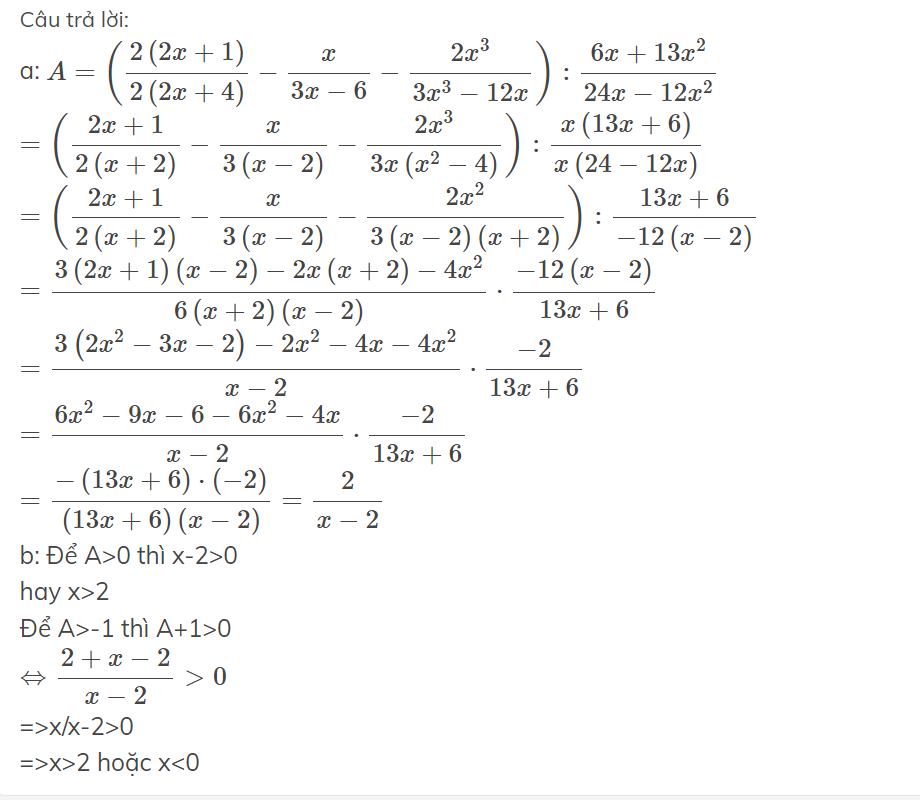
(Bài dưới được trình bày dựa theo cách trình bày ở Ví dụ 1 trang 50 sgk Toán 8 Tập 2. Bạn có thể rút gọn nếu bạn thích.)
a) - Khi x ≥ 0 ta có 5x ≥ 0 nên |5x| = 5x
Vậy A = 3x + 2 + 5x = 8x + 2
- Khi x < 0 ta có 5x < 0 nên |5x| = -5x
Vậy A = 3x + 2 - 5x = -2x + 2
b) - Khi x ≤ 0 ta có -4x ≥ 0 (nhân hai vế với số âm) nên |-4x| = -4x
Vậy B = -4x - 2x + 12 = -6x + 12
- Khi x > 0 ta có -4x < 0 nên |-4x| = -(-4x) = 4x
Vậy B = 4x - 2x + 12 = 2x + 12
c) - Khi x > 5 ta có x - 4 > 1 (trừ hai vế cho 4) hay x - 4 > 0 nên |x - 4| = x - 4
Vậy C = x - 4 - 2x + 12 = -x + 8
d) D = 3x + 2 + x + 5 khi x + 5 ≥ 0
hoặc D = 3x + 2 - (x + 5) khi x + 5 < 0
Vậy D = 4x + 7 khi x ≥ -5
hoặc D = 2x - 3 khi x < -5
(Bài dưới được trình bày dựa theo cách trình bày ở Ví dụ 1 trang 50 sgk Toán 8 Tập 2. Bạn có thể rút gọn nếu bạn thích.)
a) - Khi x ≥ 0 ta có 5x ≥ 0 nên |5x| = 5x
Vậy A = 3x + 2 + 5x = 8x + 2
- Khi x < 0 ta có 5x < 0 nên |5x| = -5x
Vậy A = 3x + 2 - 5x = -2x + 2
b) - Khi x ≤ 0 ta có -4x ≥ 0 (nhân hai vế với số âm) nên |-4x| = -4x
Vậy B = -4x - 2x + 12 = -6x + 12
- Khi x > 0 ta có -4x < 0 nên |-4x| = -(-4x) = 4x
Vậy B = 4x - 2x + 12 = 2x + 12
c) - Khi x > 5 ta có x - 4 > 1 (trừ hai vế cho 4) hay x - 4 > 0 nên |x - 4| = x - 4
Vậy C = x - 4 - 2x + 12 = -x + 8
d) D = 3x + 2 + x + 5 khi x + 5 ≥ 0
hoặc D = 3x + 2 - (x + 5) khi x + 5 < 0
Vậy D = 4x + 7 khi x ≥ -5
hoặc D = 2x - 3 khi x < -5