Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Câu 2:
c/ DO M thuộc \(\Delta\) nên tọa độ M có dạng \(M\left(a;\frac{1-3a}{2}\right)\)
Áp dụng công thức khoảng cách:
\(\frac{\left|5a-\frac{3\left(1-3a\right)}{2}+2\right|}{\sqrt{5^2+3^2}}=5\)
\(\Leftrightarrow\left|13a+1\right|=10\sqrt{34}\)
\(\Leftrightarrow\left[{}\begin{matrix}13a+1=10\sqrt{34}\\13a+1=-10\sqrt{34}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}a=\frac{-1+10\sqrt{34}}{13}\\a=\frac{-1-10\sqrt{34}}{13}\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}M\left(\frac{-1+10\sqrt{34}}{13};\frac{8-15\sqrt{34}}{13}\right)\\M\left(\frac{-1-10\sqrt{34}}{13};\frac{8+15\sqrt{34}}{13}\right)\end{matrix}\right.\)
d/ Chẳng hiểu đề câu d là gì luôn? Cái gì bằng 2 lần khoảng cách từ N đến d bạn
Câu 2:
a/ Khoảng cách:
\(d\left(A;\Delta\right)=\frac{\left|3.5+2.4-1\right|}{\sqrt{3^2+2^2}}=\frac{22\sqrt{13}}{13}\)
b/ Gọi \(M\left(x;y\right)\) là 1 điểm thuộc đường phân giác
\(\Rightarrow d\left(M;\Delta\right)=d\left(M;d\right)\)
\(\Rightarrow\frac{\left|3x+2y-1\right|}{\sqrt{3^2+2^2}}=\frac{\left|5x-3y+2\right|}{\sqrt{5^2+3^2}}\)
\(\Leftrightarrow\sqrt{34}\left|3x+2y-1\right|=\sqrt{13}\left|5x-3y+2\right|\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{34}\left(3x+2y-1\right)=\sqrt{13}\left(5x-3y+2\right)\\\sqrt{34}\left(3x+2y-1\right)=-\sqrt{13}\left(5x-3y+2\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\left(3\sqrt{34}-5\sqrt{13}\right)x+\left(2\sqrt{34}+3\sqrt{13}\right)y-\sqrt{34}-2\sqrt{13}=0\\\left(3\sqrt{34}+5\sqrt{13}\right)x+\left(2\sqrt{34}-3\sqrt{13}\right)y-\sqrt{34}+2\sqrt{13}=0\end{matrix}\right.\)

Do \(M\in d_3\) \(\Rightarrow M\left(2a;a\right)\)
\(\frac{\left|2a+a+3\right|}{\sqrt{1^2+1^2}}=2\frac{\left|2a-a-4\right|}{\sqrt{1^2+\left(-1\right)^2}}\Leftrightarrow\left|3a+3\right|=2\left|a-4\right|\)
\(\Leftrightarrow\left(3a+3\right)^2=4\left(a-4\right)^2\Leftrightarrow9a^2+18a+9=4a^2-32a+64\)
\(\Leftrightarrow5a^2+50a-55=0\Rightarrow\left[{}\begin{matrix}a=1\\a=-11\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}M\left(2;1\right)\\M\left(-22;-11\right)\end{matrix}\right.\)

Đường tròn (C) tâm \(I\left(1;-2\right)\) bán kính \(R=\sqrt{5}\)
Điểm M thuộc (C) thỏa mãn khoảng cách từ M tới \(\Delta\) lớn nhất khi M là giao điểm của (C) và đường thẳng d qua I và vuông góc \(\Delta\)
Phương trình d có dạng:
\(2\left(x-1\right)-1\left(y+2\right)=0\Leftrightarrow2x-y-4=0\)
Hệ pt tọa độ giao điểm (C) và d:
\(\left\{{}\begin{matrix}x^2+y^2-2x+4y=0\\y=2x-4\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x^2+\left(2x-4\right)^2-2x+4\left(2x-4\right)=0\\y=2x-4\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2-2x=0\\y=2x-4\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}M\left(0;-4\right)\\M\left(2;0\right)\end{matrix}\right.\)
Với \(M\left(0;-4\right)\Rightarrow d\left(M;\Delta\right)=\dfrac{\left|-2.4+7\right|}{\sqrt{1^2+2^2}}=\dfrac{1}{\sqrt{5}}\)
Với \(M\left(2;0\right)\Rightarrow d\left(M;\Delta\right)=\dfrac{\left|2+0+7\right|}{\sqrt{1^2+2^2}}=\dfrac{9}{\sqrt{5}}\)
Do \(\dfrac{9}{\sqrt{5}}>\dfrac{1}{\sqrt{5}}\) nên \(M\left(2;0\right)\) là điểm cần tìm

Đáp án: D
Khoảng cách từ điểm M(-2;1) tới đường thẳng d: x + 3 y + 2 = 0 bằng:
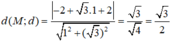

Bài 3:
H thuộc Δ nên H(x;4/5x+3/5)
\(\overrightarrow{AH}=\left(x+1;\dfrac{4}{5}x-\dfrac{12}{5}\right)\)
Δ: 4x-5y+3=0
=>VTPT là (4;-5)
=>VTCP là (5;4)
Theo đề, ta có: 5(x+1)+4(4/5x-12/5)=0
=>5x+5+16/5x-48/5=0
=>31/5x-23/5=0
=>x=23/31
=>y=4/5*23/31+3/5=37/31
a+9b=23/31+9*37/31=356/31

Ta có \(M\in\Delta_1\Rightarrow M\left(2t+3;t\right)\)
.
Khoảng cách từ M đến đường thẳng \(\Delta_2\)bằng \(\dfrac{1}{\sqrt{2}}\)
\(\Rightarrow\)\(d\left(M,\Delta_2\right)=\dfrac{1}{\sqrt{2}}\)
\(\Leftrightarrow\dfrac{\left|2t+3+t+1\right|}{\sqrt{1^2+1^2}}=\dfrac{1}{\sqrt{2}}\)
\(\Leftrightarrow\left|3t+4\right|=1\)\(\Leftrightarrow\left[{}\begin{matrix}t=-1\\t=\dfrac{-5}{3}\end{matrix}\right.\)
* \(t=-1\)
\(\Rightarrow M\left(1;-1\right)\)
*\(t=\dfrac{-5}{3}\)
\(\Rightarrow M\left(\dfrac{-1}{3};\dfrac{-5}{3}\right)\)
