Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Bài 1:
a) x≠2x≠2
Bài 2:
a) x≠0;x≠5x≠0;x≠5
b) x2−10x+25x2−5x=(x−5)2x(x−5)=x−5xx2−10x+25x2−5x=(x−5)2x(x−5)=x−5x
c) Để phân thức có giá trị nguyên thì x−5xx−5x phải có giá trị nguyên.
=> x=−5x=−5
Bài 3:
a) (x+12x−2+3x2−1−x+32x+2)⋅(4x2−45)(x+12x−2+3x2−1−x+32x+2)⋅(4x2−45)
=(x+12(x−1)+3(x−1)(x+1)−x+32(x+1))⋅2(2x2−2)5=(x+12(x−1)+3(x−1)(x+1)−x+32(x+1))⋅2(2x2−2)5
=(x+1)2+6−(x−1)(x+3)2(x−1)(x+1)⋅2⋅2(x2−1)5=(x+1)2+6−(x−1)(x+3)2(x−1)(x+1)⋅2⋅2(x2−1)5
=(x+1)2+6−(x2+3x−x−3)(x−1)(x+1)⋅2(x−1)(x+1)5=(x+1)2+6−(x2+3x−x−3)(x−1)(x+1)⋅2(x−1)(x+1)5
=[(x+1)2+6−(x2+2x−3)]⋅25=[(x+1)2+6−(x2+2x−3)]⋅25
=[(x+1)2+6−x2−2x+3]⋅25=[(x+1)2+6−x2−2x+3]⋅25
=[(x+1)2+9−x2−2x]⋅25=[(x+1)2+9−x2−2x]⋅25
=2(x+1)25+185−25x2−45x=2(x+1)25+185−25x2−45x
=2(x2+2x+1)5+185−25x2−45x=2(x2+2x+1)5+185−25x2−45x
=2x2+4x+25+185−25x2−45x=2x2+4x+25+185−25x2−45x
=2x2+4x+2+185−25x2−45x=2x2+4x+2+185−25x2−45x
=2x2+4x+205−25x2−45x=2x2+4x+205−25x2−45x
c) tự làm, đkxđ: x≠1;x≠−1

a.ĐKXĐ \(\hept{\begin{cases}x\ne-3\\x\ne2\end{cases}}\)
A=\(\frac{x+2}{x+3}-\frac{5}{\left(x+3\right)\left(x-2\right)}-\frac{1}{x-2}\)
=\(\frac{\left(x+2\right)\left(x-2\right)-5-\left(x+3\right)}{\left(x+3\right)\left(x-2\right)}=\frac{x^2-x-12}{\left(x+3\right)\left(x-2\right)}=\frac{\left(x-4\right)\left(x+3\right)}{\left(x+3\right)\left(x-2\right)}\)
=\(\frac{x-4}{x-2}\)
b. Để A >0 thì \(\frac{x-4}{x-2}\) >0 \(\Rightarrow\orbr{\begin{cases}x< 2\\x>4\end{cases}}\)
Kết hợp ĐK thì \(\orbr{\begin{cases}x< 2,x\ne-3\\x>4\end{cases}}\)
c. \(A=\frac{x-4}{x-2}=1+\frac{-2}{x-2}\)
Để A nguyên thì \(x-2\inƯ\left(-2\right)=\left\{-2;-1;1;2\right\}\)
\(\Rightarrow x\in\left\{0,1,3,4\right\}\)
Khi thay vào A, để A dương thì \(x\in\left\{0;1\right\}\)
Vậy để A nguyên dương thì \(x\in\left\{0;1\right\}\)
Câu c, có thể nói kết hợp với điều kiện giải được trong câu b, ta tìm được \(x\in\left\{0;1\right\}\)

Cái biểu thức A ban ghi rõ thì mình mới giải được chứ , ghi như thế ai hiểu mà giải.

a) xác định khi x khác +-1
b)
\(A=\left(\frac{\left(2x+1\right).\left(x+1\right)}{\left(x-1\right)\left(x+1\right)}+\frac{8}{\left(x-1\right)\left(x+1\right)}-\frac{\left(x-1\right)\left(x-1\right)}{\left(x-1\right)\left(x+1\right)}\right).\frac{\left(x-1\right)}{\left(x+1\right)}\)
\(A=\left(\frac{\left(2x^2+3x+1\right)+8-\left(x^2-2x+1\right)}{\left(x-1\right)\left(x+1\right)}\right).\frac{\left(x-1\right)}{\left(x+1\right)}=\frac{x^2+5x+8}{\left(x-1\right)\left(x+1\right)}.\frac{x-1}{x+1}\)
\(A=\frac{x^2+5x+8}{\left(x+1\right)^2}=1+\frac{3\left(x+1\right)+4}{\left(x+1\right)^2}\)
c)
GTNN \(B=\frac{3y+4}{y^2}\ge-\frac{9}{16}\)
GTNN \(A=\frac{7}{16}\)

Câu 1:
\(Tacó\)
\(\frac{2}{2x-1}+\frac{4x^2+1}{4x^2-1}-\frac{1}{2x+1}=\frac{2}{2x-1}+\frac{4x^2+1}{\left(2x+1\right)\left(2x-1\right)}-\frac{1}{2x+1}\)
\(=\frac{4x+2}{\left(2x+1\right)\left(2x-1\right)}+\frac{4x^2+1}{\left(2x+1\right)\left(2x-1\right)}-\frac{2x-1}{\left(2x+1\right)\left(2x-1\right)}\)
\(=\frac{4x+2+4x^2+1-2x+1}{\left(2x+1\right)\left(2x-1\right)}=\frac{2x\left(2x+1\right)+4}{\left(2x+1\right)\left(2x-1\right)}=\frac{2x+4}{2x-1}\)
\(b,x=\frac{1}{2}\Rightarrow2x-1=0\left(loại\right)\)
..... 2 câu sau easy

Bài 1:
a) \(x\ne2\)
Bài 2:
a) \(x\ne0;x\ne5\)
b) \(\dfrac{x^2-10x+25}{x^2-5x}=\dfrac{\left(x-5\right)^2}{x\left(x-5\right)}=\dfrac{x-5}{x}\)
c) Để phân thức có giá trị nguyên thì \(\dfrac{x-5}{x}\) phải có giá trị nguyên.
=> \(x=-5\)
Bài 3:
a) \(\left(\dfrac{x+1}{2x-2}+\dfrac{3}{x^2-1}-\dfrac{x+3}{2x+2}\right)\cdot\left(\dfrac{4x^2-4}{5}\right)\)
\(=\left(\dfrac{x+1}{2\left(x-1\right)}+\dfrac{3}{\left(x-1\right)\left(x+1\right)}-\dfrac{x+3}{2\left(x+1\right)}\right)\cdot\dfrac{2\left(2x^2-2\right)}{5}\)
\(=\dfrac{\left(x+1\right)^2+6-\left(x-1\right)\left(x+3\right)}{2\left(x-1\right)\left(x+1\right)}\cdot\dfrac{2\cdot2\left(x^2-1\right)}{5}\)
\(=\dfrac{\left(x+1\right)^2+6-\left(x^2+3x-x-3\right)}{\left(x-1\right)\left(x+1\right)}\cdot\dfrac{2\left(x-1\right)\left(x+1\right)}{5}\)
\(=\left[\left(x+1\right)^2+6-\left(x^2+2x-3\right)\right]\cdot\dfrac{2}{5}\)
\(=\left[\left(x+1\right)^2+6-x^2-2x+3\right]\cdot\dfrac{2}{5}\)
\(=\left[\left(x+1\right)^2+9-x^2-2x\right]\cdot\dfrac{2}{5}\)
\(=\dfrac{2\left(x+1\right)^2}{5}+\dfrac{18}{5}-\dfrac{2}{5}x^2-\dfrac{4}{5}x\)
\(=\dfrac{2\left(x^2+2x+1\right)}{5}+\dfrac{18}{5}-\dfrac{2}{5}x^2-\dfrac{4}{5}x\)
\(=\dfrac{2x^2+4x+2}{5}+\dfrac{18}{5}-\dfrac{2}{5}x^2-\dfrac{4}{5}x\)
\(=\dfrac{2x^2+4x+2+18}{5}-\dfrac{2}{5}x^2-\dfrac{4}{5}x\)
\(=\dfrac{2x^2+4x+20}{5}-\dfrac{2}{5}x^2-\dfrac{4}{5}x\)
c) tự làm, đkxđ: \(x\ne1;x\ne-1\)
ô hô ngộ quá nhìu người bt toán lớp 8 trong khi lớp 7 với lại óc nguyow trở lại r kaka
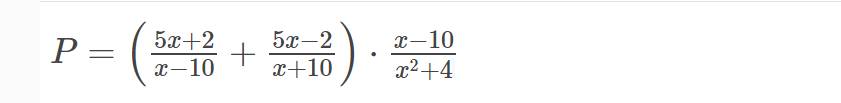
 Câu1:Điền vào...để có hăng đẳng thức đáng nhớ
Câu1:Điền vào...để có hăng đẳng thức đáng nhớ
Bài 2
a) ĐKXĐ: x - 10 0 và x + 10 0
*) x - 10 0
x 10
*) x + 10 0
x 10
Vậy ĐKXĐ: x -10; x 10
b) P = [(5x + 2)(x + 10) + (5x - 2)(x - 10)]/[(x - 10)(x + 10)] . (x - 10)/(x² + 4)
= (5x² + 50x + 2x + 20 + 5x² - 50x - 2x + 20)/[(x + 10)(x² + 4)]
= (10x² + 40)/[(x + 10)(x² + 4)]
= 10(x² + 4)/[(x + 10)(x² + 4)]
= 10/(x + 10)
c) Khi x = 2/5 ta có:
P = 10.(2/5 + 10)
= 4 + 100
= 104
giúp mik vs