Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) \(\dfrac{\sqrt{2}}{\sqrt{3}}+2.\dfrac{\sqrt{3}}{\sqrt{2}}-\sqrt{6}=\dfrac{\sqrt{2}}{\sqrt{3}}+\dfrac{\sqrt{2}.\sqrt{2}.\sqrt{3}}{\sqrt{2}}-\sqrt{6}=\dfrac{\sqrt{2}}{\sqrt{3}}+\sqrt{6}-\sqrt{6}=\dfrac{\sqrt{2}}{\sqrt{3}}\)
b)
\(3\dfrac{\sqrt{2}}{\sqrt{5}}+\dfrac{\sqrt{5}}{\sqrt{2}}-2\sqrt{10}=3\dfrac{\sqrt{2}.\sqrt{5}}{5}+\dfrac{\sqrt{5}.\sqrt{2}}{2}-2\sqrt{10}\)\(=\sqrt{10}.\left[\dfrac{3}{5}+\dfrac{1}{2}-2\right]=\sqrt{10}.\left(-\dfrac{9}{10}\right)=\dfrac{-9\sqrt{10}}{10}\)
c)
\(\dfrac{-\sqrt{3}}{\sqrt{5}}+3.\dfrac{\sqrt{5}}{\sqrt{3}}-4\sqrt{15}=\dfrac{-\sqrt{15}}{5}+3.\dfrac{\sqrt{15}}{3}-4\sqrt{15}=\sqrt{15}.\left(\dfrac{-1}{5}+1-4\right)=\sqrt{15}.\left(-\dfrac{16}{5}\right)=\dfrac{-16\sqrt{15}}{5}\)
d)\(\dfrac{2\left(\sqrt{6}+2\right)}{\left(\sqrt{6}-2\right)\left(\sqrt{6}+2\right)}+\dfrac{2\left(\sqrt{6}-2\right)}{\left(\sqrt{6}-2\right)\left(\sqrt{6}+2\right)}+\dfrac{5\sqrt{6}}{6}\)
\(=\dfrac{2\left[\left(\sqrt{6}+2\right)+\left(\sqrt{6}-2\right)\right]}{6-4}+\dfrac{5\sqrt{6}}{6}=\left(2\sqrt{6}\right)+\dfrac{5\sqrt{6}}{6}=\dfrac{17\sqrt{6}}{6}\)
Kiểm tra lại nhé ^^

a: \(=\dfrac{6}{4+\sqrt{3}-1}=\dfrac{6}{3+\sqrt{3}}=3-\sqrt{3}\)
b: \(=\left(\dfrac{\sqrt{6}\left(\sqrt{2}-1\right)}{2\left(\sqrt{2}-1\right)}-2\sqrt{6}\right)\cdot\dfrac{1}{\sqrt{6}}=\sqrt{6}\cdot\dfrac{1}{\sqrt{6}}\left(\dfrac{1}{2}-2\right)=-\dfrac{3}{2}\)

Bài 2:
\(P=\left(\dfrac{\sqrt{a}}{2}-\dfrac{1}{2\sqrt{a}}\right)^2.\left(\dfrac{\sqrt{a}-1}{\sqrt{a}+1}-\dfrac{\sqrt{a}+1}{\sqrt{a}-1}\right)\)
\(P=\left(\dfrac{a-1}{2\sqrt{a}}\right)^2.\left(\dfrac{\left(\sqrt{a}-1\right)^2-\left(\sqrt{a}+1\right)^2}{\left(\sqrt{a}+1\right)\left(\sqrt{a}-1\right)}\right)\)
\(P=\left[\dfrac{\left(a-1\right)^2}{4a}\right].\left(\dfrac{\left(\sqrt{a}-1+\sqrt{a}+1\right)\left(\sqrt{a}-1\right)-\sqrt{a}-1}{a-1}\right)\)
\(P=\dfrac{\left(a-1\right)^2}{4a}.\dfrac{2\sqrt{a}.\left(-2\right)}{a-1}\)
\(P=\dfrac{\left(a-1\right)^2\left(-4\sqrt{a}\right)}{4a.\left(a-1\right)}\)
\(P=\dfrac{\left(a-1\right).\left(-\sqrt{a}\right)}{a}=\dfrac{-a\sqrt{a}+\sqrt{a}}{a}\)
Bài 1:
\(A=\dfrac{2}{\sqrt{2}}-\dfrac{1}{\sqrt{3}-\sqrt{2}}+\dfrac{2}{\sqrt{3}-1}\)\(A=\dfrac{2\sqrt{2}}{2}-\dfrac{1\left(\sqrt{3}+\sqrt{2}\right)}{3-2}+\dfrac{2\left(\sqrt{3}+1\right)}{\left(\sqrt{3}\right)^2-1}\)
\(A=\sqrt{2}-\dfrac{\sqrt{3}+\sqrt{2}}{1}+\dfrac{2\left(\sqrt{3}+1\right)}{3-1}\)
\(A=\sqrt{2}-\sqrt{3}-\sqrt{2}+\sqrt{3}+1\)
\(A=1\)

bài 2:
a: \(\dfrac{25}{5-2\sqrt{3}}=\dfrac{125+10\sqrt{3}}{13}\)
b: \(\dfrac{8}{\sqrt{5}+2}=8\sqrt{5}-32\)
c: \(\dfrac{6}{2\sqrt{3}-\sqrt{7}}=\dfrac{12\sqrt{3}+6\sqrt{7}}{5}\)
d: \(=\dfrac{\sqrt{3}\left(3\sqrt{3}-2\right)}{\sqrt{2}\left(3\sqrt{3}-2\right)}=\dfrac{\sqrt{6}}{2}\)

Nhận xét: Cách làm thứ nhật (nhận dạng tử có thể phân tích thành nhân tử để rút gọn nhân tử đó với mẫu thích hợp hơn cách làm thứ hai (trục căn thức ở mẫu rồi thu gọn). Vì trục căn thức ở mẫu rồi rút gọn sẽ thêm nhiều phép nhân.
Nhận xét: Cách làm thứ nhật (nhận dạng tử có thể phân tích thành nhân tử để rút gọn nhân tử đó với mẫu thích hợp hơn cách làm thứ hai (trục căn thức ở mẫu rồi thu gọn). Vì trục căn thức ở mẫu rồi rút gọn sẽ thêm nhiều phép nhân.

a) \(\dfrac{30\left(\sqrt{6}-1\right)}{6-1}+\dfrac{2\left(\sqrt{6}+2\right)}{6-4}-\dfrac{6\left(3+\sqrt{6}\right)}{9-6}\)
= \(6\sqrt{6}-6+\sqrt{6}+2-6-2\sqrt{6}=5\sqrt{6}+2\)
b) \(\dfrac{\sqrt{6+2\sqrt{5}}}{2}-\dfrac{\sqrt{5}-1}{2}=\dfrac{\sqrt{5}+1-\sqrt{5}+1}{2}=1\)
c: \(=-\sqrt{3}+\dfrac{4+2\sqrt{3}}{2}=\dfrac{-2\sqrt{3}+4+2\sqrt{3}}{2}=\dfrac{4}{2}=2\)
a: \(=6\left(\sqrt{6}-1\right)+\sqrt{6}+2-6-2\sqrt{6}\)
\(=6\sqrt{6}-6-\sqrt{6}-4=5\sqrt{6}-10\)
b:\(=\dfrac{\sqrt{6+2\sqrt{5}}}{2}-\dfrac{\sqrt{5}-1}{2}\)
\(=\dfrac{\sqrt{5}+1-\sqrt{5}+1}{2}=\dfrac{2}{2}=1\)

Bài 1 bạn nhóm , trục như thường nhé :D
Bài 2. \(a.A=\sqrt{5+2\sqrt{6}}-\sqrt{5-2\sqrt{6}}=\sqrt{3+2\sqrt{3}.\sqrt{2}+2}-\sqrt{3-2\sqrt{3}.\sqrt{2}+2}=\sqrt{3}+\sqrt{2}-\sqrt{3}+\sqrt{2}=2\sqrt{2}\)
\(b.B=\sqrt{17-12\sqrt{2}}-\sqrt{9+4\sqrt{2}}=\sqrt{9-2.2\sqrt{2}.3+8}-\sqrt{8+2.2\sqrt{2}+1}=3-2\sqrt{2}-2\sqrt{2}-1=2-4\sqrt{2}\)
\(c.C=\sqrt{13+30\sqrt{2+\sqrt{9+4\sqrt{2}}}}=\sqrt{13+30\sqrt{2+\sqrt{8+2.2.\sqrt{2}+1}}}=\sqrt{13+30\sqrt{2+2\sqrt{2}+1}}=\sqrt{43+30\sqrt{2}}=\sqrt{25+2.3\sqrt{2}.5+18}=5+3\sqrt{2}\)
\(d.D=\sqrt{12-3\sqrt{7}}-\sqrt{12+3\sqrt{7}}\)
\(D^2=24-2\sqrt{\left(12-3\sqrt{7}\right)\left(12+3\sqrt{7}\right)}=24-2\sqrt{81}=24-18=6\)
\(D=-\sqrt{6}\left(do:D< 0\right)\)

\(\dfrac{1}{3-\sqrt{8}}-\dfrac{1}{\sqrt{8}-\sqrt{7}}+\dfrac{1}{\sqrt{7}-\sqrt{6}}-\dfrac{1}{\sqrt{6}-\sqrt{5}}+\dfrac{1}{\sqrt{5}-2}\)
\(=\dfrac{3+\sqrt{8}}{9-8}-\dfrac{\sqrt{8}+\sqrt{7}}{8-7}+\dfrac{\sqrt{7}+\sqrt{6}}{7-6}-\dfrac{\sqrt{6}+\sqrt{5}}{6-5}+\dfrac{\sqrt{5}+2}{5-4}\)
\(=3+\sqrt{8}-\sqrt{8}-\sqrt{7}+\sqrt{7}+\sqrt{6}-\sqrt{6}-\sqrt{5}+\sqrt{5}+2\)
\(=3+2=5\)
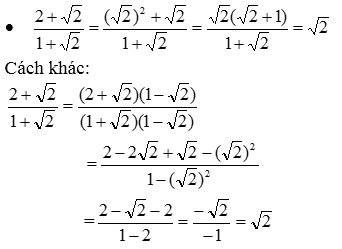
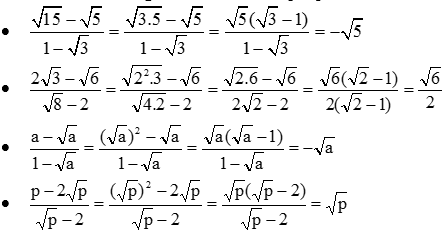


Sửa đề: \(B=\dfrac{2}{\sqrt{6}-2}+\dfrac{2}{\sqrt{6}+2}-\dfrac{5\sqrt{6}}{2}\)
Ta có: \(B=\dfrac{2}{\sqrt{6}-2}+\dfrac{2}{\sqrt{6}+2}-\dfrac{5\sqrt{6}}{2}\)
\(=\dfrac{2\left(\sqrt{6}+2\right)+2\left(\sqrt{6}-2\right)}{\left(\sqrt{6}-2\right)\left(\sqrt{6}+2\right)}-\dfrac{5\sqrt{6}}{2}\)
\(=\dfrac{2\sqrt{6}+4+2\sqrt{6}-4}{2}-\dfrac{5\sqrt{6}}{2}\)
\(=-\dfrac{\sqrt{6}}{2}\)
bạn làm sai rồi đề là 3 mà bạn