Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
ĐKXĐ: \(x\ne k\dfrac{\pi}{2}\)
\(tanx+\dfrac{1}{tanx}=2\)
\(\Rightarrow tan^2x+1=2tanx\)
\(\Leftrightarrow\left(tanx-1\right)^2=0\)
\(\Leftrightarrow tanx=1\)
\(\Rightarrow x=\dfrac{\pi}{4}+k\pi\) (thỏa mãn)
Lời giải:
$\tan 3x-\tan x=2$
$\Leftrightarrow \frac{3\tan x-\tan ^3x}{1-3\tan ^2x}-\tan x=2$
Đặt $\tan x=a$ thì:
$\frac{3a-a^3}{1-3a^2}-a=2$
$\Leftrightarrow a^3+3a^2+a-1=0$
$\Leftrihgtarrow a^2(a+1)+2a(a+1)-(a+1)=0$
$\Leftrightarrow (a+1)(a^2+2a-1)=0$
$\Leftrightarrow a=-1$ hoặc $a=-1\pm \sqrt{2}$
Đến đây thì đơn giản rồi.
ĐKXĐ: \(\left\{{}\begin{matrix}x\ne\dfrac{\pi}{2}+k\pi\\x\ne\dfrac{\pi}{6}+\dfrac{k\pi}{3}\end{matrix}\right.\)
\(\dfrac{sin3x}{cos3x}-\dfrac{sinx}{cosx}=2\)
\(\Rightarrow sin3x.cosx-cos3x.sinx=2cos3x.cosx\)
\(\Leftrightarrow sin2x=cos4x-cos2x\)
\(\Leftrightarrow cos^22x-sin^22x-sin2x-cos2x=0\)
\(\Leftrightarrow\left(sin2x+cos2x\right)\left(cos2x-sin2x-1\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}\sqrt{2}sin\left(2x+\dfrac{\pi}{4}\right)=0\\cos\left(2x+\dfrac{\pi}{4}\right)=\dfrac{\sqrt{2}}{2}\end{matrix}\right.\)
\(\Leftrightarrow...\)
ĐK: \(cosx\ne0\Leftrightarrow x\ne\frac{\pi}{2}+k\pi,k\inℤ\).
\(1+tanx=2\left(sinx+cosx\right)\)
\(\Leftrightarrow cosx+sinx=2cosx\left(sinx+cosx\right)\)
\(\Leftrightarrow\orbr{\begin{cases}sinx+cosx=0\\cosx=\frac{1}{2}\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}cosx=cos\left(-x-\frac{\pi}{2}\right)\\cosx=cos\frac{\pi}{3}\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=\pm\left(-x-\frac{\pi}{2}\right)+k2\pi\\x=\pm\frac{\pi}{3}+k2\pi\end{cases}},\left(k\inℤ\right)\)
\(\Leftrightarrow\orbr{\begin{cases}x=\frac{-\pi}{4}+k\pi\\x=\pm\frac{\pi}{3}+k2\pi\end{cases}},\left(k\inℤ\right)\)(thỏa mãn)
\(1+\tan x=2\left(\sin x+\cos x\right)\)
Bạn áp dụng đẳng thức lượng giác nhé :
\(\frac{\sin x+\cos x}{\cos x}=2\sin x+2\cos x\)
Biệt thức :
\(D=b^2-4ac\)
\(\Leftrightarrow\left(-1\right)^2-4\left(1.1\right)=-3\)
Phương trình không có nghiệm thực :
\(D< 0\)
Nghiệm tuần hoàn :
\(2\pi k-\frac{\pi}{4}\)
\(2\pi k+\frac{3\pi}{4}\)
\(2\pi k+\frac{\pi}{3}\)
\(2\pi k-\frac{\pi}{3}\)
Ps : không hiểu chỗ nào thì bạn hỏi mình nhé, nhớ k :33
# Aeri #
Đối với những phương trình lượng giác chứa tanx, cotx, sin2x hoặc cos2x, ta có thể đưa về phương trình chứa cosx, sinx, sin2x, hoặc cos2x ngoài ra cũng có thể đặt ẩn phụ t = tanx để đưa về một phương trình theo t.
Cách 1: Điều kiện của phương trình:
sin2x ≠ 0 ⇔ cos2x ≠ 1 hoặc cos2x ≠ -1 (1)
Ta có:
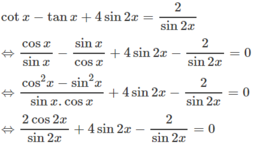
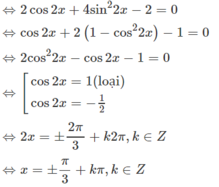
Cách 2. Đặt t = tanx
Điều kiện t ≠ 0
Phương trình đã cho có dạng
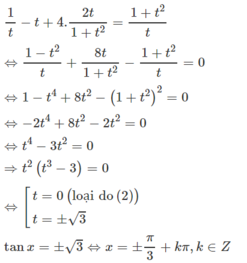
Điều kiện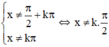 
tanx – 2.cotx + 1 = 0
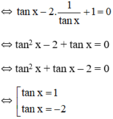
(Thỏa mãn điều kiện).
Vậy phương trình có tập nghiệm
{
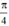+ kπ; arctan(-2) + kπ} (k ∈ Z)
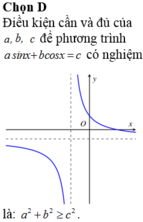
\(sinx=\dfrac{2tan\dfrac{x}{2}}{tan^2\dfrac{x}{2}+1}\)
\(cosx=\dfrac{1-tan^2\dfrac{x}{2}}{1+tan^2\dfrac{x}{2}}\)
Đặt \(t=tan\dfrac{x}{2}\)
Khi đó pt: \(\Rightarrow a\cdot\dfrac{2t}{t^2+1}+b\cdot\dfrac{1-t^2}{1+t^2}=c\)
\(\Rightarrow2t\cdot a+\left(1-t^2\right)\cdot b=\left(1+t^2\right)\cdot c\)