K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
Giải bài giúp em vs ạ em cần gấp lắm cảm ơn
2
29 tháng 9 2023
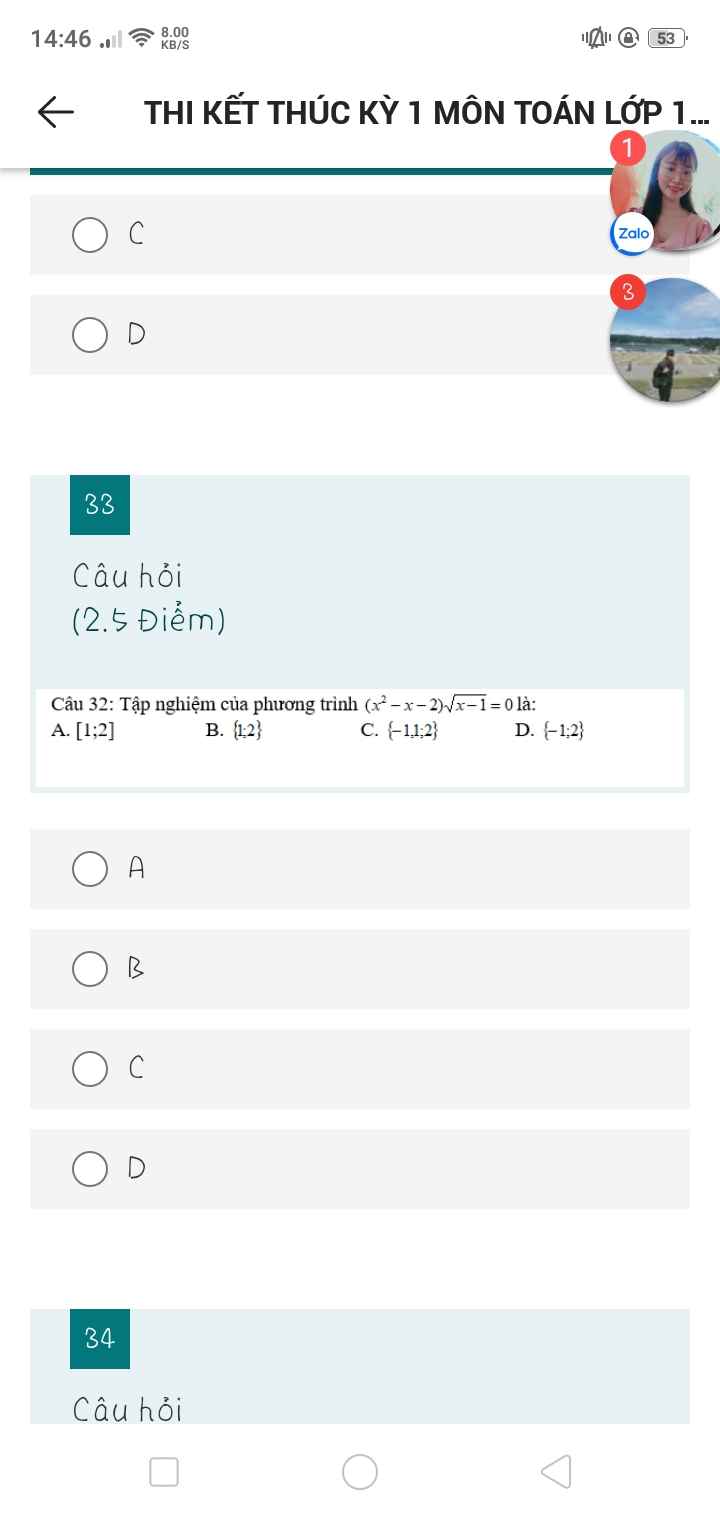
`2)`
`@` Xét `3x+6 >= 0<=>x >= -2`
`=>A=[-2;+oo)`
`@` Xét `|x-2| < 3`
`<=>-3 < x-2 < 3`
`<=>-1 < x < 5=>B=(-1;5)`
Có: `A nn B=(-1;5)`
`A uu B=[-2;+oo)`
`R \\ B=(-oo;-1]uu[5;+oo)`
_______
`3)`
`@` Xét `x+3 >= 2x+7<=>x <= -4=>A=(-oo;-4]`
`@` Xét `4x+5 > 0<=>x > -5/4=>B=(-5/4;+oo)`
`@` Xét `|x+4| < 2<=>-2 < x+4 < 2<=>-6 < x < -2 =>C=(-6;-2)`
Có: `A nn B nn C=\emptyset`
`A \\ B nn C=(-6;-4]`
`C \\ A nn B=\emptyset`.
H9
HT.Phong (9A5)
CTVHS
27 tháng 9 2023
Bài 4:
Theo định lý sin ta có:
\(\dfrac{AC}{sinB}=\dfrac{BC}{sinA}\)
\(\Rightarrow BC=a=\dfrac{b\cdot sinA}{sinB}=\dfrac{2\cdot sin60^o}{sin45^o}=\sqrt{6}\)
\(\Rightarrow\widehat{C}=180^o-60^o-45^o=75^o\)
\(\dfrac{AC}{sinB}=\dfrac{AB}{sinC}\)
\(\Rightarrow AB=c=\dfrac{b\cdot sinC}{sinB}=\dfrac{2\cdot sin75^o}{sin45^o}=1+\sqrt{3}\)
Diện tích tam giác ABC là:
\(S_{ABC}=\dfrac{1}{2}\cdot AC\cdot AB\cdot sinA=\dfrac{1}{2}\cdot2\cdot\left(1+\sqrt{3}\right)\cdot sin75^o=\dfrac{\sqrt{6}+2\sqrt{2}}{2}\) (đvdt)
Bán kình hình tròn tam giác ABC khi đó là:
\(S_{ABC}=\dfrac{abc}{4R}\)
\(\Rightarrow R=\dfrac{abc}{4S_{ABC}}=\dfrac{2\cdot\left(1+\sqrt{3}\right)\cdot\sqrt{6}}{4\cdot\left(\dfrac{\sqrt{6}+2\sqrt{2}}{2}\right)}=3-\sqrt{3}\)
H9
HT.Phong (9A5)
CTVHS
27 tháng 9 2023
Bài 3:
a) Xét tam giác ABC theo định lý côsin ta có:
\(cosC=\dfrac{a^2+b^2-c^2}{2ab}=\dfrac{8^2+10^2-13^2}{2\cdot8\cdot10}=-0,03125\)
\(\Rightarrow\widehat{C}=cos^{-1}-0,03125\approx91^o>90^o\)
Nên tam giác ABC có góc C là góc tù
c) Theo hệ thức Heron ta có diện tích tam giác ABC là:
\(S_{ABC}=\sqrt{p\cdot\left(p-a\right)\cdot\left(p-b\right)\cdot\left(p-c\right)}\)
\(\Rightarrow S_{ABC}=\sqrt{\dfrac{8+10+13}{2}\cdot\left(\dfrac{8+10+13}{2}-8\right)\cdot\left(\dfrac{8+10+13}{2}-10\right)\cdot\left(\dfrac{8+10+13}{2}-13\right)}\)
\(\Rightarrow S_{ABC}\approx40\) (đvdt)
b) Bán kính đường tròn ngoại tiếp tam giác ABC là:
\(S_{ABC}=\dfrac{abc}{4R}\)
\(\Rightarrow R=\dfrac{abc}{4S_{ABC}}=\dfrac{8\cdot10\cdot13}{4\cdot40}=6,5\)
16 tháng 9 2021
a)\(A=\left\{2,3,4,5\right\}\)
b)\(B=\left\{-2,-1,0,1,2\right\}\)
c)\(C=\left\{-3;0;3;6;9\right\}\)
d)\(A=\left\{3;4;7;12;19\right\}\)
16 tháng 9 2021
a)\(n\in\)\(N^*\); \(3< n^2< 30\Leftrightarrow\sqrt{3}< n< \sqrt{30}\)
\(\Rightarrow n=\left\{2;3;4;5\right\}\)
\(\Rightarrow A=\left\{2;3;4;5\right\}\)
b)\(\left|n\right|< 3\Leftrightarrow-3< n< 3\) mà \(n\in Z\)
\(\Rightarrow n=\left\{-2;-1;0;1;2\right\}\)
\(\Rightarrow B=\left\{-2;-1;0;1;2\right\}\)
c)Các phần tử của C là x ; x=3k với k nguyên và thỏa mãn \(-4< x< 12\)
\(\Rightarrow x=\left\{-3;0;3;6;9\right\}\) (với các k lần lượt là \(-1;0;1;2;3\))
\(\Rightarrow C=\left\{-3;0;3;6;9\right\}\)
d)Các phần tử của A có dạng \(n^2+3\) với \(n\in N;n< 5\Rightarrow n=\left\{0;1;2;3;4\right\}\)
\(\Rightarrow A=\left\{3;4;7;12;19\right\}\)
NV
Nguyễn Việt Lâm
Giáo viên
11 tháng 4 2022
a.
\(0< a< \dfrac{\pi}{2}\Rightarrow cosa>0\Rightarrow cosa=\sqrt{1-sin^2a}=\dfrac{4}{5}\)
\(\Rightarrow tana=\dfrac{sina}{cosa}=\dfrac{3}{4}\) ; \(cota=\dfrac{1}{tana}=\dfrac{4}{3}\)
\(\Rightarrow A=\dfrac{\dfrac{4}{3}+\dfrac{3}{4}}{\dfrac{4}{3}-\dfrac{3}{4}}=...\)
b.
\(A=\dfrac{\dfrac{2sina}{cosa}+\dfrac{3cosa}{cosa}}{\dfrac{4sina}{cosa}-\dfrac{5cosa}{cosa}}=\dfrac{2tana+3}{4tana-5}=\dfrac{2.3+3}{4.3-5}=...\)
\(B=\dfrac{\dfrac{3sina}{cos^3a}-\dfrac{2cosa}{cos^3a}}{\dfrac{5sin^3a}{cos^3a}+\dfrac{4cos^3a}{cos^3a}}=\dfrac{3tana\left(1+tan^2a\right)-2\left(1+tan^2a\right)}{5tan^3a+4}=...\) em tự thay số
c.
\(B=\dfrac{cos^2x+2sinx.cosx+1}{sin^2x+3}=\dfrac{\dfrac{cos^2x}{cos^2x}+\dfrac{2sinx.cosx}{cos^2x}+\dfrac{1}{cos^2x}}{\dfrac{sin^2x}{cos^2x}+\dfrac{3}{cos^2x}}\)
\(=\dfrac{1+2tanx+\left(1+tan^2x\right)}{tan^2x+3\left(1+tan^2x\right)}=...\)
6 tháng 2 2023
Bài 1:
a: Đặt 14,4-3,6t^2=0
=>3,6t^2=14,4
=>t^2=4
=>t=2
b: TXĐ: [0;2]
TGT: [0;14,4]
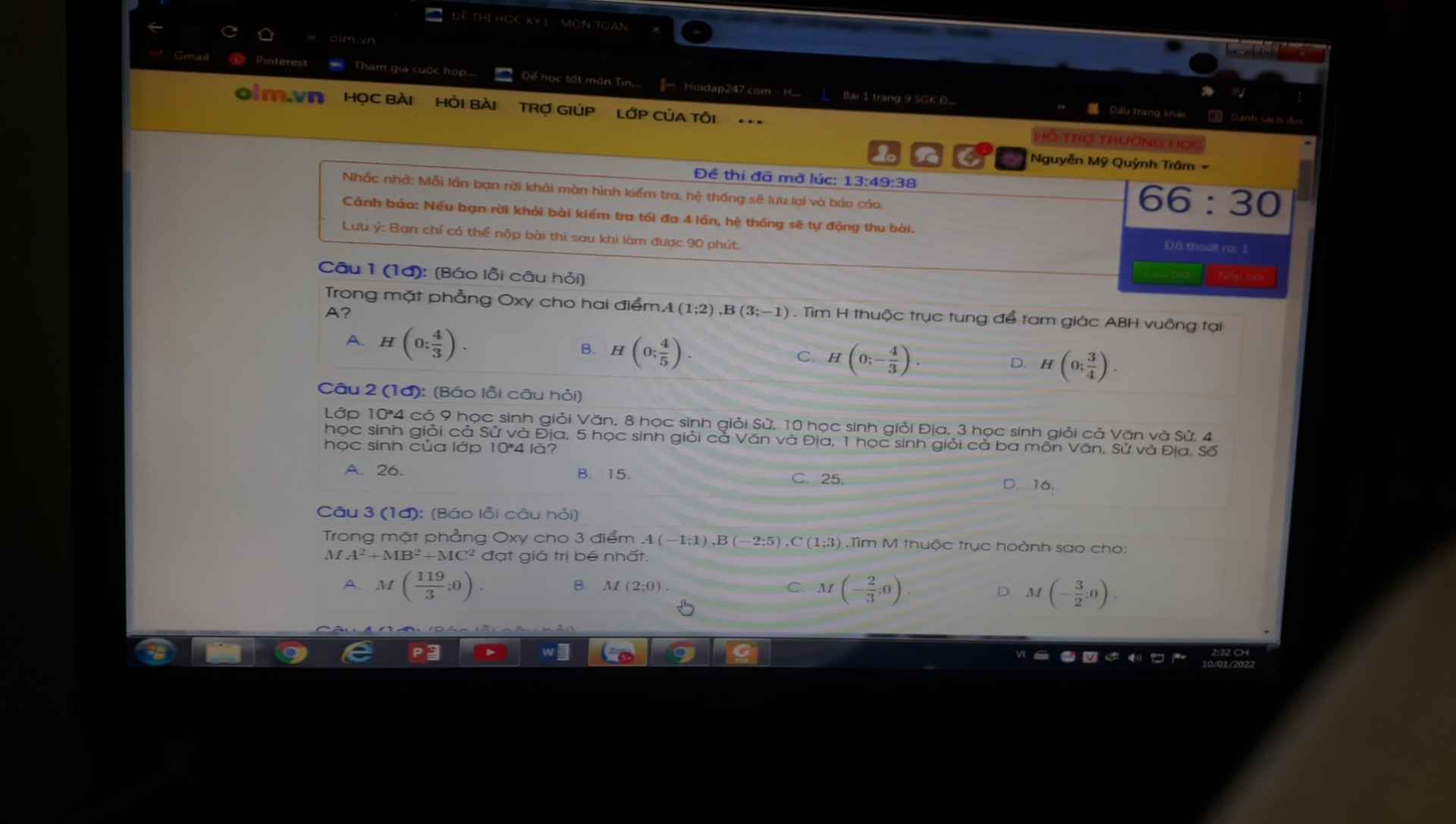
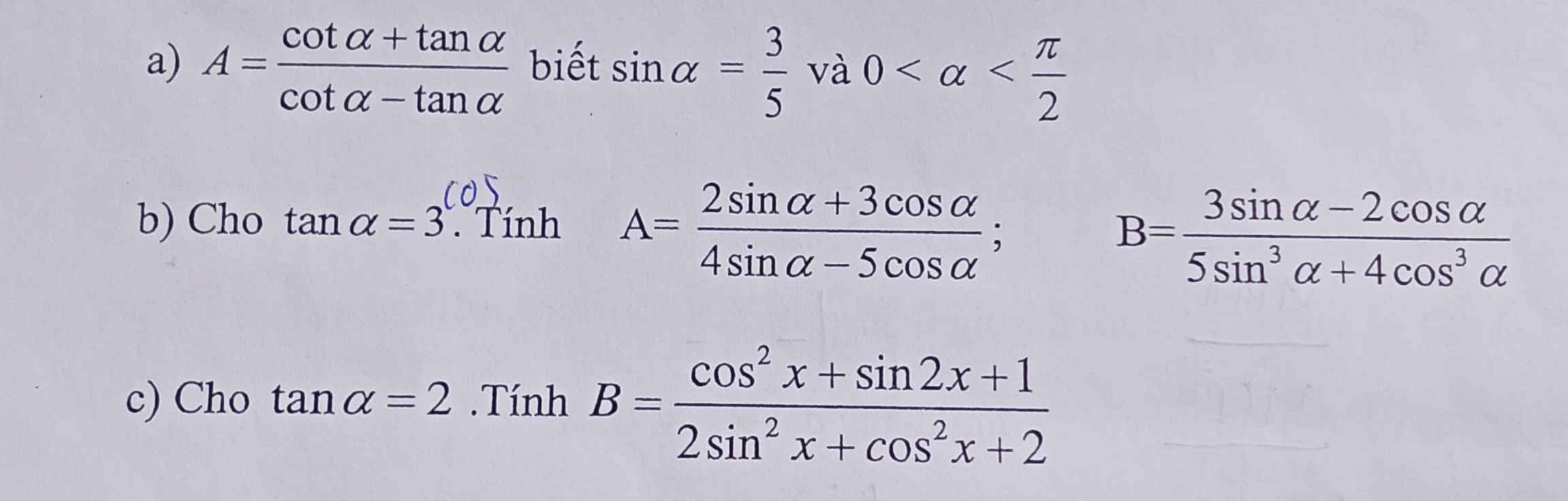
\(\left(x^2-x-2\right)\sqrt{x-1}=0\left(đk:x\ge1\right)\)
\(\Leftrightarrow\left(x-2\right)\left(x+1\right)\sqrt{x-1}=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=1\end{matrix}\right.\) (do x+1>0)
Ý B.
B