
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Mình xin không viết lại điều kiện , tại đề có rồi
\(A=\left(\dfrac{a\sqrt{a}+b\sqrt{b}}{\sqrt{a}+\sqrt{b}}-\sqrt{ab}\right)\left(\dfrac{\sqrt{a}+\sqrt{b}}{\sqrt{a}-\sqrt{b}}\right)=\left(a+b-\sqrt{ab}-\sqrt{ab}\right)\cdot\dfrac{\sqrt{a}+\sqrt{b}}{\sqrt{a}-\sqrt{b}}\\ =\left(\sqrt{a}-\sqrt{b}\right)^2\cdot\dfrac{\sqrt{a}+\sqrt{b}}{\sqrt{a}-\sqrt{b}}=\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{a}-\sqrt{b}\right)=a-b\)\(B=\left(\dfrac{a\sqrt{a}-b\sqrt{b}}{\sqrt{a}-\sqrt{b}}+\sqrt{ab}\right):\left(\sqrt{a}+\sqrt{b}\right)^2=\left(a+b+\sqrt{ab}+\sqrt{ab}\right):\left(\sqrt{a}+\sqrt{b}\right)^2=\left(\sqrt{a}+\sqrt{b}\right)^2:\left(\sqrt{a}+\sqrt{b}\right)^2=1\)
\(C=\dfrac{x-y}{\sqrt{x}-\sqrt{y}}-\dfrac{\sqrt{x^3}-\sqrt{y^3}}{x+\sqrt{xy}+y}-2\sqrt{y}=\sqrt{x}+\sqrt{y}-\left(\sqrt{x}-\sqrt{y}\right)-2\sqrt{y}=0\)
a) Ta có: \(A=\left(\dfrac{a\sqrt{a}+b\sqrt{b}}{\sqrt{a}+\sqrt{b}}-\sqrt{ab}\right)\cdot\dfrac{\sqrt{a}+\sqrt{b}}{\sqrt{a}-\sqrt{b}}\)
\(=\left(a-\sqrt{ab}+b-\sqrt{ab}\right)\cdot\dfrac{\sqrt{a}+\sqrt{b}}{\sqrt{a}-\sqrt{b}}\)
\(=\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{a}-\sqrt{b}\right)\)
=a-b
b) Ta có: \(B=\left(\dfrac{a\sqrt{a}-b\sqrt{b}}{\sqrt{a}-\sqrt{b}}+\sqrt{ab}\right):\left(\sqrt{a}+\sqrt{b}\right)^2\)
\(=\left(a+\sqrt{ab}+\sqrt{ab}+b\right):\left(\sqrt{a}+\sqrt{b}\right)^2\)
\(=1\)
c) Ta có: \(C=\dfrac{x-y}{\sqrt{x}-\sqrt{y}}-\dfrac{\sqrt{x^3}-\sqrt{y^3}}{x+\sqrt{xy}+y}-2\sqrt{y}\)
\(=\sqrt{x}+\sqrt{y}-\sqrt{x}+\sqrt{y}-2\sqrt{y}\)
=0

\(a,=\sqrt{x^3}-1=x\sqrt{x}-1\\ b,=\sqrt{x^3}-\sqrt{y^3}=x\sqrt{x}-y\sqrt{y}\\ c,=8\sqrt{x^3}+\sqrt{y^3}=2x\sqrt{x}+y\sqrt{y}\)
a) \(\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)=\left(\sqrt{x}\right)^3-1=x\sqrt{x}-1\)
b) \(\left(\sqrt{x}+\sqrt{y}\right)\left(x-\sqrt{x}.\sqrt{y}+y\right)=\left(\sqrt{x}\right)^3+\left(\sqrt{y}\right)^3=x\sqrt{x}+y\sqrt{y}\)
c) \(\left(2\sqrt{x}+\sqrt{y}\right)\left(3\sqrt{x}-2\sqrt{y}\right)=6x-4\sqrt{xy}+3\sqrt{xy}-2y=6x-\sqrt{xy}-2y\)


Ủa r siêng năng may vượt mức chi r để hs phải đi tính zị trời😤


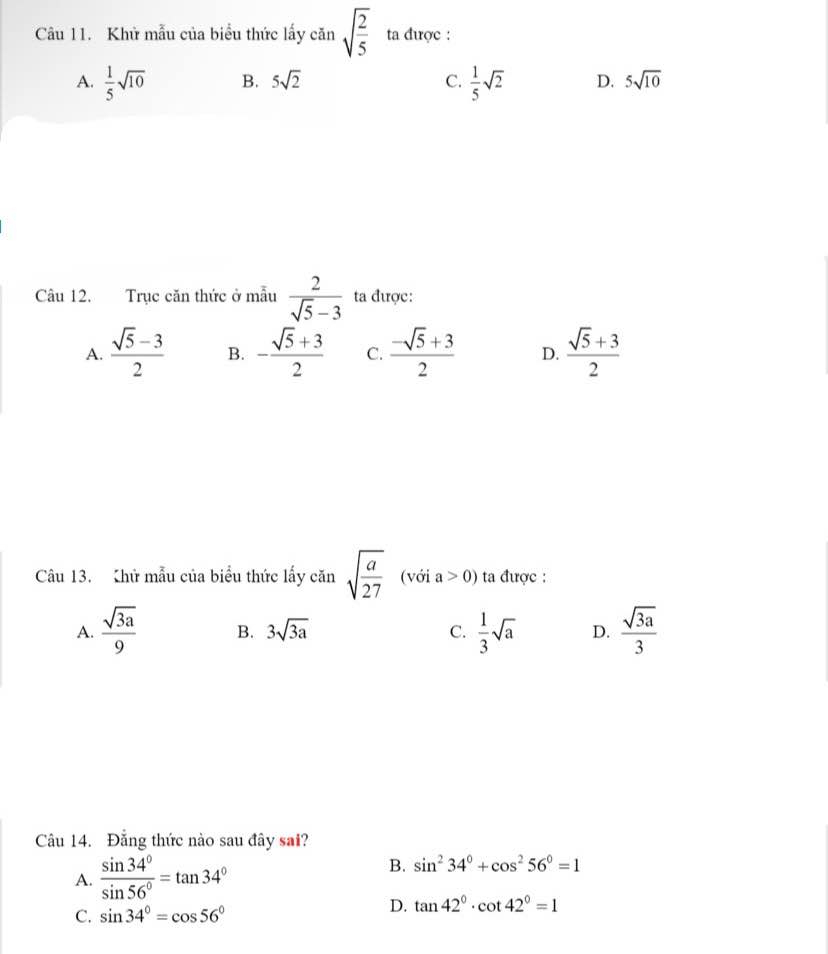
\(11,=\dfrac{\sqrt{10}}{5}=\dfrac{1}{5}\sqrt{10}\left(A\right)\\ 12,=\dfrac{2\left(\sqrt{5}+3\right)}{-4}=-\dfrac{\sqrt{5}+3}{2}\left(B\right)\\ 13,=\dfrac{\sqrt{a}}{3\sqrt{3}}=\dfrac{\sqrt{3a}}{9}\left(A\right)\\ 14,B\)

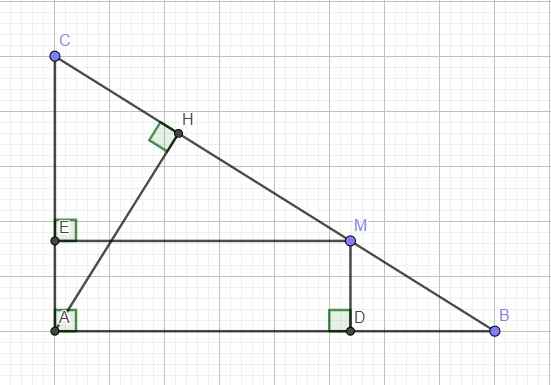
Theo giả thiết: \(ME\perp AC\Rightarrow\Delta MEA\) vuông tại E \(\Rightarrow\) E thuộc đường tròn đường kính AM (1)
Tương tự, tam giác MDA vuông tại D \(\Rightarrow\) D thuộc đường tròn đường kính AM (2)
Tam giác MHA vuông tại H \(\Rightarrow\) H thuộc đường tròn đường kính AM (3)
(1);(2);(3) \(\Rightarrow\) 5 điểm A, D, M, H, E cùng thuộc đường tròn đường kính AM (đpcm)

\(a,\) Tự vẽ nhaa
\(b,\) Gọi \(A\left(x_A;y_A\right);B\left(x_B;y_B\right)\) là tọa độ giao điểm của \(\left(P\right)\) và \(\left(d\right)\)
Ta có : \(\left(P\right)=\left(d\right)\)
Suy ra :
\(2x^2=-3x+1\)
\(\Leftrightarrow2x^2+3x-1=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_1=\dfrac{-3+\sqrt{17}}{4}\\x_2=\dfrac{-3-\sqrt{17}}{4}\end{matrix}\right.\)
Thay \(x_1=\dfrac{-3+\sqrt{17}}{4}\) vào \(\left(P\right):y=2x^2\Rightarrow y=2.\left(\dfrac{-3+\sqrt{17}}{4}\right)=\dfrac{-3+\sqrt{17}}{2}\)
Thay \(x_2=\dfrac{-3-\sqrt{17}}{4}\) vào \(\left(d\right):y=-3x+1\Rightarrow y=-3.\left(\dfrac{-3-\sqrt{17}}{4}\right)+1=\dfrac{13+3\sqrt{17}}{4}\)
Vậy toa độ giao điểm của 2 đồ thị hàm số là
\(A\left(\dfrac{-3+\sqrt{17}}{4};\dfrac{-3+\sqrt{17}}{2}\right)\) và \(B\left(\dfrac{-3-\sqrt{17}}{4};\dfrac{13+3\sqrt{17}}{4}\right)\)

a: 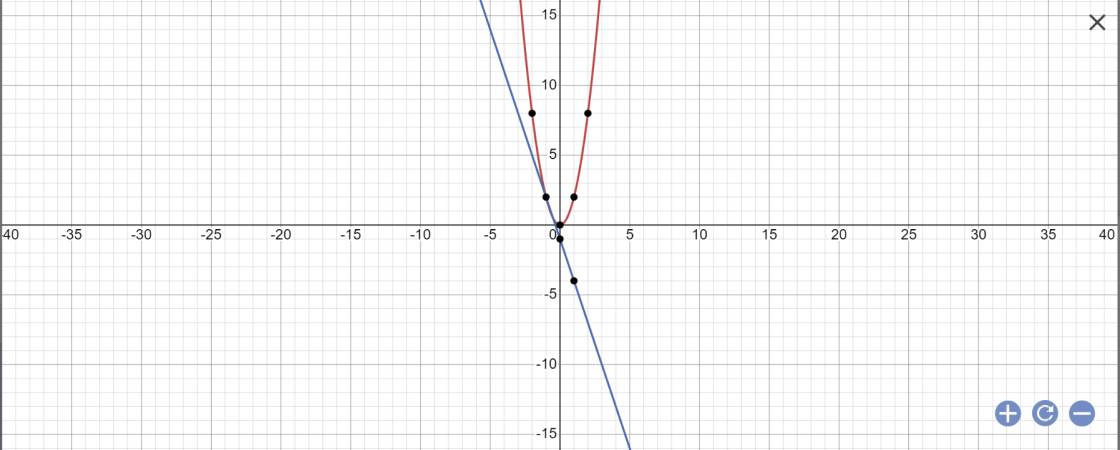
b: PTHĐGĐ là:
2x^2+3x+1=0
=>x=-1 hoặc x=-1/2
=>y=2 hoặc y=1/2
\(a,\) Tự vẽ nha
\(b,\) Gọi \(A\left(x_A;y_A\right);B\left(x_B;y_B\right)\) là tọa độ của \(\left(P\right)\) và \(\left(d\right)\)
Ta có : \(\left(P\right)=\left(d\right)\)
Suy ra :
\(2x^2=-3x-1\)
\(\Leftrightarrow2x^2+3x+1=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_1=-\dfrac{1}{2}\\x_2=-1\end{matrix}\right.\)
Thay \(x_1=-\dfrac{1}{2}\) vào \(\left(P\right):y=2x^2\Rightarrow y=2.\left(-\dfrac{1}{2}\right)^2=\dfrac{1}{2}\)
Thay \(x_2=-1\) vào \(\left(d\right):y=-3x-1\Rightarrow y=-3.\left(-1\right)-1=2\)
Vậy tọa độ của 2 đồ thị hàm số là \(A\left(-\dfrac{1}{2};\dfrac{1}{2}\right);B\left(-1;2\right)\)
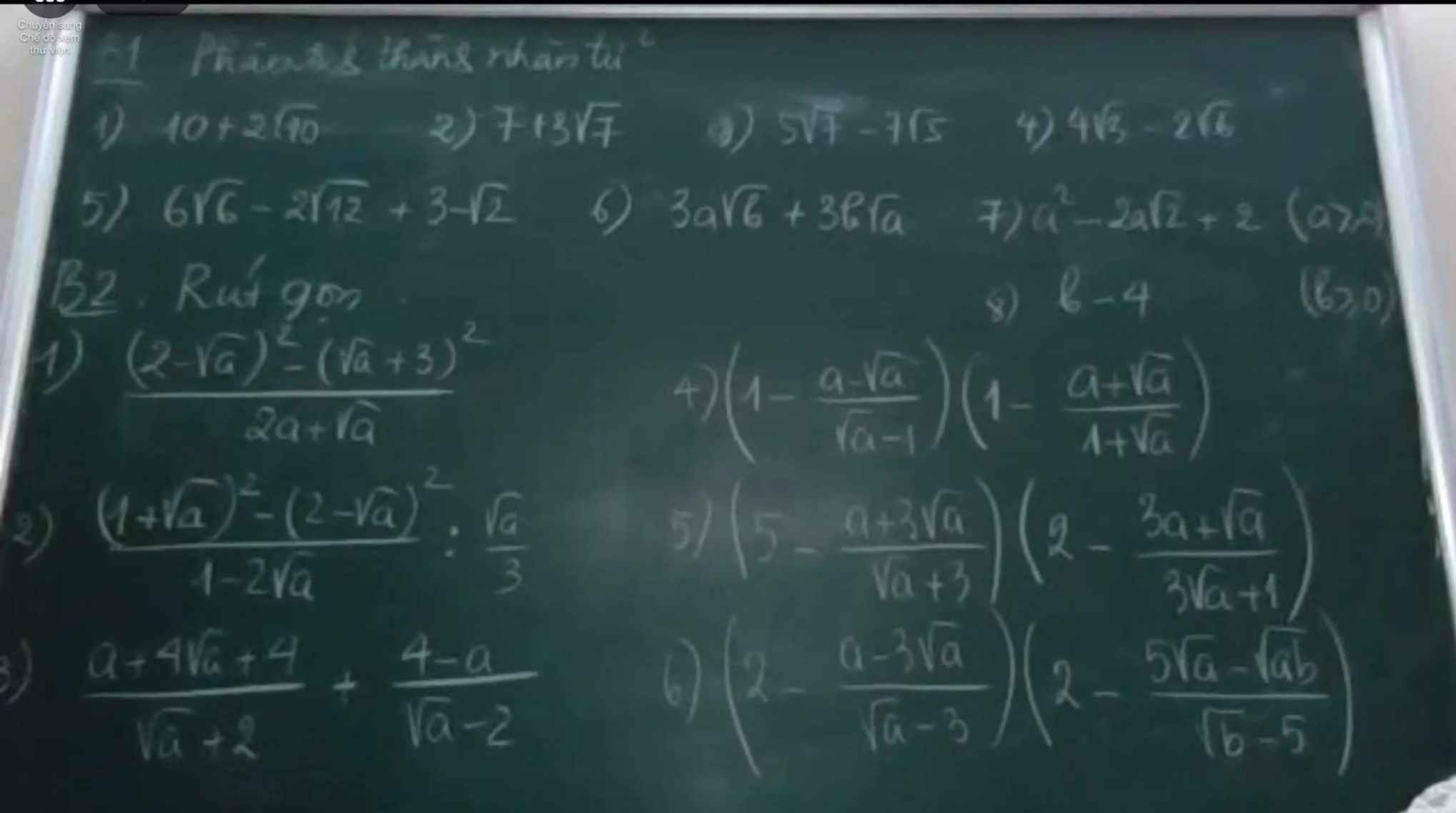

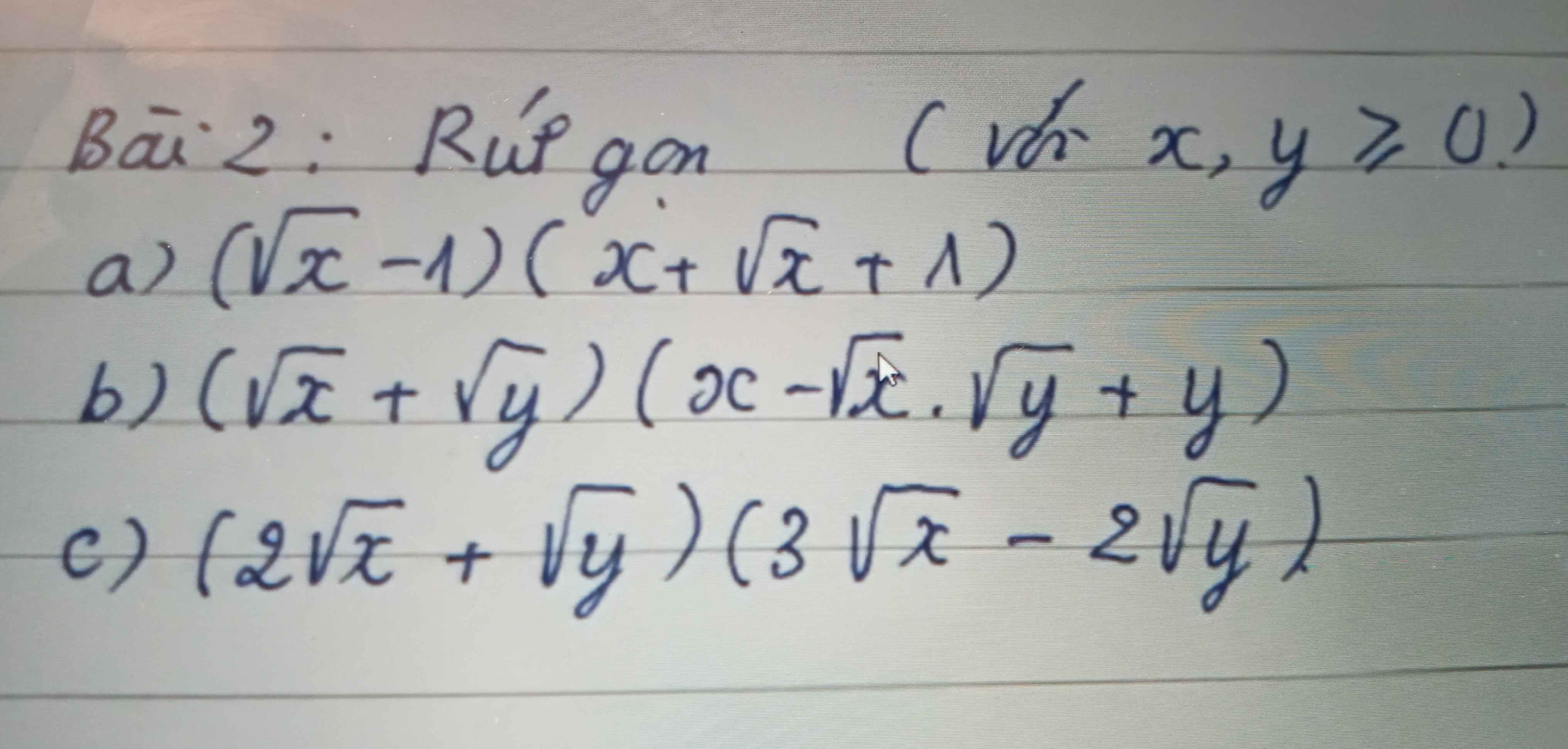



 mọi người giúp em với ạ 🥺
mọi người giúp em với ạ 🥺
Bài 1:1) \(10+2\sqrt{10}=\sqrt{10}\left(2+\sqrt{10}\right)\)
2) \(7+3\sqrt{7}=\sqrt{7}\left(3+\sqrt{7}\right)\)
các câu 3,4,5 bạn làm tương tự như 2 câu trên
6) \(3a\sqrt{b}+3b\sqrt{a}=3\sqrt{ab}\left(\sqrt{a}+\sqrt{b}\right)\)
7) \(a^2-2a\sqrt{2}+2=a^2-2a\sqrt{2}+\left(\sqrt{2}\right)^2=\left(a-\sqrt{2}\right)^2\)
8) \(b-4=\left(\sqrt{b}\right)^2-2^2=\left(\sqrt{b}-2\right)\left(\sqrt{b}+2\right)\)
Bài 2: 1) \(\dfrac{\left(2-\sqrt{a}\right)^2-\left(\sqrt{a}+3\right)^2}{2a+\sqrt{a}}\left(a>0\right)\)
\(=\dfrac{\left(2-\sqrt{a}-\sqrt{a}-3\right)\left(2-\sqrt{a}+\sqrt{a}+3\right)}{\sqrt{a}\left(2\sqrt{a}+1\right)}\)
\(=\dfrac{\left(-2\sqrt{a}-1\right).5}{\sqrt{a}\left(2\sqrt{a}+1\right)}=-\dfrac{5}{\sqrt{a}}\)
3) \(\dfrac{a+4\sqrt{a}+4}{\sqrt{a}+2}+\dfrac{4-a}{\sqrt{a}-2}\left(a\ge0,a\ne4\right)\)
\(=\dfrac{\left(\sqrt{a}+2\right)^2}{\sqrt{a}+2}+\dfrac{\left(2-\sqrt{a}\right)\left(2+\sqrt{a}\right)}{\sqrt{a}-2}=\sqrt{a}+2-\left(2+\sqrt{a}\right)=0\)
4) \(\left(1-\dfrac{a-\sqrt{a}}{\sqrt{a}-1}\right)\left(1-\dfrac{a+\sqrt{a}}{1+\sqrt{a}}\right)\left(a\ge0,a\ne1\right)\)
\(=\left(1-\dfrac{\sqrt{a}\left(\sqrt{a}-1\right)}{\sqrt{a}-1}\right)\left(1-\dfrac{\sqrt{a}\left(\sqrt{a}+1\right)}{1+\sqrt{a}}\right)\)
\(=\left(1-\sqrt{a}\right)\left(1-\sqrt{a}\right)=\left(1-\sqrt{a}\right)^2=a-2\sqrt{a}+1\)
mấy câu còn lại bạn làm tương tự
Bài 1:
1) \(10+2\sqrt{10}=2\sqrt{5}\left(\sqrt{5}+\sqrt{2}\right)\)
2) \(7+3\sqrt{7}=\sqrt{7}\left(\sqrt{7}+3\right)\)
3) \(5\sqrt{7}-7\sqrt{5}=\sqrt{35}\left(\sqrt{5}-\sqrt{7}\right)\)
4) \(4\sqrt{3}-2\sqrt{6}=2\sqrt{6}\left(\sqrt{2}-\sqrt{1}\right)\)
5) \(6\sqrt{6}-2\sqrt{12}+3\sqrt{2}\)
\(=\sqrt{216}-\sqrt{48}+\sqrt{18}\)
\(=\sqrt{6}\left(6-2\sqrt{2}+\sqrt{3}\right)\)
6) \(3a\sqrt{6}+36\sqrt{a}\)
\(=3\sqrt{a}\left(\sqrt{6a}+12\right)\)
\(=3\sqrt{6a}\left(\sqrt{a}+2\sqrt{6}\right)\)
7) \(a^2-2a\sqrt{2}+2=\left(a-\sqrt{2}\right)^2\)
8) \(b-4=\left(\sqrt{b}-2\right)\left(\sqrt{b}+2\right)\)
Bài 2:
a) Ta có: \(\dfrac{\left(2-\sqrt{a}\right)^2-\left(\sqrt{a}+3\right)^2}{2a+\sqrt{a}}\)
\(=\dfrac{a-4\sqrt{a}+4-a-6\sqrt{a}-9}{2a+\sqrt{a}}\)
\(=\dfrac{-10\sqrt{a}-5}{\sqrt{a}\left(2\sqrt{a}+1\right)}\)
\(=\dfrac{-5\left(2\sqrt{a}+1\right)}{\sqrt{a}\left(2\sqrt{a}+1\right)}\)
\(=-\dfrac{5}{\sqrt{a}}\)
4) Ta có: \(\left(1-\dfrac{a-\sqrt{a}}{\sqrt{a}-1}\right)\left(1-\dfrac{a+\sqrt{a}}{1+\sqrt{a}}\right)\)
\(=\left(1-\sqrt{a}\right)\left(1-\sqrt{a}\right)\)
\(=a-2\sqrt{a}+1\)