
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a) ĐKXĐ: \(\left\{{}\begin{matrix}x\le-1\\x\ge2\end{matrix}\right.\)
\(\sqrt{x^2-x-2}-\sqrt{x-2}=0\\ \Leftrightarrow\sqrt{x^2-x-2}=\sqrt{x-2}\\ \Leftrightarrow x^2-x-2=x-2\\ \Leftrightarrow x^2-2x=0\\ \Leftrightarrow x\left(x-2\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\left(ktm\right)\\x=2\left(tm\right)\end{matrix}\right.\)
\(a,ĐK:x\ge2\\ PT\Leftrightarrow x^2-x-2=x-2\\ \Leftrightarrow x^2-2x=0\\ \Leftrightarrow\left[{}\begin{matrix}x=2\left(tm\right)\\x=0\left(ktm\right)\end{matrix}\right.\Leftrightarrow x=2\\ b,ĐK:\left[{}\begin{matrix}x\le-1\\x\ge1\end{matrix}\right.\\ PT\Leftrightarrow\sqrt{x^2-1}=x^2-1\\ \Leftrightarrow x^2-1=\left(x^2-1\right)^2\\ \Leftrightarrow\left(x^2-1\right)\left(x^2-1-1\right)=0\\ \Leftrightarrow\left(x-1\right)\left(x+1\right)\left(x-\sqrt{2}\right)\left(x+\sqrt{2}\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=1\left(tm\right)\\x=-1\left(tm\right)\\x=\sqrt{2}\left(tm\right)\\x=-\sqrt{2}\left(tm\right)\end{matrix}\right.\)
\(c,ĐK:\left[{}\begin{matrix}x\le-2\\x\ge1\end{matrix}\right.\\ PT\Leftrightarrow\sqrt{x^2-x}=-\sqrt{x^2+x-2}\\ \Leftrightarrow x^2-x=x^2+x-2\\ \Leftrightarrow2x=2\\ \Leftrightarrow x=1\left(tm\right)\)

Bài 5:
a: Xét ΔBEC và ΔADC có
\(\widehat{C}\) chung
\(\widehat{EBC}=\widehat{DAC}\)
Do đó: ΔBEC\(\sim\)ΔADC

\(3,\\ A=\dfrac{1}{x^2-4x+9}=\dfrac{1}{\left(x-2\right)^2+5}\)
Vì \(\left(x-2\right)^2+5\ge5\Leftrightarrow A\le\dfrac{1}{5}\)
\(A_{max}=\dfrac{1}{5}\Leftrightarrow x=2\)
\(B=\dfrac{1}{x^2-6x+17}=\dfrac{1}{\left(x-3\right)^2+8}\)
Vì \(\left(x-3\right)^2+8\ge8\Leftrightarrow B\le\dfrac{1}{8}\)
\(B_{max}=\dfrac{1}{8}\Leftrightarrow x=3\)

Bài 7:
a: \(A=x+\sqrt{x}\ge0\forall x\)
Dấu '=' xảy ra khi x=0


\(4,\\ b,B=\dfrac{x}{y}+\dfrac{y}{z}+\dfrac{z}{x}\ge3\sqrt[3]{\dfrac{xyz}{xyz}}=3\)
Dấu \("="\Leftrightarrow x=y=z\)
\(c,x+y=4\Leftrightarrow x=4-y\\ \Leftrightarrow C=\left(4-y\right)^2+y^2\\ C=16-8y+y^2+y^2=2\left(y^2-4y+4\right)+8\\ C=2\left(y-2\right)^2+8\ge8\\ C_{min}=8\Leftrightarrow x=y=2\)


a) x\(^3\)+3x\(^2\)-2x-6=0
⇔(x+3)(x+\(\sqrt{2}\))(x-\(\sqrt{2}\))
⇔x+3=0 hoặc x+\(\sqrt{2}\)=0 hoặc x-\(\sqrt{2}\)=0
⇔x=-3 hoặc x=-\(\sqrt{2}\) hoặc x=\(\sqrt{2}\)
b)2x\(^3\)+7x\(^2\)+7x+2=0
⇔(x+1)(2x+1)(x+2)=0
⇔x+1=0 hoặc2x+1=0 hoặc x+2=0
⇔x=-1 hoặcx=-\(\dfrac{1}{2}\) hoặc x=-2
c)x\(^3\)+7x\(^2\)-56x+48=0
⇔(x-1)(x-4)(x+12)=0
⇔x-1=0 hoặcx-4=0 hoặcx+12=0
⇔x =1hoặcx=4 hoặcx=-12

\(d,ĐK:x\ge1\\ PT\Leftrightarrow\sqrt{x-1}=2+\sqrt{x+1}\\ \Leftrightarrow x-1=2+x+1+4\sqrt{x+1}\\ \Leftrightarrow4\sqrt{x+1}=-4\Leftrightarrow x\in\varnothing\left(4\sqrt{x+1}\ge0\right)\\ g,ĐK:x\ge\dfrac{1}{2}\\ PT\Leftrightarrow x+\sqrt{2x-1}+x-\sqrt{2x-1}+2\sqrt{\left(x+\sqrt{2x-1}\right)\left(x-\sqrt{2x-1}\right)}=2\\ \Leftrightarrow2x+2\sqrt{x^2-2x+1}=2\\ \Leftrightarrow\sqrt{\left(x-1\right)^2}=\dfrac{2-2x}{2}=1-x\\ \Leftrightarrow\left|x-1\right|=1-x\\ \Leftrightarrow\left[{}\begin{matrix}x-1=1-x\left(x\ge1\right)\\x-1=x-1\left(x< 1\right)\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\left(tm\right)\\x\in R\end{matrix}\right.\)

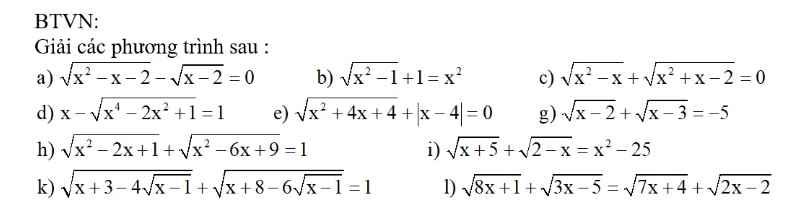








a, Xét tg ADH và tg BCK có
\(AD=BC;\widehat{ADH}=\widehat{BCK}\) (hình thang cân ABCD)\(;\widehat{AHD}=\widehat{BKC}\left(=90^0\right)\)
Nên \(\Delta ADH=\Delta BCK\left(ch-gn\right)\)
\(\Rightarrow DH=CK\)