Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

x2 +y2 +z2 -2xy-2zx-2yz=(x-y-z)2 -4yz=(x-y-z)2 - \(2.\sqrt{yz^2}\)=\(\left(x-y-z-2\sqrt{yz}\right)+\left(x-y-z+2\sqrt{yz}\right)\)
x2 -2xy - y2 -z2 =(x-y)2 -z2 =(x-y-z)(x-y+z)

a) Ta có: \(VT=\left(x-y-z\right)^2\)
\(=\left(x-y-z\right)\left(x-y-z\right)\)
\(=x^2-xy-xz-yx+y^2+yz-zx+zy+z^2\)
\(=x^2+y^2+z^2-2xy+2yz-2xz\)
=VP(đpcm)
b) Ta có: \(VT=\left(x+y-z\right)^2\)
\(=\left(x+y-z\right)\left(x+y-z\right)\)
\(=x^2+xy-xz+yx+y^2-yz-zx-zy+z^2\)
\(=x^2+y^2+z^2+2xy-2yz-2zx\)
=VP(đpcm)
c) Sửa đề: Chứng minh \(\left(x-y\right)\left(x^3+x^2y+xy^2+y^3\right)=x^4-y^4\)
Ta có: \(VT=\left(x-y\right)\left(x^3+x^2y+xy^2+y^3\right)\)
\(=x^4+x^3y+x^2y^2+xy^3-x^3y-x^2y^2-xy^3-y^4\)
\(=x^4-y^4\)
=VP(đpcm)
d) Ta có: \(VT=\left(x+y\right)\left(x^4-x^3y+x^2y^2-xy^3+y^4\right)\)
\(=x^5-x^4y+x^3y^2-x^2y^3+xy^4+x^4y-x^3y^2+x^2y^3-xy^4+y^5\)
\(=x^5+y^5\)
=VP(đpcm)
a, b, nhân vào là ra à
c, nghe cứ là lạ
d, cũng nhân là ra hà
\(=x^5-x^4y+x^3y^2-x^2y^3+xy^4+x^4y-x^3y^2+x^2y^3-xy^4+y^5=x^5+y^5\)

x 2 - 2 x y + y 2 - z 2 = x - y 2 - z 2 = (x – y + z)(x – y − z)
y 2 - 2 y z + z 2 - x 2 = y - z 2 - x 2 = (y – z + x)(y – z − x) = -(x +y – z)(x – y + z)
z 2 - 2 z x + x 2 - y 2 = z - x 2 - y 2 = (z – x + y)(z – x -y) = (x- y –z).(x + y – z)
MTC = (x – y + z)(x + y − z)(x – y − z)
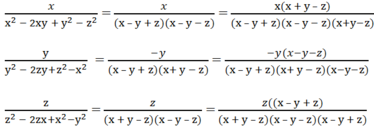

=[(x+y)+z]2
=(x+y)2+2(x+y)z+z2
=x2+2xy+y2+2xz+2yz+z2
=x2+y2+z2+2xy+2yz+2xz

a) Ta có: \(VP=x^2+y^2+z^2-2xy+2yz-2zx\)
\(=\left(x^2-xy-xz\right)+\left(y^2-xy+yz\right)+\left(z^2-yz-zx\right)\)
\(=x\left(x-y-z\right)+y\left(y-x+z\right)+z\left(z-y-x\right)\)
\(=x\left(x-y-z\right)-y\left(x-y-z\right)-z\left(x-y-z\right)\)
\(=\left(x-y-z\right)\left(x-y-z\right)\)
\(=\left(x-y-z\right)^2=VT\)(đpcm)
b) Ta có: \(VP=x^2+y^2+z^2+2xy-2yz-2zx\)
\(=\left(x^2+xy-zx\right)+\left(y^2+xy-2yz\right)+\left(z^2-yz-zx\right)\)
\(=x\left(x+y-z\right)+y\left(x+y-z\right)+z\left(z-y-x\right)\)
\(=\left(x+y-z\right)\left(x+y\right)-z\left(x+y-z\right)\)
\(=\left(x+y-z\right)\left(x+y-z\right)\)
\(=\left(x+y-z\right)^2=VT\)(đpcm)
c) Ta có: \(VP=x^4-y^4\)
\(=\left(x^2-y^2\right)\left(x^2+y^2\right)\)
\(=\left(x-y\right)\left(x+y\right)\left(x^2+y^2\right)\)
\(=\left(x-y\right)\left(x^3+xy^2+x^2y+y^3\right)=VT\)(đpcm)
d) Ta có: \(VT=\left(x+y\right)\left(x^4-x^3y+x^2y^2-xy^3+y^4\right)\)
\(=x^5-x^4y+x^3y^2-x^2y^3+xy^4+x^4y-x^3y^2+x^2y^3-xy^4+y^5\)
\(=x^5+y^5=VP\)(đpcm)

\(B=\dfrac{\left(x+y+z\right)^2}{x^2-\left(y^2+2yz+z^2\right)}=\dfrac{\left(x+y+z\right)^2}{x^2-\left(y+z\right)^2}\)
\(=\dfrac{\left(x+y+z\right)^2}{\left(x+y+z\right)\left(x-y-z\right)}=\dfrac{x+y+z}{x-y-z}\)

.