Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a. Đề bài em ghi sai thì phải
Vì:
\(x+y=2\left(\sqrt{x-3}+\sqrt{y-3}\right)\)
\(\Leftrightarrow\left(x-3-2\sqrt{x-3}+1\right)+\left(y-3-2\sqrt{y-3}+1\right)+4=0\)
\(\Leftrightarrow\left(\sqrt{x-3}-1\right)^2+\left(\sqrt{y-3}-1\right)^2+4=0\) (vô lý)
b.
Xét hàm \(f\left(x\right)=x^3+ax^2+bx+c\)
Hàm đã cho là hàm đa thức nên liên tục trên mọi khoảng trên R
Hàm bậc 3 nên có tối đa 3 nghiệm
\(f\left(-2\right)=-8+4a-2b+c>0\)
\(f\left(2\right)=8+4a+2b+c< 0\)
\(\Rightarrow f\left(-2\right).f\left(2\right)< 0\Rightarrow f\left(x\right)\) luôn có ít nhất 1 nghiệm thuộc (-2;2)
\(\lim\limits_{x\rightarrow+\infty}f\left(x\right)=x^3\left(1+\dfrac{a}{x}+\dfrac{b}{x^2}+\dfrac{c}{x^3}\right)=+\infty.\left(1+0+0+0\right)=+\infty\)
\(\Rightarrow\) Luôn tồn tại 1 số thực dương n đủ lớn sao cho \(f\left(n\right)>0\)
\(\Rightarrow f\left(2\right).f\left(n\right)< 0\Rightarrow f\left(x\right)\) luôn có ít nhất 1 nghiệm thuộc \(\left(2;n\right)\) hay \(\left(2;+\infty\right)\)
Tương tự \(\lim\limits_{x\rightarrow-\infty}f\left(x\right)=-\infty\Rightarrow f\left(-2\right).f\left(m\right)< 0\Rightarrow f\left(x\right)\) luôn có ít nhất 1 nghiệm thuộc \(\left(-\infty;-2\right)\)
\(\Rightarrow f\left(x\right)\) có đúng 3 nghiệm pb \(\Rightarrow\) hàm cắt Ox tại 3 điểm pb

Đặt \(\left(\dfrac{x}{6};\dfrac{y}{3};\dfrac{z}{2}\right)=\left(a;b;c\right)\Rightarrow2^{6a}+4^{3b}+8^{2c}=4\)
\(\Leftrightarrow64^a+64^b+64^c=4\)
Áp dụng BĐT Cô-si:
\(4=64^a+64^b+64^c\ge3\sqrt[3]{64^{a+b+c}}\Rightarrow64^{a+b+c}\le\dfrac{64}{27}\)
\(\Rightarrow a+b+c\le log_{64}\left(\dfrac{64}{27}\right)\Rightarrow M=log_{64}\left(\dfrac{64}{27}\right)\)
Lại có: \(x;y;z\ge0\Rightarrow a;b;c\ge0\)
\(\Rightarrow\left\{{}\begin{matrix}64^a\ge1\\64^b\ge1\\64^c\ge1\end{matrix}\right.\) \(\Rightarrow\left(64^b-1\right)\left(64^c-1\right)\ge0\)
\(\Rightarrow64^{b+c}+1\ge64^b+64^c\) (1)
Lại có: \(b+c\ge0\Rightarrow64^{b+c}\ge1\Rightarrow\left(64^a-1\right)\left(64^{b+c}-1\right)\ge0\)
\(\Rightarrow64^{a+b+c}+1\ge64^a+64^{b+c}\) (2)
Cộng vế (1);(2) \(\Rightarrow4=64^a+64^b+64^c\le64^{a+b+c}+2\)
\(\Rightarrow64^{a+b+c}\ge2\Rightarrow a+b+c\ge log_{64}2\)
\(\Rightarrow N=log_{64}2\)
\(\Rightarrow T=2log_{64}\left(\dfrac{64}{27}\right)+6log_{64}\left(2\right)\approx1,4\)

\(4=2^x+2^y\ge2\sqrt{2^{x+y}}\Rightarrow2^{x+y}\le4\Rightarrow x+y\le2\)
\(\Rightarrow xy\le1\)
\(P=4x^2y^2+2x^3+2y^3+10xy\)
\(P=4x^2y^2+10xy+2\left(x+y\right)\left[\left(x+y\right)^2-3xy\right]\)
\(P\le4x^2y^2+10xy+4\left(4-3xy\right)=4x^2y^2-2xy+16\)
Đặt \(xy=t\Rightarrow0< t\le1\)
Xét hàm \(f\left(t\right)=4t^2-2t+16\) trên \((0;1]\)
\(\Rightarrow...\)

\(\Leftrightarrow2y^3-6y^2+7y-3=-2x\sqrt{1-x}+2\sqrt{1-x}+\sqrt{1-x}\)
\(\Leftrightarrow2\left(y^3-3y^2+3y+1\right)+y-1=2\left(1-x\right)\sqrt{1-x}+\sqrt{1-x}\)
\(\Leftrightarrow2\left(y-1\right)^3+y-1=2\left(\sqrt{1-x}\right)^3+\sqrt{1-x}\) (1)
Xét hàm \(f\left(t\right)=2t^3+t\)
\(f'\left(t\right)=6t^2+1>0\Rightarrow f\left(t\right)\) đồng biến
Nên (1) tương đương: \(y-1=\sqrt{1-x}\Rightarrow y=1+\sqrt{1-x}\)
\(\Rightarrow P=x+2\sqrt{1-x}+2=-\left(1-x-2\sqrt{1-x}+1\right)+4=-\left(\sqrt{1-x}-1\right)^2+4\le4\)
⇒ P = x + 2 √ 1 − x + 2
= − ( 1 − x − 2 √ 1 − x + 1 ) + 4
= − ( √ 1 − x − 1 ) 2 + 4 ≤ 4
Cho xin một like đi các dân chơi à.


\(\left(x+y\right)xy=x^2+y^2-xy\)
\(\Leftrightarrow\left(x+y\right)xy=\left(x+y\right)^2-3xy\)
Đặt \(x+y=t\Rightarrow xy=\frac{t^2}{t+3}\)
Lại có \(\left(x+y\right)^2\ge4xy\Rightarrow t^2\ge\frac{4t^2}{t+3}\)
\(\Leftrightarrow t^2\left(\frac{t-1}{t+3}\right)\ge0\Rightarrow\left[{}\begin{matrix}t\ge1\\t< -3\end{matrix}\right.\)
\(A=\frac{x^3+y^3}{\left(xy\right)^3}=\frac{\left(x+y\right)\left(x^2+y^2-xy\right)}{\left(xy\right)^3}=\frac{\left(x+y\right)\left(x+y\right)xy}{\left(xy\right)^3}=\left(\frac{x+y}{xy}\right)^2\)
\(A=\left(\frac{t\left(t+3\right)}{t^2}\right)^2=\left(\frac{t+3}{t}\right)^2=\left(1+\frac{3}{t}\right)^2\)
\(\Rightarrow y'=-\frac{6\left(t+3\right)}{t^3}< 0\) \(\forall t\ge1;t< -3\)
\(\lim\limits_{x\rightarrow-\infty}\left(1+\frac{3}{t}\right)^2=1\Rightarrow A_{max}=A\left(1\right)=16\)
\(\Rightarrow M=16\) khi \(x=y=\frac{1}{2}\)

Do \(1\le x\le2\Rightarrow\left(x-1\right)\left(x-2\right)\le0\)
\(\Leftrightarrow x^2+2\le3x\)
Hoàn toàn tương tự ta có \(y^2+2\le3y\)
Do đó: \(P\ge\dfrac{x+2y}{3x+3y+3}+\dfrac{2x+y}{3x+3y+3}+\dfrac{1}{4\left(x+y-1\right)}\)
\(P\ge\dfrac{x+y}{x+y+1}+\dfrac{1}{4\left(x+y-1\right)}\)
Đặt \(a=x+y-1\Rightarrow1\le a\le3\)
\(\Rightarrow P\ge f\left(a\right)=\dfrac{a+1}{a+2}+\dfrac{1}{4a}\)
\(f'\left(a\right)=\dfrac{3a^2-4a-4}{4a^2\left(a+2\right)^2}=\dfrac{\left(a-2\right)\left(3a+2\right)}{4a^2\left(a+2\right)^2}=0\Rightarrow a=2\)
\(f\left(1\right)=\dfrac{11}{12}\) ; \(f\left(2\right)=\dfrac{7}{8}\) ; \(f\left(3\right)=\dfrac{53}{60}\)
\(\Rightarrow f\left(a\right)\ge\dfrac{7}{8}\Rightarrow P_{min}=\dfrac{7}{8}\) khi \(\left(x;y\right)=\left(1;2\right);\left(2;1\right)\)
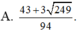
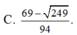
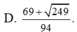
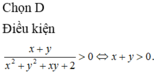

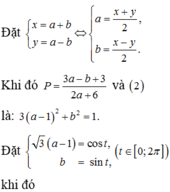





 thầy ơi giải như này được ko ạ?
thầy ơi giải như này được ko ạ? 
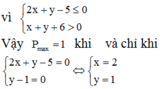
tag ko co thong bao de mai t nghien cuu
Bài này cái khó là sử lý điều kiện thôi nên t làm phần đó thôi nhé.
Từ điều kiện suy ra được.
log\(\sqrt{3}\)(3x + 3y) + (3x + 3y) = log\(\sqrt{3}\)(x2 + y2 + xy + 2) + (x2 + y2 + xy + 2)
Dễ thấy hàm số f(t) = log\(\sqrt{3}\)(t) + t đồng biến trên (0; +\(\infty\)) nên
=> 3x + 3y = x2 + y2 + xy + 2