Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(a,A=\dfrac{1}{x-2}+\dfrac{1}{x+2}+\dfrac{x^2+1}{x^2-4}\left(dkxd:x\ne\pm2\right)\)
\(=\dfrac{x+2+x-2+x^2+1}{\left(x-2\right)\left(x+2\right)}\)
\(=\dfrac{x^2+2x+1}{\left(x-2\right)\left(x+2\right)}\)
\(=\dfrac{\left(x+1\right)^2}{x^2-4}\)
Vậy \(A=\dfrac{\left(x+1\right)^2}{x^2-4}\)
\(b,\) Theo đề, ta có : \(-2< x< 2\)
\(\Rightarrow x-2< 0;x+2>0;\left(x+1\right)^2>0\)
\(\Rightarrow A< 0\) hay phân thức luôn có giá trị âm

a: ĐKXĐ: x<>1
Sửa đề: \(C=\dfrac{5x+1}{x^3-1}+\dfrac{2x-1}{x^2+x+1}+\dfrac{2}{x-1}\)
\(=\dfrac{5x+1+\left(2x-1\right)\left(x-1\right)+2x^2+2x+2}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{2x^2+7x+3+2x^2-2x-x+1}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{4x^2+4x+4}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{4}{x-1}\)
b: |x|=4
=>x=4 hoặc x=-4
Khi x=4 thì \(C=\dfrac{4}{4-1}=\dfrac{4}{3}\)
Khi x=-4 thì \(C=\dfrac{4}{-4-1}=\dfrac{4}{-5}=\dfrac{-4}{5}\)
c: C>0
=>4/x-1>0
=>x-1>0
=>x>1
d: C nguyên
=>x-1 thuộc {1;-1;2;-2;4;-4}
=>x thuộc {2;0;3;-1;5;-3}
Trong xu thế hội nhập hiện nay, hợp tác quốc tế là vấn đề quan trọng và tất yếu của mỗi quốc gia, dân tộc trên thế giới. Trong những năm gần đây, Việt Nam đã và đang trở thành một trong những điển hình về hợp tác quốc tế
a, từ nhận định trên em hãy cho biết hợp tác là gì? cơ sở và nguyên tắc của đang và nhà nước ta?
b, nêu 1 số thành quả hợp tác giữa các nước ta và các nước trên thế giới? từ đó em hãy cho biết hộc sinh hiên nay cần phải làm gì để rèn luyện tinh thần hợp tác

Điều kiện x ≠ 1 và x ≠ - 1
Ta có:
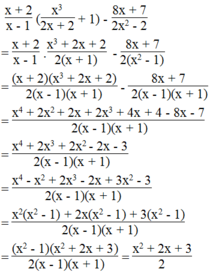
Biểu thức dương khi x 2 + 2 x + 3 > 0
Ta có: x 2 + 2 x + 3 = x 2 + 2 x + 1 + 2 = x + 1 2 + 2 > 0 với mọi giá trị của x.
Vậy giá trị của biểu thức dương với mọi giá trị x ≠ 1 và x ≠ - 1

a) \(A=\left(2x+1\right)^2-\left(x+2\right)\left(x-2\right)-2x\left(x+1\right)\)
\(A=4x^2+4x+1-x^2+4-2x^2-2x\)
\(A=x^2+2x+5\)
b) Để A = 4
=> \(x^2+2x+5=4\)
\(\Leftrightarrow x^2+2x+1=0\)
\(\Leftrightarrow\left(x+1\right)^2=0\)
\(\Leftrightarrow x+1=0\Leftrightarrow x=-1\)
c) Ta có A = x2 + 2x + 5
A = ( x + 1 )2 + 4
=> \(A\ge4>0\left(đpcm\right)\)
a,\(A=\left(2x+1\right)^2-\left(x+2\right)\left(x-2\right)-2x\left(x+1\right)\)
\(=4x^2+4x+1-x^2+4-2x^2-2x\)
\(=x^2+2x+5\)
b,\(A=x^2+2x+5=4\)
\(\Rightarrow x^2+2x+5-4=0\)
\(x^2+2x+1=0\)
\(\left(x+1\right)^2=0\)
\(x+1=0\)
\(x=-1\)
c, Ta có: \(A=x^2+2x+5=\left(x^2+2x+1\right)+4=\left(x+1\right)^2+4\ge4>0\)
Hay: A > 0 => đpcm
=.= hok tốt!!

a. \(A=\dfrac{1}{x-1}-\dfrac{1}{x+1}+\dfrac{4x+2}{x^2-1}\)
\(A=\dfrac{x+1}{\left(x-1\right)\left(x+1\right)}-\dfrac{x-1}{\left(x-1\right)\left(x+1\right)}+\dfrac{4x+2}{\left(x-1\right)\left(x+1\right)}\)
\(A=\dfrac{\left(x+1\right)-\left(x-1\right)+4x+2}{\left(x-1\right)\left(x+1\right)}\)
\(A=\dfrac{x+1-x+1+4x+2}{\left(x-1\right)\left(x+1\right)}\)
\(A=\dfrac{4x+4}{\left(x-1\right)\left(x+1\right)}=\dfrac{4\left(x+1\right)}{\left(x-1\right)\left(x+1\right)}=\dfrac{4}{x-1}\)
b) Ta có: \(A=\dfrac{4}{x-1}=\dfrac{4}{2015}\) (ĐK: \(x\ne\pm1\) )
\(\Leftrightarrow8060=4\left(x-1\right)\)
\(\Leftrightarrow8060=4x-4\)
\(\Leftrightarrow8064=4x\)
\(\Leftrightarrow x=\dfrac{8064}{4}=2016\left(tm\right)\)
c) Ta có: \(\dfrac{4}{x-1}\left(x\ne1\right)\)
Để \(\dfrac{4}{x-1}\) nhận giá trị nguyên thì \(4:\left(x-1\right)\Leftrightarrow x-1\in\text{Ư}\left(4\right)=\left\{1;4;2\right\}\)
Vậy với x ∈ {2; 5; 3; 0; -1; -3} thì biểu thức \(\dfrac{4}{x-1}\) nhận giá trị nguyên
d) Thay \(x=-\dfrac{1}{2}\) vào biểu thức A ta được:
\(\dfrac{4}{-\dfrac{1}{2}-1}=-3\)
Vậy biểu thức A có giá trị -3 tại \(x=-\dfrac{1}{2}\)

a: \(A=\dfrac{3\left(1-2x\right)}{2x\left(x^2+1\right)-\left(x^2+1\right)}\)
\(=\dfrac{-3\left(2x-1\right)}{\left(x^2+1\right)\left(2x-1\right)}=\dfrac{-3}{x^2+1}\)
b: Khi x=3 thì \(A=\dfrac{-3}{3^2+1}=-\dfrac{3}{10}\)
c: x^2+1>=0
=>3/x^2+1>=0
=>-3/x^2+1<=0
=>A<=0(ĐPCM)
ĐKXĐ: \(x\notin\left\{1;-1\right\}\)
a) Ta có: \(C=\left(x^2-1\right)\cdot\left(\dfrac{1}{x-1}-\dfrac{1}{x+1}+1\right)\)
\(=\left(x^2-1\right)\cdot\left(\dfrac{x+1}{\left(x-1\right)\left(x+1\right)}-\dfrac{x-1}{\left(x+1\right)\left(x-1\right)}+\dfrac{x^2-1}{\left(x-1\right)\left(x+1\right)}\right)\)
\(=\left(x^2-1\right)\cdot\dfrac{x+1-x+1+x^2-1}{\left(x+1\right)\left(x-1\right)}\)
\(=x^2-1\)