
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bài 6:
b: PTHĐGĐ là:
\(x^2+4x-1=x-3\)
\(\Leftrightarrow x^2+3x-4=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-4\\x=1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}y=-7\\y=-2\end{matrix}\right.\)

Câu 5:
\(\Leftrightarrow-x^2+7x-9+2x-9=0\)
\(\Leftrightarrow x^2-9x+18=0\)
=>x=3
=>Chọn A

a, Ta có : \(\sin^2x+\cos^2x=1\)
\(\Rightarrow\sin x=\sqrt{1-\cos^2x}=\left|\dfrac{\sqrt{15}}{4}\right|\)
Mà \(0< x< \dfrac{\pi}{2}\)
\(\Rightarrow\sin x=\dfrac{\sqrt{15}}{4}\)
Ta lại có : \(\left\{{}\begin{matrix}\sin2x=2\sin x\cos x=\dfrac{\sqrt{15}}{8}\\\cos2x=2\cos^2x-1=-\dfrac{7}{8}\end{matrix}\right.\)
Vậy ...
c, Ta có : \(\tan2x=\dfrac{2\tan x}{1-\tan^2x}=\dfrac{4}{3}=\dfrac{\sin2x}{\cos2x}\)
- Ta có HPT : \(\left\{{}\begin{matrix}\sin^22x+\cos^22x=1\\3\sin2x-4\cos2x=0\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}\sin2x=\left|\dfrac{4}{5}\right|\\\cos2x=\left|\dfrac{3}{5}\right|\end{matrix}\right.\)
Lại có : \(\pi< x< \dfrac{3}{2}\pi\)
\(\Rightarrow\left\{{}\begin{matrix}\sin2x=\dfrac{4}{5}\\\cos2x=\dfrac{3}{5}\end{matrix}\right.\)
Vậy ...

a. BPT đã cho vô nghiệm khi:
\(f\left(x\right)\ge0\) nghiệm đúng với mọi x
\(\Leftrightarrow\left\{{}\begin{matrix}a=1>0\\\Delta'=\left(m+2\right)^2-\left(3m^2+5m-8\right)\le0\end{matrix}\right.\)
\(\Leftrightarrow-2m^2-m+12\le0\) \(\Rightarrow\left[{}\begin{matrix}m\ge\dfrac{-1+\sqrt{97}}{4}\\m\le\dfrac{-1-\sqrt{97}}{4}\end{matrix}\right.\)
b.
\(f\left(x\right)=0\) có 2 nghiệm pb
\(\Leftrightarrow\Delta'=-2m^2-m+12>0\)
\(\Leftrightarrow\dfrac{-1-\sqrt{97}}{4}< m< \dfrac{-1+\sqrt{97}}{4}\)

a: Tọa độ đỉnh của (P) là:
\(\left\{{}\begin{matrix}x=\dfrac{-3}{2\cdot\left(-1\right)}=\dfrac{3}{2}\\y=-\dfrac{3^2-4\cdot\left(-1\right)\cdot\left(-2\right)}{4\cdot\left(-1\right)}=-\dfrac{9-4\cdot2}{-4}\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=\dfrac{3}{2}\\y=\dfrac{9-8}{4}=\dfrac{1}{4}\end{matrix}\right.\)
Vì (P): \(y=-x^2+3x-2\) có a=-1<0
nên hàm số đồng biến trên khoảng \(\left(-\infty;\dfrac{3}{2}\right)\) và nghịch biến trên khoảng \(\left(\dfrac{3}{2};+\infty\right)\)
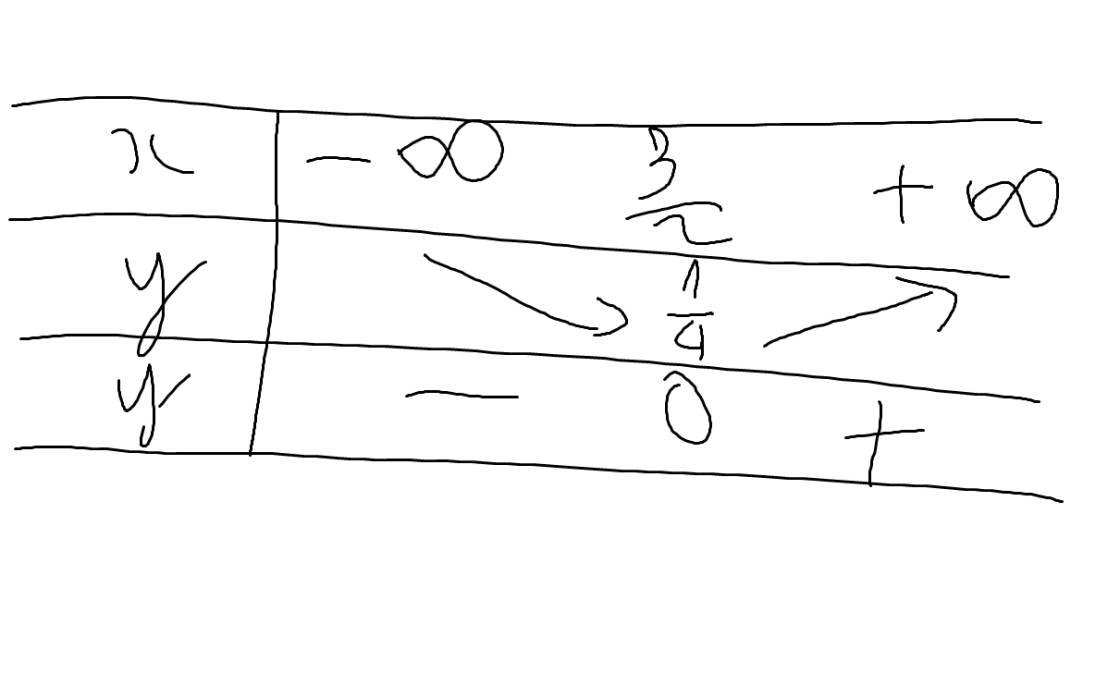
Bảng biến thiên là:

Đồ thị là:
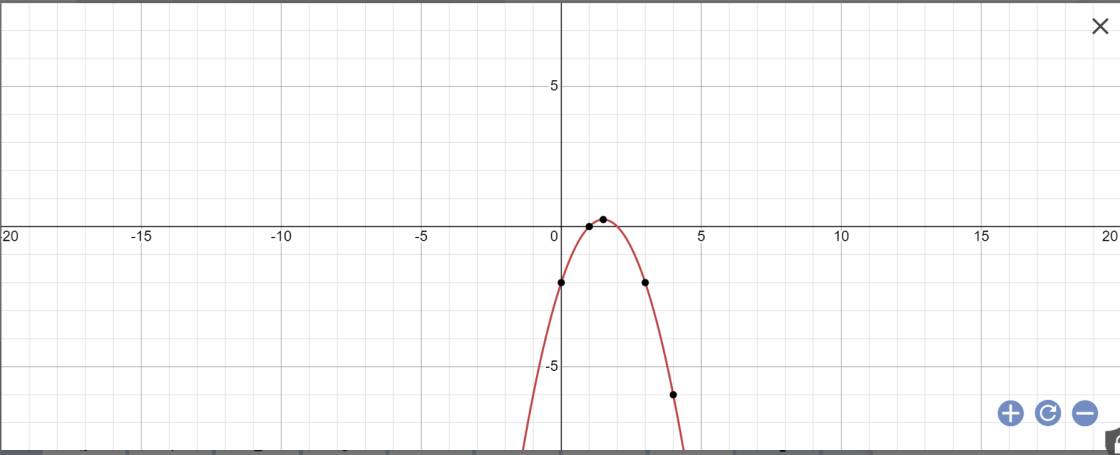
b: Dựa vào đồ thị, ta sẽ có:
Để y>0 thì 1<x<2
c: \(x^2-3x-m=0\)
=>\(x^2-3x=m\)
=>\(-x^2+3x=-m\)
=>\(-x^2+3x-2=2-m\)
Đặt \(y=g\left(x\right)=-x^2+3x-2\)
\(\Leftrightarrow y'=g'\left(x\right)=-2x+3\)
Đặt g'(x)=0
=>-2x+3=0
=>\(x=\dfrac{3}{2}\)
Để phương trình có 1 nghiệm thì \(2-m=\dfrac{1}{4}\)
=>\(m=2-\dfrac{1}{4}=\dfrac{7}{4}\)
Để phương trình có hai nghiệm thì \(2-m\ne\dfrac{1}{4}\)
=>\(m\ne2-\dfrac{1}{4}=\dfrac{7}{4}\)

ĐKXĐ: \(1\le x\le4\)
Đặt \(\sqrt{x-1}+\sqrt{4-x}=t\Rightarrow t^2=3+2\sqrt{\left(x-1\right)\left(4-x\right)}\)
\(\Rightarrow-\sqrt{\left(x-1\right)\left(4-x\right)}=-\dfrac{1}{2}t^2+\dfrac{3}{2}\)
Ta có:
\(\sqrt{x-1}+\sqrt{4-x}\le\sqrt{2\left(x-1+4-x\right)}=\sqrt{6}\)
\(\sqrt{x-1}+\sqrt{4-x}\ge\sqrt{x-1+4-x}=\sqrt{3}\)
\(\Rightarrow t\in\left[\sqrt{3};\sqrt{6}\right]\)
Phương trình trở thành:
\(-\dfrac{1}{2}t^2+t+\dfrac{3}{2}=m\)
Xét hàm \(f\left(t\right)=-\dfrac{1}{2}t^2+t+\dfrac{3}{2}\) với \(t\in\left[\sqrt{3};\sqrt{6}\right]\)
\(a=-\dfrac{1}{2}< 0;-\dfrac{b}{2a}=1< \sqrt{3}\)
\(\Rightarrow f\left(t\right)\) nghịch biến trên \(\left[\sqrt{3};\sqrt{6}\right]\)
\(\Rightarrow f\left(\sqrt{6}\right)\le f\left(t\right)\le f\left(\sqrt{3}\right)\Rightarrow\dfrac{-3+2\sqrt{6}}{2}\le f\left(t\right)\le\sqrt{3}\)
Vậy pt đã cho có nghiệm khi \(\dfrac{-3+2\sqrt{6}}{2}\le m\le\sqrt{3}\)

Lời giải:
$\overrightarrow{MA}=(1-x, 3-y), \overrightarrow{MB}=(4-x, 2-y)$
Để $MAB$ là tam giác vuông cân tại $M$ thì:
\(\left\{\begin{matrix}
\overrightarrow{MA}.\overrightarrow{MB}=0\\
MA^2=MB^2\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix}
(1-x)(4-x)+(3-y)(2-y)=0\\
(1-x)^2+(3-y)^2=(4-x)^2+(2-y)^2\end{matrix}\right.\)
\(\Leftrightarrow \left\{\begin{matrix} x^2-5x+y^2-5y+10=0\\ 6x-2y-10=0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x^2-5x+y^2-5y+10=0\\ y=3x-5\end{matrix}\right.\)
\(\Leftrightarrow (x,y)=(2,1), (3,4)\)






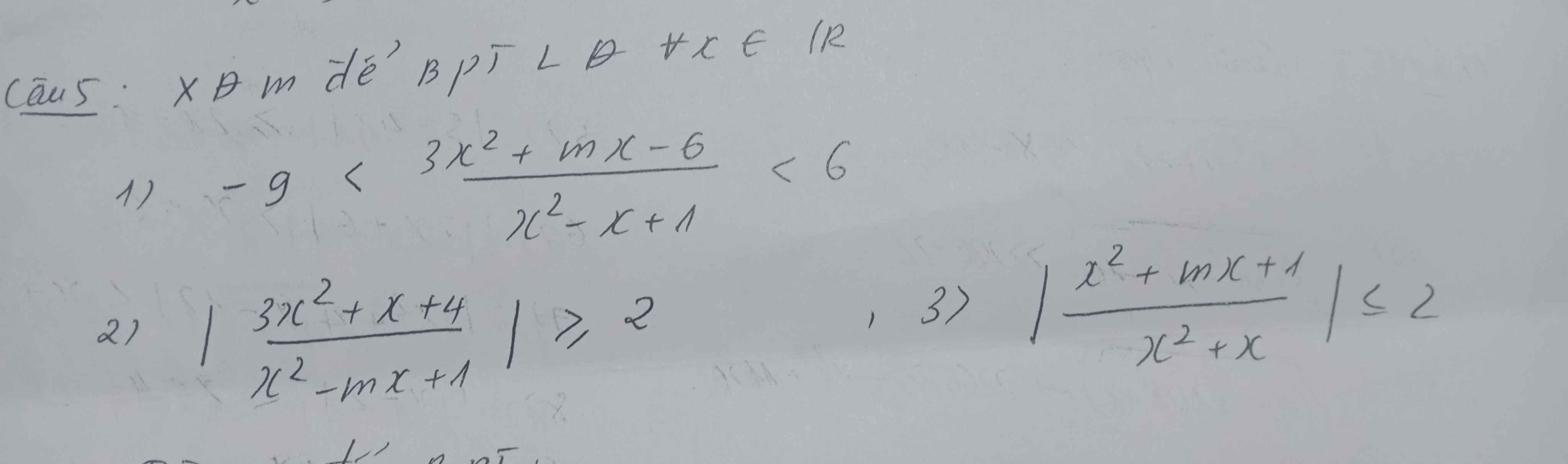

 làm giúp e câu này với ạ
làm giúp e câu này với ạ
Tiền lời khi bán 1 đôi giày: \(x-30\) (đô la)
Số tiền lời mà cửa hàng thu được:
\(\left(x-30\right)\left(80-x\right)=-x^2+110x-2400=-\left(x-55\right)^2+625\le625\)
Dấu "=" xảy ra khi \(x-55=0\Leftrightarrow x=55\)
Vậy cửa hàng bán với giá 55 đô la 1 đôi giày sẽ thu được lời lớn nhất